Max Diem - Quantum Mechanical Foundations of Molecular Spectroscopy

If the wavefunctions Ψ( x, t ) are normalized, Eq. (2.7)simplifies to

(2.9)

since the denominator in Eq. (2.8)equals 1. This expectation value may be viewed as an expected average of many independent measurements and embodies the probabilistic nature of quantum mechanics.

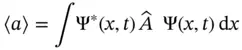
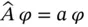Postulate 5: The eigenfunctions ϕ i , which are the solutions of the equation , form a complete orthogonal set of functions or, in other words, define a vector space. This, again, will be demonstrated in Section 2.3for the particle‐in‐a‐box wavefunctions, which are all orthogonal to each other and therefore may be considered unit vectors in a vector space.

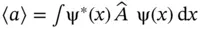When evaluating the expectation values ( Eq. [2.9]), the functions ψ( x ) may or may not be eigenfunctions of 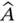because the real eigenfunctions ϕ ( x ) form a complete vector space. Functions that are not eigenfunctions of 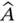can be written as linear combinations of the basis functions ϕ ( x ). Thus, any arbitrary wavefunction ψ of a system can be written in terms of a series expansion of the true eigenfunctions ϕ ( x ) as follows: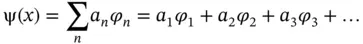

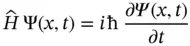
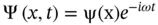
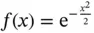(2.10)

The expansion coefficients a nindicate how much each wavefunction contributes to, or resembles, the true eigenfunction of the operator. This aspect is particularly important for the approximate methods for solving the Schrödinger equation discussed in Appendix 2.

Postulate 6: Time‐dependent systems are described by the time‐dependent Schrödinger equation

(2.11)

where the time‐dependent wavefunctions are the product of a time‐independent part, ψ(x), and a time evolution part:

(2.12)

We shall encounter the time‐dependent Schrödinger equation mainly in processes where molecular systems are subject to a perturbation by electromagnetic radiation (i.e. in spectroscopy) and shall develop the formalism that predicts whether or not the incident radiation will cause a transition in the molecule between two states with energy difference Δ E = h ν = ħ ω .

Next, a simple operator/eigenvalue example will be presented to illustrate some of the mathematical aspects.

Example 2.1Operator/eigenvalue problem

Show that the function is an eigenfunction of the operator 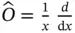, that is, show that

Answer:

(E2.1.1)

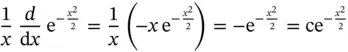
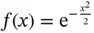The function is an eigenfunction of the operator. The eigenvalue c = −1.

Postulate 7: In many‐electron atoms, no two electrons can have identically the same set of quantum numbers. This postulate is known as the Pauli exclusion principle. It is also formulated as follows: the product wavefunction for all electrons in an atom must be antisymmetric with respect to interchange of two electrons. This postulate leads to the formulation of the product wavefunction in the form of Slater determinants (see Section 9.2) in many‐electron systems. The value of a determinant is zero when two rows or two columns are equal; thus, an atomic system where any electrons have exactly the same four quantum numbers would have an undefined product wavefunction. Furthermore, exchange of two rows (or columns) leads to a sign change of the value of the determinant. This last statement implies the antisymmetric property of the product wavefunction that changes its sign upon exchange of two electrons.

Commutation of operators: Although not really a postulate of quantum mechanics (since it follows from well‐defined mathematical principles), a discussion of the effects of operator commutation is included here. In physics, one often wishes to determine several quantities simultaneously, such as the position and momentum of a moving object or the x , y , and z components of the angular momentum. Since Postulate 3 above states that every observable is associated with a quantum mechanical operator, one has to investigate the case of solving for the eigenvalues of two operators simultaneously.

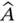Let and 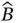be two operators such that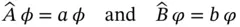

(2.13)

where a and b are the eigenvalues and φ and ϕ the eigenfunctions of and , respectively. These eigenvalues can be determined simultaneously in the same vector space if and only if the operators commutate, that is, if the order of application of the operators on the eigenfunction is immaterial. This commutator of two operators is written as

(2.14)