M. Kemal Ozgoren - Kinematics of General Spatial Mechanical Systems

(3.190)

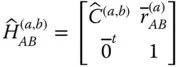
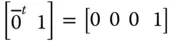Note that the HTM defined above has three major partitions. Its invariant trivial partition is its last row, which is . Its rotational partition is the 3 × 3 matrix and its translational partition is the 3 × 1 matrix .

By using the preceding definitions, Eq. (3.187)can be written in the following compact and linear form, which is known as the homogeneous transformation equation .

(3.191)

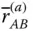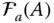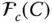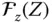If there are several different reference frames such as , , , …, , then the following successive homogeneous transformation equations can be written.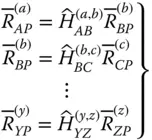

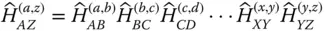
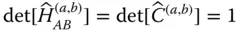
(3.192)

As for the overall homogeneous transformation equation, it can be written as

(3.193)

Upon successive substitutions, the preceding equations lead to the following equation for the combined HTM.

(3.194)

As noticed above, the expression of the overall HTM given by Eq. (3.194)involves only matrix multiplications and thus it is much more compact and easier to compute as compared with the accumulation of the consecutive expressions given by Eqs. (3.184)and (3.185)for the rotation matrix and the bias term of the overall affine transformation expressed by Eq. 3.180.

1 (a) Determinant of an HTM

Referring to Eq. (3.190), it can be shown that

(3.195)

1 (b) Inverse of an HTM

Equation (3.191)can be written in the following two ways: first by interchanging and ; and then by inverting .

(3.196)

(3.197)

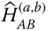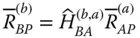
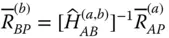
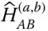Equations (3.196)and (3.197)imply that the inverse of can be taken as follows: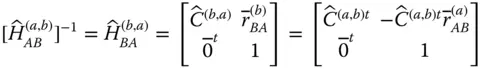

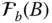(3.198)

1 (c) Decomposition of an HTM

The overall displacement of with respect to 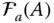consists of translational and rotational displacements. So, it can be described in the following two alternative ways.

(3.199)

(3.200)

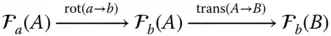
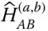According to the above descriptions, can be factorized as shown below.

1 (i) First translation and then rotation:(3.201)

2 (ii) First rotation and then translation:(3.202)

The factorizations described above suggest the following definitions of pure rotational and translational displacements and the associated homogeneous transformation matrices.

1 (d) HTM of a Pure Rotation

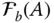A pure rotational displacement of with respect to 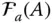or a pure rotational displacement of 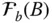with respect to can be achieved by pivoting about either of the origins A and B . In either case, the resultant HTM will be the same. That is,

(3.203)

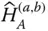In Eq. (3.203), and 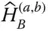are the abbreviated symbols that stand for and 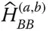.