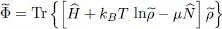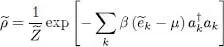(23) 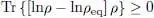
Relation (22)thus implies that for any density operator ρ having a trace equal to 1, we have:
(24) 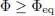
the equality occurring if, and only if, ρ = ρ eq.
Relation (24)can be used to fix a variational principle: choosing a family of density operators ρ having a trace equal to 1, we try to identify in this family the operator that yields the lowest value for Φ. This operator will then be the optimal operator within this family. Furthermore, this operator yields an upper value for the grand potential, with an error of second order with respect to the error made on ρ .
2. Approximation for the equilibrium density operator
We now use this variational principle with a family of density operators that leads to manageable calculations.
2-a. Trial density operators
The Hartree-Fock method is based on the assumption that a good approximation is to consider that each particle is independent of the others, but moving in the mean potential they create. We therefore compute an approximate value of the density operator by replacing the Hamiltonian Ĥ by a sum of independent particles’ Hamiltonians  :
:
(25) 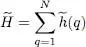
We now introduce the basis of the creation and annihilation operators, associated with the eigenvectors of the one-particle operator 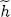 :
:
(26) 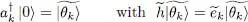
The symmetric one-particle operator 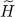 can then be written, according to relation (B-14) of Chapter XV:
can then be written, according to relation (B-14) of Chapter XV:
(27) 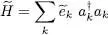
where the real constants 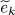 are the eigenvalues of the operator
are the eigenvalues of the operator 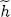 .
.
We choose as trial operators acting in the Fock space the set of operators  that can be written in the form corresponding to an equilibrium in the grand canonical ensemble – see relation (42)of Appendix VI. We then set:
that can be written in the form corresponding to an equilibrium in the grand canonical ensemble – see relation (42)of Appendix VI. We then set:
(28) 
where 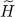 is any symmetric one-particle operator, the constant β the inverse of the temperature defined in (4), μ a real constant playing the role of a chemical potential, and
is any symmetric one-particle operator, the constant β the inverse of the temperature defined in (4), μ a real constant playing the role of a chemical potential, and  the trace of
the trace of  :
:
(29) 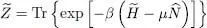
Consequently, the relevant variables in our problem are the states 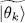 , which form an arbitrary orthonormal basis in the individual state space, and the energies
, which form an arbitrary orthonormal basis in the individual state space, and the energies 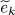 . These variables determine the ak as well as
. These variables determine the ak as well as 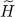 , and we have to find which of their values minimizes the function:
, and we have to find which of their values minimizes the function:
(30) 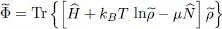
Taking (27)and (28)into account, we can write:
(31) 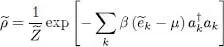
The following computations are simplified since the Fock space can be considered to be the tensor product of independent spaces associated with the individual states 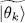 ; consequently, the trial density operator (28)can be written as a tensor product of operators each acting on a single mode k :
; consequently, the trial density operator (28)can be written as a tensor product of operators each acting on a single mode k :
(32) 
2-b. Partition function, distributions
Equality (32)has the same form as relation (5)of Complement B XV, with a simple change: the replacement of the free particle energies ek = ħ 2 k 2/2 m by the energies 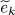 , which are as yet unknown. As this change does not impact the mathematical structure of the density operator, we can directly use the results of Complement B XV.
, which are as yet unknown. As this change does not impact the mathematical structure of the density operator, we can directly use the results of Complement B XV.
α. Variational partition function
The function  only depends on the variational energies
only depends on the variational energies 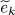 , since the trace of (32)may be computed in the basis {
, since the trace of (32)may be computed in the basis { 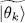 }, which yields:
}, which yields:
Читать дальше

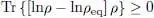
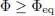
 :
: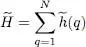
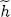 :
: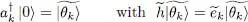
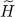 can then be written, according to relation (B-14) of Chapter XV:
can then be written, according to relation (B-14) of Chapter XV: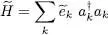
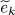 are the eigenvalues of the operator
are the eigenvalues of the operator  that can be written in the form corresponding to an equilibrium in the grand canonical ensemble – see relation (42)of Appendix VI. We then set:
that can be written in the form corresponding to an equilibrium in the grand canonical ensemble – see relation (42)of Appendix VI. We then set:
 the trace of
the trace of 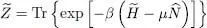
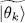 , which form an arbitrary orthonormal basis in the individual state space, and the energies
, which form an arbitrary orthonormal basis in the individual state space, and the energies