Mohammad Asadzadeh - An Introduction to the Finite Element Method for Differential Equations

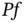projection in 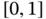.Figure 3.15 Midpoint approximation of the integral 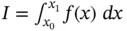.Figure 3.16 Trapezoidal approximation 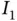of the integral 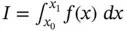.Figure 3.17 Simpson's rule approximation of the integral 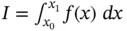.Figure 3.18 Identification of subintervals for composite Simpson's rule.Figure 3.19 Coefficients for composite Simpson's rule.

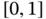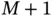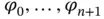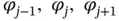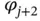4 Chapter 5Figure 5.1 A partition of into nonuniform subintervals.Figure 5.2 The basis functions .Figure 5.3 , and .Figure 5.4 The basis test and trial functions for this problem.

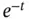5 Chapter 6Figure 6.1 Example of finite difference schemes for population model. E.E., ...Figure 6.2 Two continuous linear Galerkin approximations of .Figure 6.3 The jump and the right and left limits 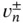.Figure 6.4 Time directions in forward and dual solutions.Figure 6.5 Orthogonality: .

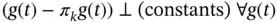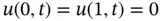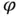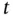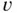6 Chapter 7Figure 7.1 A decreasing temperature profile with data .Figure 7.2 and its linear interpolant (both in and ) .Figure 7.3 Quadratic Lagrange basis functions 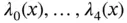.Figure 7.4 The linear time (a) and space (b) basis functions.Figure 7.5 Flow of cars with on highway.Figure 7.6 Characteristic line.Figure 7.7 A 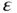boundary layer.Figure 7.8 Upstreams transported oscillatory approximate solution.Figure 7.9 A 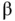‐telted mesh in a slab .

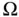7 Chapter 8Figure 8.1 A smooth domain with an outward unit normal 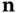.Figure 8.2 A rectangular domain with outward unit normals to its sides.Figure 8.3 A linear function on a subinterval 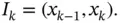Figure 8.4 Example of triangulation of a domain.Figure 8.5 A triangle in 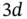as a piecewise linear function and its projection...Figure 8.6 A linear basis function in a triangulation in 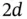.Figure 8.7 Uniform triangulation of 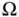with 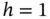.Figure 8.8 Support of a single basis function in Example 8.1.Figure 8.9 (a) Uniform triangulation of 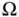and (b) the reference element 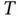.Figure 8.10 The nodal interpolant of 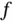in case.

Читать дальше