(2.113) 
whose substitution into the triple integrals in Eqs. ( 2.110) and ( 2.111) leads to the integration in the longitude, 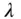 , being carried out independently of
, being carried out independently of 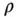 and
and 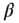 as follows:
as follows:
(2.114) 
This implies that 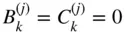 and
and
(2.115) 
These simplifications allow the gravitational potential of an axisymmetric body to be expressed as follows:
(2.116) 
where
(2.117) 
A more useful expression for the gravitational potential can be obtained as follows in terms of the non‐dimensional distance,  , where
, where  is the equatorial radius of the axisymmetric body:
is the equatorial radius of the axisymmetric body:
(2.118) 
where 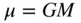 and
and
(2.119) 
are called Jeffery's constants , and are unique for a body of a given mass distribution. Jeffery's constants represent the spherical harmonics of the mass distribution, and diminish in magnitude as the order, k , increases. The largest of these constants, 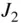 , denotes a non‐dimensional difference between the moments of inertia about the polar axis,
, denotes a non‐dimensional difference between the moments of inertia about the polar axis,  , and an axis in the equatorial plane (
, and an axis in the equatorial plane (  or
or  in Fig. 2.5), and is a measure of the ellipticity (or oblateness ) of the body. The higher order term,
in Fig. 2.5), and is a measure of the ellipticity (or oblateness ) of the body. The higher order term, 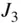 indicates the pear‐shaped or triangular harmonic, whereas
indicates the pear‐shaped or triangular harmonic, whereas  and
and 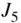 are the measures of square and pentagonal shaped harmonics, respectively. For a reasonably large body, it is seldom necessary to include more than the first four Jeffery's constants. For example, Earth's spherical harmonics are given by
are the measures of square and pentagonal shaped harmonics, respectively. For a reasonably large body, it is seldom necessary to include more than the first four Jeffery's constants. For example, Earth's spherical harmonics are given by 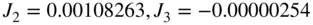 , and
, and 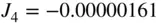 .
.
The acceleration due to gravity of an axisymmetric body is obtained by taking the gradient of the gravitational potential, Eq. (2.118), with respect to the position vector, 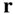 , and can be resolved in the radial,
, and can be resolved in the radial, 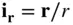 , and the north polar,
, and the north polar, 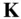 , directions (Fig. 2.5) as follows:
, directions (Fig. 2.5) as follows:
(2.120) 
where the following identities have been employed:
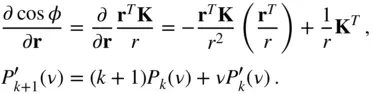
The acceleration can be alternatively resolved in two mutually perpendicular directions, 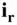 and
and 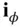 (see Fig. 2.5). The unit vectors
(see Fig. 2.5). The unit vectors 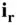 and
and 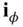 denote the radial and southward directions, as shown in Fig. 2.5, while the unit vector
denote the radial and southward directions, as shown in Fig. 2.5, while the unit vector 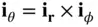 signifies the direction of the increasing longitude; that is, the eastward direction. These unit vectors constitute a moving coordinate frame,
signifies the direction of the increasing longitude; that is, the eastward direction. These unit vectors constitute a moving coordinate frame, 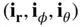 , attached to the test mass as shown in Fig. 2.5. Such a frame is called a local‐horizon frame (see Chapter 5). The acceleration given by Eq. (2.120)is resolved in
, attached to the test mass as shown in Fig. 2.5. Such a frame is called a local‐horizon frame (see Chapter 5). The acceleration given by Eq. (2.120)is resolved in 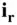 and
and 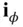 by substituting
by substituting 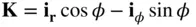 , resulting in
, resulting in
Читать дальше


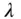 , being carried out independently of
, being carried out independently of 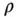 and
and 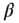 as follows:
as follows:
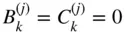 and
and


 , where
, where  is the equatorial radius of the axisymmetric body:
is the equatorial radius of the axisymmetric body:
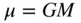 and
and
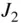 , denotes a non‐dimensional difference between the moments of inertia about the polar axis,
, denotes a non‐dimensional difference between the moments of inertia about the polar axis,  , and an axis in the equatorial plane (
, and an axis in the equatorial plane ( 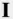 or
or 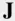 in Fig. 2.5), and is a measure of the ellipticity (or oblateness ) of the body. The higher order term,
in Fig. 2.5), and is a measure of the ellipticity (or oblateness ) of the body. The higher order term, 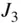 indicates the pear‐shaped or triangular harmonic, whereas
indicates the pear‐shaped or triangular harmonic, whereas  and
and 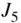 are the measures of square and pentagonal shaped harmonics, respectively. For a reasonably large body, it is seldom necessary to include more than the first four Jeffery's constants. For example, Earth's spherical harmonics are given by
are the measures of square and pentagonal shaped harmonics, respectively. For a reasonably large body, it is seldom necessary to include more than the first four Jeffery's constants. For example, Earth's spherical harmonics are given by 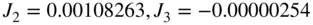 , and
, and 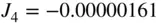 .
.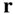 , and can be resolved in the radial,
, and can be resolved in the radial, 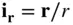 , and the north polar,
, and the north polar, 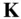 , directions (Fig. 2.5) as follows:
, directions (Fig. 2.5) as follows:
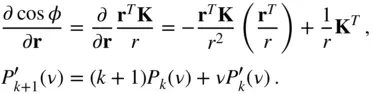
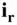 and
and 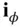 (see Fig. 2.5). The unit vectors
(see Fig. 2.5). The unit vectors 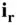 and
and 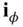 denote the radial and southward directions, as shown in Fig. 2.5, while the unit vector
denote the radial and southward directions, as shown in Fig. 2.5, while the unit vector 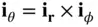 signifies the direction of the increasing longitude; that is, the eastward direction. These unit vectors constitute a moving coordinate frame,
signifies the direction of the increasing longitude; that is, the eastward direction. These unit vectors constitute a moving coordinate frame, 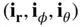 , attached to the test mass as shown in Fig. 2.5. Such a frame is called a local‐horizon frame (see Chapter 5). The acceleration given by Eq. (2.120)is resolved in
, attached to the test mass as shown in Fig. 2.5. Such a frame is called a local‐horizon frame (see Chapter 5). The acceleration given by Eq. (2.120)is resolved in 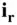 and
and 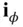 by substituting
by substituting 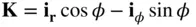 , resulting in
, resulting in










