Robert Bartoszynski - Probability and Statistical Inference
Здесь есть возможность читать онлайн «Robert Bartoszynski - Probability and Statistical Inference» — ознакомительный отрывок электронной книги совершенно бесплатно, а после прочтения отрывка купить полную версию. В некоторых случаях можно слушать аудио, скачать через торрент в формате fb2 и присутствует краткое содержание. Жанр: unrecognised, на английском языке. Описание произведения, (предисловие) а так же отзывы посетителей доступны на портале библиотеки ЛибКат.
- Название:Probability and Statistical Inference
- Автор:
- Жанр:
- Год:неизвестен
- ISBN:нет данных
- Рейтинг книги:4 / 5. Голосов: 1
-
Избранное:Добавить в избранное
- Отзывы:
-
Ваша оценка:
Probability and Statistical Inference: краткое содержание, описание и аннотация
Предлагаем к чтению аннотацию, описание, краткое содержание или предисловие (зависит от того, что написал сам автор книги «Probability and Statistical Inference»). Если вы не нашли необходимую информацию о книге — напишите в комментариях, мы постараемся отыскать её.
Probability and Statistical Inference, Third Edition
Probability and Statistical Inference

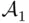 and
and  contain the class
contain the class 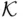 , then the intersection
, then the intersection  also contains
also contains  . The property extends to any intersection of fields containing
. The property extends to any intersection of fields containing 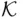 (not only the intersections of two such fields).
(not only the intersections of two such fields).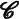 be the intersection of all fields containing
be the intersection of all fields containing  . We claim that
. We claim that  is the minimal unique field containing
is the minimal unique field containing  . We have to show that (1)
. We have to show that (1)  exists, (2)
exists, (2)  is a field containing
is a field containing 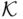 , (3)
, (3) 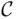 is unique, and (4)
is unique, and (4)  is minimal.
is minimal.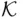 . We may take here the class of all subsets of
. We may take here the class of all subsets of 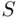 : it is a field (as well as a
: it is a field (as well as a 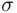 ‐field and monotone class), and it contains all sets in
‐field and monotone class), and it contains all sets in 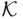 . Property (2) follows from the fact that the intersection of fields containing
. Property (2) follows from the fact that the intersection of fields containing  is a field containing
is a field containing  . Property (3) (i.e., uniqueness of
. Property (3) (i.e., uniqueness of 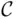 ) follows from the fact that the result of the operation of intersection is unique.
) follows from the fact that the result of the operation of intersection is unique.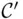 containing
containing 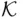 such that
such that 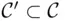 . Then
. Then 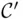 must appear as one of the factors in the intersection defining
must appear as one of the factors in the intersection defining 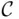 so that
so that  . Consequently,
. Consequently, 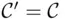 . This completes the proof for the case of fields. The proofs for
. This completes the proof for the case of fields. The proofs for  ‐fields and monotone classes are exactly the same, since an intersection of
‐fields and monotone classes are exactly the same, since an intersection of 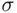 ‐fields (or monotone classes) containing
‐fields (or monotone classes) containing 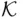 is again a
is again a 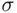 ‐field (monotone class) containing
‐field (monotone class) containing 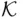 .
.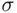 ‐fields of events. Beyond the trivial situations of finite or countably infinite sample spaces
‐fields of events. Beyond the trivial situations of finite or countably infinite sample spaces 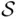 , where one can always consider the maximal
, where one can always consider the maximal 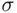 ‐field consisting of all subsets of
‐field consisting of all subsets of  , one is forced to restrict consideration to classes of events that form
, one is forced to restrict consideration to classes of events that form 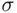 ‐fields generated by some “simple” events. The events in these
‐fields generated by some “simple” events. The events in these  ‐fields are typically of a very rich structure, and one seldom has useful criteria for distinguishing events (elements of the
‐fields are typically of a very rich structure, and one seldom has useful criteria for distinguishing events (elements of the 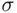 ‐field in question) from “nonevents,” that is, subsets of
‐field in question) from “nonevents,” that is, subsets of 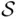 to which probabilities are not assigned. However, as shown by the two examples below, the smallest
to which probabilities are not assigned. However, as shown by the two examples below, the smallest  ‐field generated by some class is richer than the smallest field generated by the same class.
‐field generated by some class is richer than the smallest field generated by the same class.










