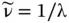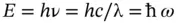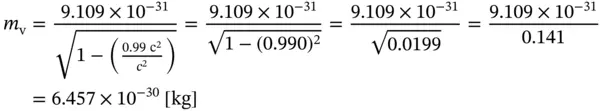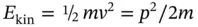The implication of the aforementioned wave–particle duality will be discussed in the next section.
1.3 The Photoelectric Effect
In 1905, Einstein reported experimental results that further demonstrated the energy quantization of light. In the photoelectric experiment, light of variable color (frequency) illuminated a photocathode contained in an evacuated tube. An anode in the same tube was connected externally to the cathode through a current meter and a source of electric potential (such as a battery). Since the cathode and anode were separated by vacuum, no current was observed, unless light with a frequency above a threshold frequency was illuminating the photocathode. Einstein correctly concluded that light particles, or photons, with a frequency above this threshold value had sufficient kinetic energy to knock out electrons from the metal atoms of the photocathode. These “photoelectrons” left the metal surface with a kinetic energy given by
(1.9) 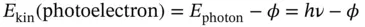
where ϕ is the work function, or the energy required to remove an electron from metal atoms. This energy basically is the atoms' ionization energy multiplied by Avogadro's number. Furthermore, Einstein reported that the photocurrent produced by the irradiation of the photocathode was proportional to the intensity of light, or the number of photons, but that increasing the intensity of light that had a frequency below the threshold did not produce any photocurrent. This provided further proof of Eq. (1.9).
This experiment further demonstrated that light has particle character with the kinetic energy of the photons given by Eq. (1.7), which led to the concept of wave–particle duality of light. Later, de Broglie theorized that the momentum p of a photon was given by
(1.10) 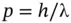
Equation (1.10)is known as the de Broglie equation. The wave–particle duality was later (1927) confirmed to be true for moving masses as well by the electron diffraction experiment of Davisson and Germer [3]. In this experiment, a beam of electrons was diffracted by an atomic lattice and produced a distinct interference pattern that suggested that the moving electrons exhibited wave properties. The particle–wave duality of both photons and moving matter can be summarized as follows.
For photons, the wave properties are manifested by diffraction experiments and summarized by Maxwell's equation. As for all wave propagation, the velocity of light, c , is related to wavelength λ and frequency ν by
(1.11) 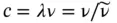
with c = 2.998 × 10 8[m/s] and λ expressed in [m] and ν expressed in [Hz = s −1]. The quantity  is referred to as the wavenumber of radiation (in units of m −1or cm −1) that indicates how many wave cycles occur per unit length:
is referred to as the wavenumber of radiation (in units of m −1or cm −1) that indicates how many wave cycles occur per unit length:
(1.12) 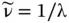
The (kinetic) energy of a photon is given by
(1.13) 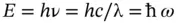
with ħ = h/ 2π and ω, the angular frequency, defined before as ω = 2π ν .
From the classical definition of the momentum of matter and light, respectively,
(1.14) 
it follows that the photon mass is given by
(1.15) 
Notice that a photon can only move at the velocity of light and the photon mass can only be defined at the velocity c . Therefore, a photon has zero rest mass, m 0.
Particles of matter, on the other hand, have a nonzero rest mass, commonly referred to as their mass. This mass, however, is a function of velocity v and should be referred to as m ν, which is given by
(1.16) 
Example 1.1Calculation of the mass of an electron moving at 99.0 % of the velocity of light (such velocities can easily be reached in a synchrotron).
Answer:
According to Eq. (1.16), the mass m vof an electron at ν = 0.99 c is
(E1.1.1) 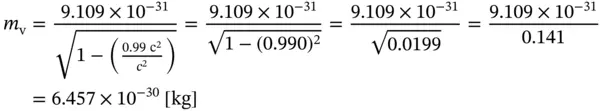
The electron at 99 % of the velocity of light has a mass of about seven times its rest mass.
Equation (1.16)demonstrates that the mass of any matter particle will reach infinity when accelerated to the velocity of light. Their kinetic energy at velocity ν (far from the velocity of light) is given by the classical expression
(1.17) 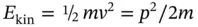
The discussion of the last paragraphs demonstrates that at the beginning of the twentieth century, experimental evidence was amassed that pointed to the necessity to redefine some aspects of classical physics. The next of these experiments that led to the formulation of quantum mechanics was the observation of “spectral lines” in the absorption and emission spectra of the hydrogen atom.
1.4 Hydrogen Atom Absorption and Emission Spectra
Between the last decades of the nineteenth century and the first decade of the twentieth century, several researchers discovered that hydrogen atoms, produced in gas discharge lamps, emit light at discrete colors, rather than as a broad continuum of light as observed for a blackbody ( Figure 1.2a). These emissions occur in the ultraviolet, visible, and near‐infrared spectral regions, and a portion of such an emission spectrum is shown schematically in Figure 1.3. These observations predate the efforts discussed in the previous two sections and therefore may be considered the most influential in the development of the connection between spectroscopy and quantum mechanics.
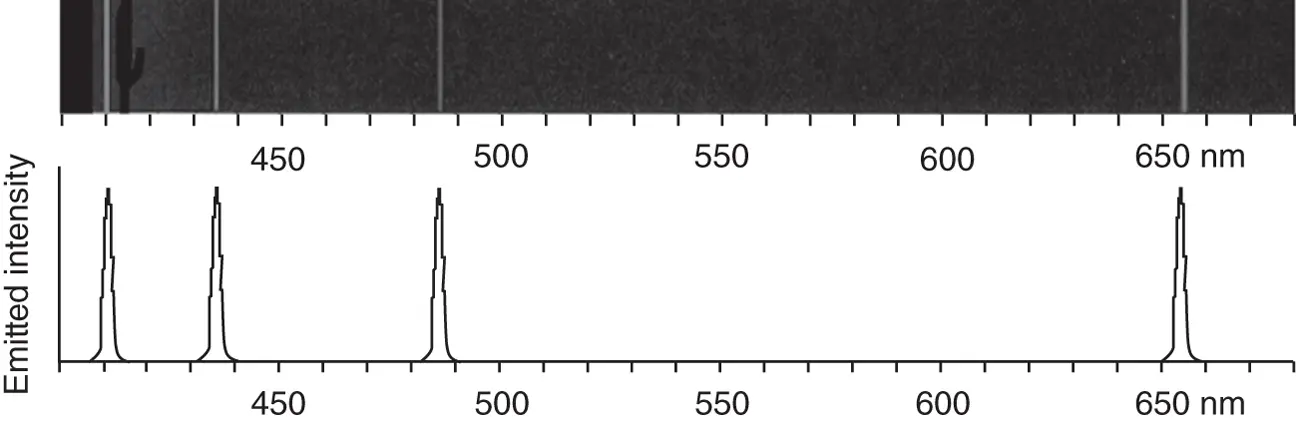
Figure 1.3 Portion of the hydrogen atom emission in the visible spectral range, represented as a “line spectrum” and schematically as an emission spectrum.
These experiments demonstrated that the H atom can exist in certain “energy states” or “stationary states.” These states can undergo a process that is referred to as a “transition.” When the atom undergoes such a transition from a higher or more excited state to a lower or less excited state, the energy difference between the states is emitted as a photon with an energy corresponding to the energy difference between the states:
Читать дальше

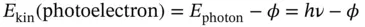
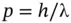
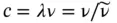
 is referred to as the wavenumber of radiation (in units of m −1or cm −1) that indicates how many wave cycles occur per unit length:
is referred to as the wavenumber of radiation (in units of m −1or cm −1) that indicates how many wave cycles occur per unit length: