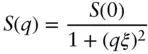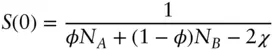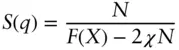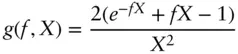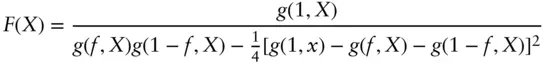Ian W. Hamley - Small-Angle Scattering

SAS data from blends is often fitted using the Ornstein‐Zernike function:

( 1.117)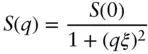

This expression can be derived from Eq. (1.116)in the limit of small q , [62, 65] with

(1.118)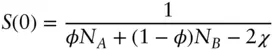

The correlation length in Eq. (1.117)can be shown [61] to be

(1.119)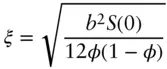

Analogous equations to Eq. (1.116)have been obtained for block copolymer melts, also using the random‐phase approximation. The result for a diblock copolymer (degree of polymerization N , Flory‐Huggins interaction parameter χ and volume fraction of one component f ) is [28, 66]

( 1.120)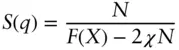

Here F ( X ) is a combination of Debye functions (cf. Eq. (1.107), X is defined after this equation) as follows:

(1.121)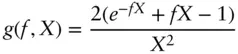

and

(1.122)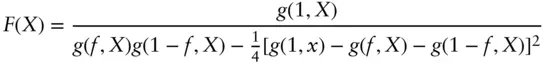

Figure 1.23shows an example of structure factors for a diblock copolymer in a disordered melt at several χN values, calculated using Eq. (1.120). Since χ is inversely proportional to temperature, the S ( q ) functions become less intense and broader as temperature increases, as expected.

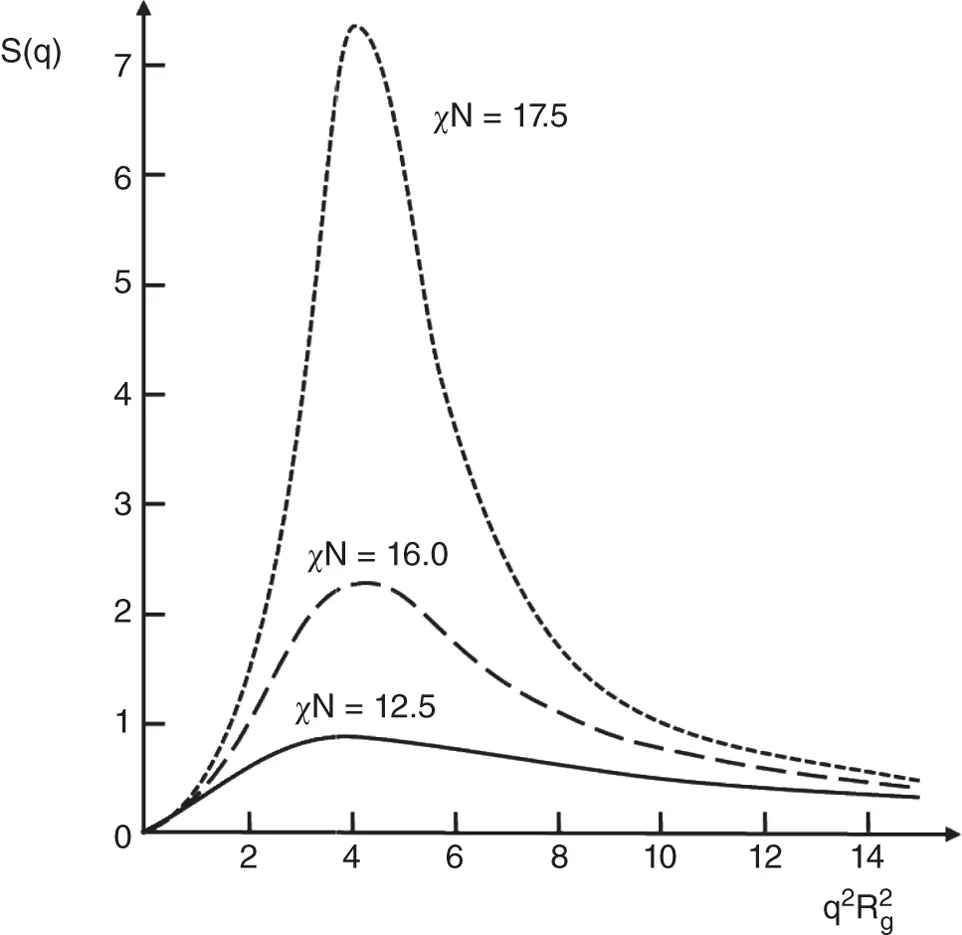
Figure 1.23 Structure factor for a diblock copolymer melt with f = 0.25 at three values of χN indicated [66]. The order‐disorder transition within this model occurs at χN = 17.6 at this composition.

Source: From Leibler [66]. © 1980, American Chemical Society.

Further information about scattering from block copolymers can be found elsewhere [28, 67, 68].

1 1. van de Hulst, H.C. (1957). Light Scattering by Small Particles. New York: Dover.

2 2. Brown, W. (ed.) (1996). Light Scattering ‐ Principles and Development. Oxford: Oxford University Press.

3 3. Lindner, P. and Zemb, T. (eds.) (2002). Neutrons, X‐Rays and Light. Scattering Methods Applied to Soft Condensed Matter. Amsterdam: Elsevier.

4 4. Glatter, O. (2018). Scattering Methods and their Application in Colloid and Interface Science. Amsterdam: Elsevier.

5 5. Guinier, A. and Fournet, G. (1955). Small Angle Scattering of X‐Rays. New York: Wiley.

6 6. Glatter, O. and Kratky, O. (eds.) (1982). Small Angle X‐Ray Scattering. London: Academic.

7 7. Feigin, L.A. and Svergun, D.I. (1987). Structure Analysis by Small‐Angle X‐Ray and Neutron Scattering. New York: Plenum.

8 8. Hamley, I.W. (1991). Scattering from uniform, cylindrically symmetric particles in liquid crystal phases. The Journal of Chemical Physics 95: 9376–9383.

9 9. Svergun, D.I. and Koch, M.H.J. (2003). Small‐angle scattering studies of biological macromolecules in solution. Reports on Progress in Physics 66: 1735–1782.

10 10. Glatter, O. (2002). The inverse scattering problem in small‐angle scattering. In: Neutrons, X‐Rays and Light Scattering Methods Applied to Soft Condensed Matter (eds. P. Lindner and T. Zemb), 73–102. Amsterdam: Elsevier.

11 11. Svergun, D.I., Koch, M. H. J, Timmins, P.A., and May, R.P. (2013). Small Angle X‐ray and Neutron Scattering from Solutions of Biological Macromolecules. Oxford: Oxford University Press.

12 12. Roe, R.‐J. (2000). Methods of X‐Ray and Neutron Scattering in Polymer Science. New York: Oxford University Press.

13 13. Glatter, O. (2018). Scattering Methods and their Application in Colloid and Interface Science, Chap.3. Amsterdam: Elsevier.

14 14. Brennich, M., Pernot, P., and Round, A. (2017). How to Analyze and Present SAS Data for Publication. In: Biological Small Angle Scattering: Techniques, Strategies and Tips, Advances in Experimental Medicine and Biology (ed. B. Chaudhuri), 47–64. Berlin: Springer.

15 15. Castelletto, V., Edwards‐Gayle, C.J.C., Hamley, I.W. et al. (2019). Self‐assembly of a catalytically active lipopeptide and its incorporation into cubosomes. ACS Applied Bio Materials 2: 3639–3648.

16 16. Kotlarchyk, M. and Chen, S.‐H. (1983). Analysis of small‐angle scattering spectra from polydisperse interacting colloids. The Journal of Chemical Physics 79: 2461–2469.

17 17. Pedersen, J.S. (2002). Modelling of small‐angle scattering data. In: Neutrons, X‐Rays and Light Scattering Methods Applied to Soft Condensed Matter (eds. P. Lindner and T. Zemb). Amsterdam: Elsevier.

18 18. Pedersen, J.S. (1997). Analysis of small‐angle scattering data from colloids and polymer solutions: modeling and least‐squares fitting. Advances in Colloid and Interface Science 70: 171–210.

19 19. Foffi, G., Savin, G., Bucciarelli, S. et al. (2014). Hard sphere‐like glass transition in eye lens alpha‐crystalline solutions. Proceedings of National Academy of Sciences of the United States of America 111: 16748–16753.

20 20. Hamley, I.W. (2007). Introduction to Soft Matter. Revised Edition. Chichester: Wiley.

21 21. Bressler, I., Kohlbrecher, J., and Thünemann, A.F. (2015). SASfit: a tool for small‐angle scattering data analysis using a library of analytical expressions. Journal of Applied Crystallography 48: 1587–1598.

22 22. Hosemann, R. and Bagchi, S.N. (1962). Direct Analysis of Diffraction by Matter. Amsterdam: North‐Holland.

23 23. Caillé, A. X-ray scattering by smectic-A crystals. Comptes Rendus Hebdomaires des Seances de l'Academie des Sciences, Series B 274: 891–892.

24 24. Zhang, R.T., Suter, R.M., and Nagle, J.F. (1994). Theory of the structure factor of lipid bilayers. Physical Review E 50: 5047–5060.

25 25. Pabst, G., Rappolt, M., Amenitsch, H., and Laggner, P. (2000). Structural information from multilamellar liposomes at full hydration: full q‐range fitting with high quality x‐ray data. Physical Review E 62: 4000–4009.

26 26. Giacovazzo, C., Monaco, H.L., Viterbo, D. et al. (1992). Fundamentals of Crystallography. Oxford: International Union of Crystallography/Oxford University Press.

27 27. Förster, S., Timmann, A., Konrad, M. et al. (2005). Scattering functions of ordered mesoscopic materials. Journal of Physical Chemistry B 109: 1347–1360.

28 28. Hamley, I.W. and Castelletto, V. (2004). Small‐angle scattering of block copolymers in the melt, solution and crystal states. Progress in Polymer Science 29: 909–948.

29 29. Cullity, B.D. and Stock, S.R. (2001). Elements of X‐Ray Diffraction. Prentice Hall: Upper Saddle River, New Jersey.

30 30. de Jeu, W.H. (2016). Basic X‐Ray Scattering for Soft Matter. Oxford: Oxford University Press.