Simon Haykin - Nonlinear Filters
Здесь есть возможность читать онлайн «Simon Haykin - Nonlinear Filters» — ознакомительный отрывок электронной книги совершенно бесплатно, а после прочтения отрывка купить полную версию. В некоторых случаях можно слушать аудио, скачать через торрент в формате fb2 и присутствует краткое содержание. Жанр: unrecognised, на английском языке. Описание произведения, (предисловие) а так же отзывы посетителей доступны на портале библиотеки ЛибКат.
- Название:Nonlinear Filters
- Автор:
- Жанр:
- Год:неизвестен
- ISBN:нет данных
- Рейтинг книги:5 / 5. Голосов: 1
-
Избранное:Добавить в избранное
- Отзывы:
-
Ваша оценка:
Nonlinear Filters: краткое содержание, описание и аннотация
Предлагаем к чтению аннотацию, описание, краткое содержание или предисловие (зависит от того, что написал сам автор книги «Nonlinear Filters»). Если вы не нашли необходимую информацию о книге — напишите в комментариях, мы постараемся отыскать её.
Discover the utility of using deep learning and (deep) reinforcement learning in deriving filtering algorithms with this insightful and powerful new resource Nonlinear Filters: Theory and Applications
Nonlinear Filters
Nonlinear Filters: Theory and Applications

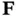 such that
such that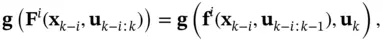
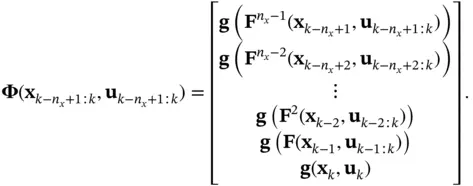
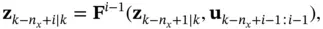
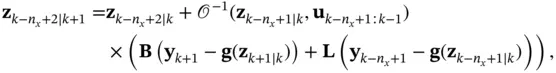
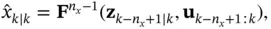
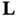 is the observer gain,
is the observer gain,  , and
, and 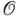 is the discrete nonlinear observability matrix, which is computed at sample time
is the discrete nonlinear observability matrix, which is computed at sample time  as:
as: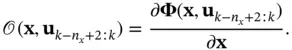
 :
: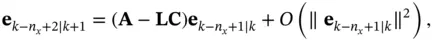
 and
and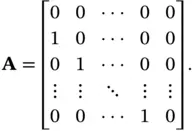
 , with
, with 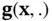 and
and 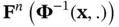 being uniformly Lipschitz continuous functions of the state:
being uniformly Lipschitz continuous functions of the state:
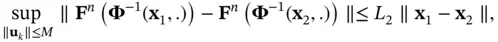
 and
and  denote the corresponding Lipschitz constants. However, convergence is guaranteed only for a neighborhood around the true state [36].
denote the corresponding Lipschitz constants. However, convergence is guaranteed only for a neighborhood around the true state [36].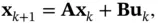
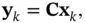
 ,
,  , and
, and  . It is assumed that the pair
. It is assumed that the pair 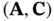 is observable, and
is observable, and 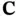 is full rank. The goal is to reconstruct the state vector from the input and the output vectors using the discrete‐time sliding‐mode framework. Using a nonsingular transformation matrix,
is full rank. The goal is to reconstruct the state vector from the input and the output vectors using the discrete‐time sliding‐mode framework. Using a nonsingular transformation matrix, 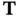 , the state vector is transformed into a partitioned form:
, the state vector is transformed into a partitioned form: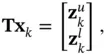
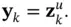
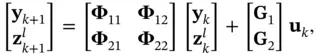
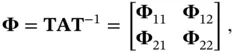
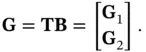
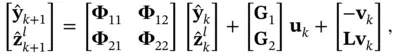
 , and
, and 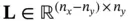 is the observer gain. Defining the estimation errors as:
is the observer gain. Defining the estimation errors as:










