Daniel J. Duffy - Numerical Methods in Computational Finance
Здесь есть возможность читать онлайн «Daniel J. Duffy - Numerical Methods in Computational Finance» — ознакомительный отрывок электронной книги совершенно бесплатно, а после прочтения отрывка купить полную версию. В некоторых случаях можно слушать аудио, скачать через торрент в формате fb2 и присутствует краткое содержание. Жанр: unrecognised, на английском языке. Описание произведения, (предисловие) а так же отзывы посетителей доступны на портале библиотеки ЛибКат.
- Название:Numerical Methods in Computational Finance
- Автор:
- Жанр:
- Год:неизвестен
- ISBN:нет данных
- Рейтинг книги:4 / 5. Голосов: 1
-
Избранное:Добавить в избранное
- Отзывы:
-
Ваша оценка:
Numerical Methods in Computational Finance: краткое содержание, описание и аннотация
Предлагаем к чтению аннотацию, описание, краткое содержание или предисловие (зависит от того, что написал сам автор книги «Numerical Methods in Computational Finance»). Если вы не нашли необходимую информацию о книге — напишите в комментариях, мы постараемся отыскать её.
Part A Mathematical Foundation for One-Factor Problems
Chapters 1 to 7 introduce the mathematical and numerical analysis concepts that are needed to understand the finite difference method and its application to computational finance.
Part B Mathematical Foundation for Two-Factor Problems
Chapters 8 to 13 discuss a number of rigorous mathematical techniques relating to elliptic and parabolic partial differential equations in two space variables. In particular, we develop strategies to preprocess and modify a PDE before we approximate it by the finite difference method, thus avoiding ad-hoc and heuristic tricks.
Part C The Foundations of the Finite Difference Method (FDM)
Chapters 14 to 17 introduce the mathematical background to the finite difference method for initial boundary value problems for parabolic PDEs. It encapsulates all the background information to construct stable and accurate finite difference schemes.
Part D Advanced Finite Difference Schemes for Two-Factor Problems
Chapters 18 to 22 introduce a number of modern finite difference methods to approximate the solution of two factor partial differential equations. This is the only book we know of that discusses these methods in any detail.
Part E Test Cases in Computational Finance
Chapters 23 to 26 are concerned with applications based on previous chapters. We discuss finite difference schemes for a wide range of one-factor and two-factor problems.
This book is suitable as an entry-level introduction as well as a detailed treatment of modern methods as used by industry quants and MSc/MFE students in finance. The topics have applications to numerical analysis, science and engineering.
More on computational finance and the author’s online courses, see www.datasim.nl.


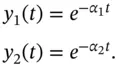
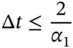
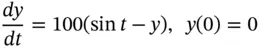
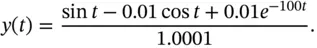
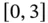 into 1.2 million subintervals in order to achieve accuracy to three decimal places. The implicit Euler and Crank–Nicolson methods are not much better.
into 1.2 million subintervals in order to achieve accuracy to three decimal places. The implicit Euler and Crank–Nicolson methods are not much better.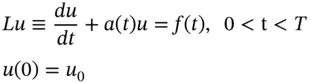


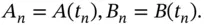 Then, using this formula and mathematical induction we can give an explicit solution at any time level as follows:
Then, using this formula and mathematical induction we can give an explicit solution at any time level as follows: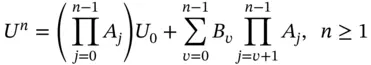

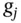 . A special case is when the coefficients
. A special case is when the coefficients 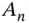 and
and 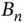 are constant
are constant 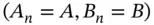 , that is:
, that is: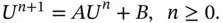
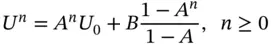
 power of constant
power of constant 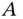 and
and 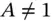 .
.