Robert Bartoszynski - Probability and Statistical Inference
Здесь есть возможность читать онлайн «Robert Bartoszynski - Probability and Statistical Inference» — ознакомительный отрывок электронной книги совершенно бесплатно, а после прочтения отрывка купить полную версию. В некоторых случаях можно слушать аудио, скачать через торрент в формате fb2 и присутствует краткое содержание. Жанр: unrecognised, на английском языке. Описание произведения, (предисловие) а так же отзывы посетителей доступны на портале библиотеки ЛибКат.
- Название:Probability and Statistical Inference
- Автор:
- Жанр:
- Год:неизвестен
- ISBN:нет данных
- Рейтинг книги:4 / 5. Голосов: 1
-
Избранное:Добавить в избранное
- Отзывы:
-
Ваша оценка:
Probability and Statistical Inference: краткое содержание, описание и аннотация
Предлагаем к чтению аннотацию, описание, краткое содержание или предисловие (зависит от того, что написал сам автор книги «Probability and Statistical Inference»). Если вы не нашли необходимую информацию о книге — напишите в комментариях, мы постараемся отыскать её.
Probability and Statistical Inference, Third Edition
Probability and Statistical Inference

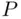 satisfies Axiom 3 of countable additivity, then
satisfies Axiom 3 of countable additivity, then 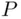 is continuous from above and from below. Conversely, if a function
is continuous from above and from below. Conversely, if a function 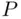 satisfies Axioms 1 and 2, is finitely additive, and is either continuous from below or continuous from above at the empty set
satisfies Axioms 1 and 2, is finitely additive, and is either continuous from below or continuous from above at the empty set  , then
, then  is countably additive.
is countably additive.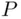 satisfies Axiom 3, and let
satisfies Axiom 3, and let 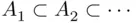 be a monotone increasing sequence. We have
be a monotone increasing sequence. We have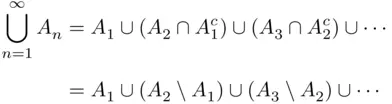
 (see Section 1.5), using ( 2.8), and the assumption of countable additivity, we obtain
(see Section 1.5), using ( 2.8), and the assumption of countable additivity, we obtain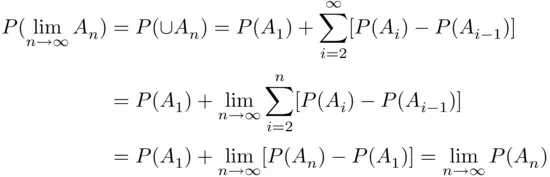
 from below. To prove continuity from above, we pass to the complements, and proceed as above.
from below. To prove continuity from above, we pass to the complements, and proceed as above. is finitely additive and continuous from below, and let
is finitely additive and continuous from below, and let 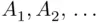 be a sequence of mutually disjoint events. Put
be a sequence of mutually disjoint events. Put  so that
so that 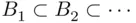 is a monotone increasing sequence with
is a monotone increasing sequence with 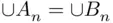 . We have then, using continuity from below and finite additivity,
. We have then, using continuity from below and finite additivity,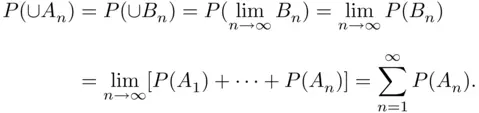
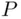 is countably additive.
is countably additive.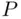 is finitely additive and continuous from above at the empty set
is finitely additive and continuous from above at the empty set 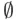 (impossible event). Taking again a sequence of disjoint events
(impossible event). Taking again a sequence of disjoint events 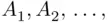 let
let  . We have
. We have 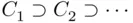 and
and  . By finite additivity, we obtain
. By finite additivity, we obtain
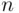 , we can write
, we can write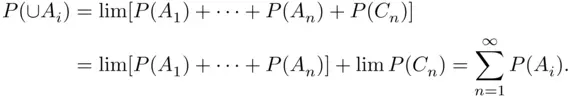
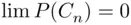 ,
, 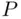 is countably additive, and the proof is complete.
is countably additive, and the proof is complete.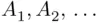 is a sequence of events such that
is a sequence of events such that
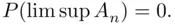
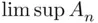 = “infinitely many events
= “infinitely many events  occur” =
occur” = 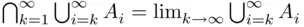 (because the unions
(because the unions 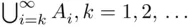 form a decreasing sequence). Consequently, using the continuity of
form a decreasing sequence). Consequently, using the continuity of 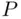 , subadditivity property (2.2), and assumption ( 2.10), we have
, subadditivity property (2.2), and assumption ( 2.10), we have










