3.14.3 Reverberation Time
In a reverberant space, the reverberation time T Ris normally defined to be the time for the sound pressure level to drop by 60 dB when the sound source is cut off (see Figure 3.20). Different reverberation times are desired for different types of spaces (see Figure 3.21). The Sabine formula is often used, T R= T 60(for 60 dB):
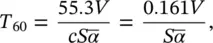
where V is room volume (m 3), c is the speed of sound (m/s), S is wall area (m 2), and 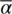 is the angle‐averaged wall absorption coefficient, or
is the angle‐averaged wall absorption coefficient, or
(3.74) 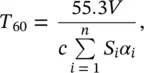
where S iis i th wall area of absorption coefficient α i.
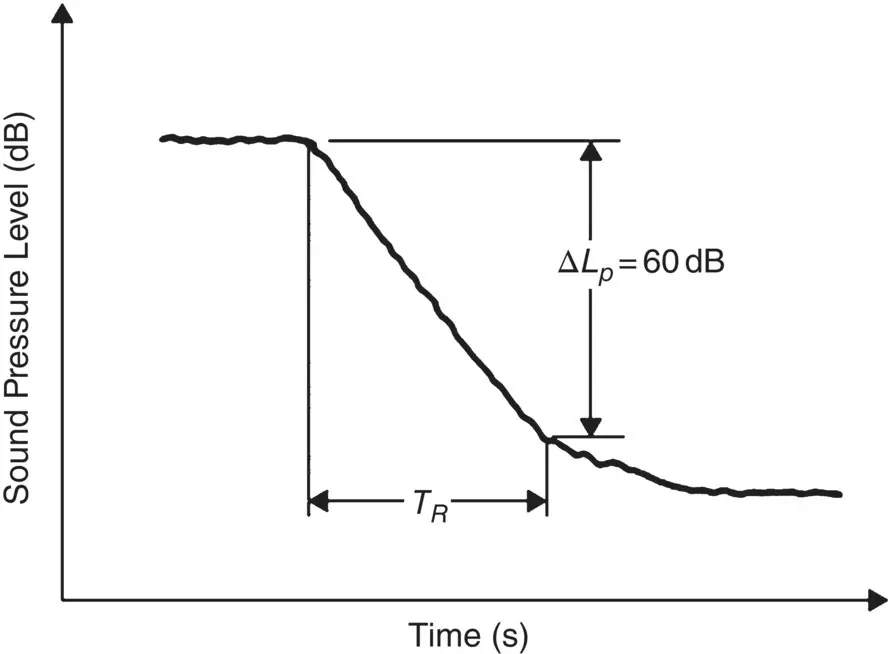
Figure 3.20 Measurement of reverberation time T R.
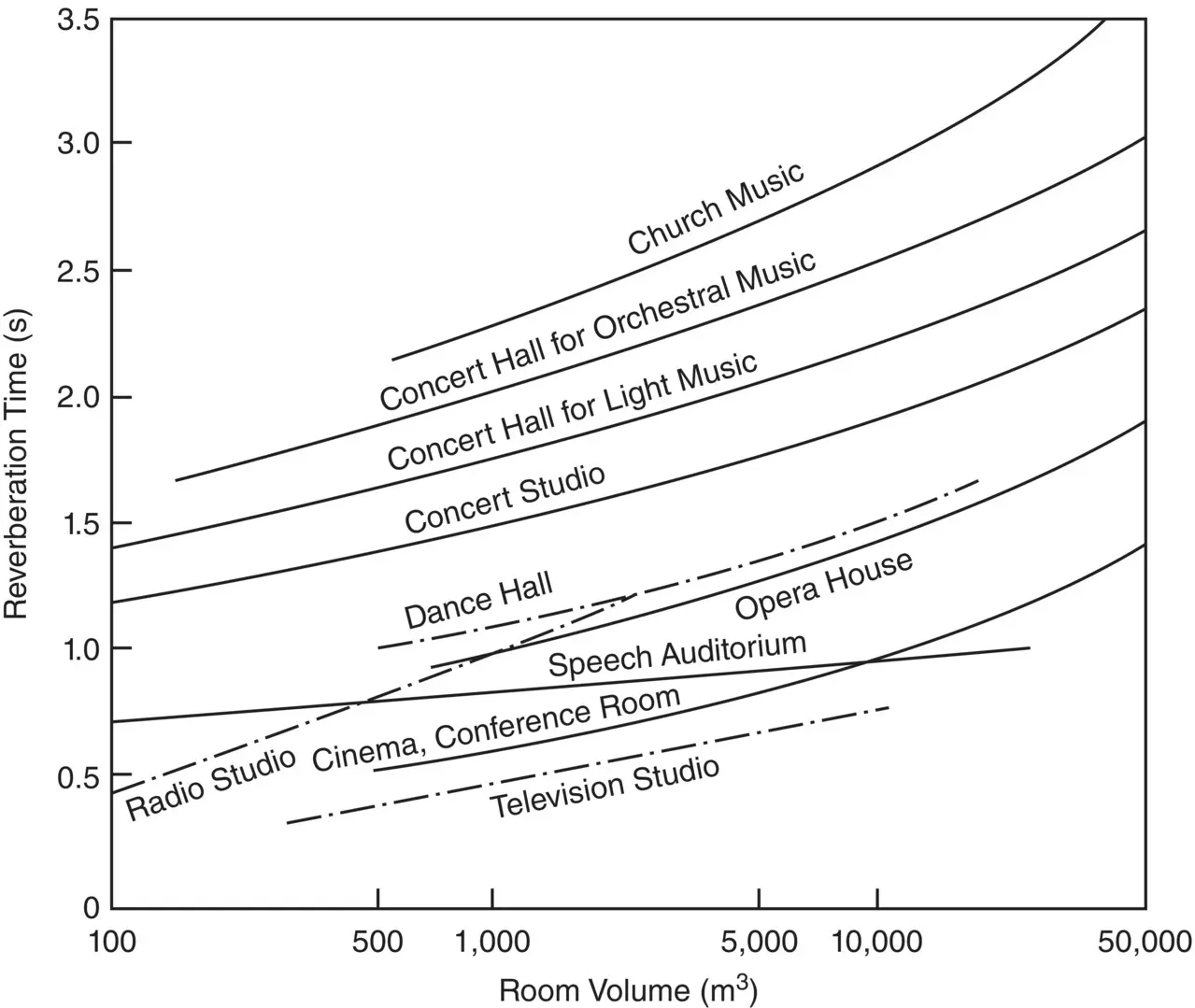
Figure 3.21 Examples of recommended reverberation times.
In practice, when the reverberation time is measured (see Figure 3.20), it is normal practice to ignore the first 5‐dB drop in sound pressure level and find the time between the 5‐dB and 35‐dB drops and multiply this time by 2 to obtain the reverberation time T R.
A room has dimensions 5 × 6 × 10 m 3. What is the reverberation time T 60if the floor (6 × 10 m) has absorbing material 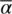 = 0.5 placed on it?
= 0.5 placed on it?
We will assume that 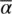 = 0 on the other surfaces (that are made of hard painted concrete.)
= 0 on the other surfaces (that are made of hard painted concrete.)

Notice that the Sabine reverberation time formula T 60= 0.16 V/S  still predicts a reverberation time as
still predicts a reverberation time as 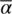 → 1, which does not agree with the physical world. This is approximately the case of an anechoic room (see Figure 3.22). Some improved formulas have been devised by Eyring and Millington‐Sette that overcome this problem. Sabine's formula is acceptable, provided
→ 1, which does not agree with the physical world. This is approximately the case of an anechoic room (see Figure 3.22). Some improved formulas have been devised by Eyring and Millington‐Sette that overcome this problem. Sabine's formula is acceptable, provided 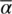 ≤ 0.5.
≤ 0.5.
A classroom has dimensions 4 × 6 × 10 m 3and a reverberation time of 1.5 seconds. (a) Determine the total sound absorption of the classroom; (b) if 35 students are in the classroom, and each is equivalent to 0.45 sabins (m 2) sound absorption, what is the new reverberation time of the classroom?
1 the volume of the classroom is V = 240 m3. Therefore
2 The total sound absorption is now 25.8 + 35(0.45) = 41.55 sabins (m2). Then
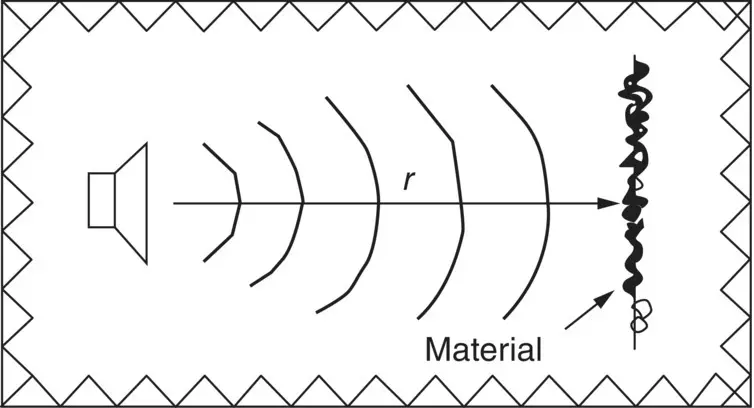
Figure 3.22 Sound source in anechoic room.
If we have a diffuse sound field (the same sound energy at any point in the room) and the field is also reverberant (the sound waves may come from any direction, with equal probability), then the sound intensity striking the wall of the room is found by integrating the plane wave intensity over all angles θ , 0 < θ < 90°. This involves a weighting of each wave by cos θ , and the average intensity for the wall in a reverberant field becomes
(3.75) 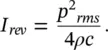
Note the factor 1/4 compared with the plane wave case.
For a point in a room at distance r from a source of power W watts, we will have a direct field intensity contribution W/ 4π r 2from an omnidirectional source to the mean square pressure and also a reverberant contribution.
We may define the reverberant field as the field created by waves after the first reflection of direct waves from the source. Thus the energy/second absorbed at the first reflection of waves from the source of sound power W is W 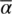 , where
, where  is the average absorption coefficient of the walls. The power thus supplied to the reverberant field is W (1 −
is the average absorption coefficient of the walls. The power thus supplied to the reverberant field is W (1 −  ) (after the first reflection). Since the power lost by the reverberant field must equal the power supplied to it for steady‐state conditions, then
) (after the first reflection). Since the power lost by the reverberant field must equal the power supplied to it for steady‐state conditions, then
(3.76) 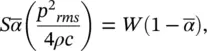
where p 2 rmsis the mean‐square sound pressure contribution caused by the reverberant field.
There is also the direct field contribution to be accounted for. If the source is a broadband noise source, these two contributions: (i) the direct term p 2 d,rms= ρcW /4 πr 2and (ii) the reverberant contribution,  . So,
. So,
(3.77) 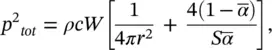
and after dividing by p 2 ref, and W refand taking 10 log, we obtain
(3.78) 
where R is the so‐called room constant 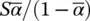 .
.
Читать дальше

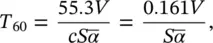
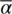 is the angle‐averaged wall absorption coefficient, or
is the angle‐averaged wall absorption coefficient, or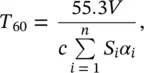


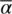 = 0.5 placed on it?
= 0.5 placed on it?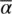 = 0 on the other surfaces (that are made of hard painted concrete.)
= 0 on the other surfaces (that are made of hard painted concrete.)
 still predicts a reverberation time as
still predicts a reverberation time as 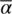 → 1, which does not agree with the physical world. This is approximately the case of an anechoic room (see Figure 3.22). Some improved formulas have been devised by Eyring and Millington‐Sette that overcome this problem. Sabine's formula is acceptable, provided
→ 1, which does not agree with the physical world. This is approximately the case of an anechoic room (see Figure 3.22). Some improved formulas have been devised by Eyring and Millington‐Sette that overcome this problem. Sabine's formula is acceptable, provided 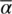 ≤ 0.5.
≤ 0.5.
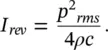
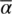 , where
, where  is the average absorption coefficient of the walls. The power thus supplied to the reverberant field is W (1 −
is the average absorption coefficient of the walls. The power thus supplied to the reverberant field is W (1 −  ) (after the first reflection). Since the power lost by the reverberant field must equal the power supplied to it for steady‐state conditions, then
) (after the first reflection). Since the power lost by the reverberant field must equal the power supplied to it for steady‐state conditions, then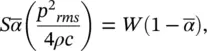
 . So,
. So,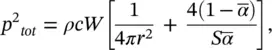

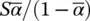 .
.










