Ronald J. Anderson - The Practice of Engineering Dynamics

1 Cover

2 Preface

3 About the Companion Website

4 Part I: Modeling: Deriving Equations of Motion 1 Kinematics 1.1 Derivatives of Vectors 1.2 Performing Kinematic Analysis 1.3 Two Dimensional Motion with Constant Length 1.4 Two Dimensional Motion with Variable Length 1.5 Three Dimensional Kinematics 1.6 Absolute Angular Velocity and Acceleration 1.7 The General Acceleration Expression 2 Newton's Equations of Motion2.1 The Study of Motion 2.2 Newton's Laws 2.3 Newton's Second Law for a Particle 2.4 Deriving Equations of Motion for Particles 2.5 Working with Rigid Bodies 2.6 Using in the Rigid Body Force Balance 2.7 Using in the Rigid Body Force Balance 2.8 Moment Balance for a Rigid Body 2.9 The Angular Momentum Vector – 2.10 A Physical Interpretation of Moments and Products of Inertia 2.11 Euler's Moment Equations 2.12 Throwing a Spiral 2.13 A Two Body System 2.14 Gyroscopic Motion 3 Lagrange's Equations of Motion 3.1 An Example to Start 3.2 Lagrange's Equation for a Single Particle 3.3 Generalized Forces 3.4 Generalized Forces as Derivatives of Potential Energy 3.5 Dampers – Rayleigh's Dissipation Function 3.6 Kinetic Energy of a Free Rigid Body 3.7 A Two Dimensional Example using Lagrange's Equation 3.8 Standard Form of the Equations of Motion

5 Part II: Simulation: Using the Equations of Motion 4 Equilibrium Solutions 4.1 The Simple Pendulum 4.2 Equilibrium with Two Degrees of Freedom 4.3 Equilibrium with Steady Motion 4.4 The General Equilibrium Solution 5 Stability 5.1 Analytical Stability 5.2 Linearization of Functions 5.3 Example: A System with Two Degrees of Freedom 5.4 Routh Stability Criterion 5.5 Standard Procedure for Stability Analysis 6 Mode Shapes 6.1 Eigenvectors 6.2 Comparing Translational and Rotational Degrees of Freedom 6.3 Nodal Points in Mode Shapes 6.4 Mode Shapes with Damping 6.5 Modal Damping 7 Frequency Domain Analysis 7.1 Modeling Frequency Response 7.2 Seismic Disturbances 7.3 Power Spectral Density 8 Time Domain Solutions 8.1 Getting the Equations of Motion Ready for Time Domain Simulation 8.2 A Time Domain Example 8.3 Numerical Schemes for Solving the Equations of Motion 8.4 Euler Integration 8.5 An Example Using the Euler Integrator 8.6 The Central Difference Method: An Method 8.7 Variable Time Step Methods 8.8 Methods with Higher Order Truncation Error 8.9 The Structure of a Simulation Program

6 Part III: Working with Experimental Data 9 Experimental Data – Frequency Domain Analysis 9.1 Typical Test Data 9.2 Transforming to the Frequency Domain – The CFT 9.3 Transforming to the Frequency Domain – The DFT 9.4 Transforming to the Frequency Domain – A Faster DFT 9.5 Transforming to the Frequency Domain – The FFT 9.6 Transforming to the Frequency Domain – An Example 9.7 Sampling and Aliasing 9.8 Leakage and Windowing 9.9 Decimating Data 9.10 Averaging DFTs

7 A Representative Dynamic SystemsA.1 System 1 A.2 System 2 A.3 System 3 A.4 System 4 A.5 System 5 A.6 System 6 A.7 System 7 A.8 System 8 A.9 System 9 A.10 System 10 A.11 System 11 A.12 System 12 A.13 System 13 A.14 System 14 A.15 System 15 A.16 System 16 A.17 System 17 A.18 System 18 A.19 System 19 A.20 System 20 A.21 System 21 A.22 System 22 A.23 System 23

8 B Moments and Products of Inertia B.1 Moments of Inertia B.2 Parallel Axis Theorem for Moments of Inertia B.3 Parallel Axis Theorem for Products of Inertia B.4 Moments of Inertia for Commonly Encountered Bodies

9 C Dimensions and Units

10 D Least Squares Curve Fitting

11 Index

12 End User License Agreement

1 Chapter 5Table 5.1 Parameter values for the two degree of freedom example.Table 5.2 Eigenvalues and eigenvectors for the two degree of freedom example.Table 5.3 The Routh table.Table 5.4 The initial Routh table for the two degrees of freedom example.Table 5.5 The final Routh table for the two degrees of freedom example.

2 Chapter 6Table 6.1 Parameters for the rigid rod example.

3 Chapter 7Table 7.1 Parameters for the linear system with two masses.

4 Chapter 8Table 8.1 Euler integration example.Table 8.2 Pendulum simulation pseudo‐code.Table 8.3 General simulation pseudo‐code.

5 Chapter 9Table 9.1 Fourier transforms computational effort.Table 9.2 Sampled data.Table 9.3 DFT coefficients.Table 9.4 DFT coefficients.

6 4Table D.1 Sample data points.

1 Chapter 1 Figure 1.1 A vector changing with time. Figure 1.2 Even 2D problems are 3D. Figure 1.3 A rigid rod rotating about a fixed point. Figure 1.4 A slider in a slot. Figure 1.5 A three dimensional robot. Figure 1.6 Relative position vectors. Figure 1.7 The velocity and acceleration components of the slider.

2 Chapter 2 Figure 2.1 A slider in a slot. Figure 2.2 Creating the Free Body Diagram of the slider. Figure 2.3 A single particle in a rigid body. Figure 2.4 Free body diagram of a single particle in a rigid body.Figure 2.5 A single mass system to help interpret inertia properties.Figure 2.6 Term 1 – a product of inertia term.Figure 2.7 Term 2 – a product of inertia term.Figure 2.8 Term 3 – a moment of inertia term.Figure 2.9 An American football in flight.Figure 2.10 A cylinder on a wedge.Figure 2.11 FBDs of the cylinder and the wedge.Figure 2.12 A spinning top.Figure 2.13 A bicycle.Figure 2.14 The “tipped” top.Figure 2.15 The bicycle turning to the right.

3 Chapter 3Figure 3.1 A mass on a wire.Figure 3.2 Gravitational force acting on a mass.Figure 3.3 Spring force acting on a mass.Figure 3.4 A linear viscous damper.Figure 3.5 A single particle in a rigid body.Figure 3.6 2D rigid body example.Figure 3.7 2D rigid body potential energy.

4 Chapter 4Figure 4.1 A simple pendulum.Figure 4.2 The equilibrium solutions for the 2D example.Figure 4.3 An eccentric rotating body.Figure 4.4 The constant acceleration case.

5 Chapter 5Figure 5.1 Stability response types.Figure 5.2 Linearization.

6 Chapter 6Figure 6.1 The higher frequency mode for the two degrees of freedom system (...Figure 6.2 The lower frequency mode for the two degrees of freedom system (0...Figure 6.3 A rigid rod supported on springs.Figure 6.4 Undamped mode shapes for the rigid rod supported on springs.Figure 6.5 Nodal points.Figure 6.6 Damped mode shapes for the rigid rod supported on springs.Figure 6.7 Calculating the damping ratio from the location of an eigenvalue....Figure 6.8 Waveforms for different percent damping.

7 Chapter 7Figure 7.1 A linear system with two masses.Figure 7.2 Amplitude response of mass 1.Figure 7.3 Phase response of mass 1.Figure 7.4 Amplitude response of mass 2.Figure 7.5 Phase response of mass 2.Figure 7.6 Seismic disturbance of a system.Figure 7.7 A measured variable 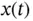plotted versus time.Figure 7.8 DFT component amplitudes.Figure 7.9 Component mean‐square values.Figure 7.10 The cumulative mean‐square value.Figure 7.11 Cumulative mean‐square curve for the example system.Figure 7.12 PSD for the example system.

8 Chapter 8Figure 8.1 The pendulum.Figure 8.2 Predicted results for the sample time domain simulation.Figure 8.3 Graphical representation of Euler's method.Figure 8.4 Solution errors as a function of time step.Figure 8.5 The midpoint method.Figure 8.6 Structure of a simulation program.

9 Chapter 9Figure 9.1 A measured variable 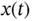plotted versus time.Figure 9.2 The square of plotted versus time.Figure 9.3 The example function, 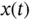, plotted versus time.Figure 9.4 The DFT amplitudes of the example function, , plotted versus fre...Figure 9.5 Aliasing.Figure 9.6 The folding frequency.Figure 9.7 Aliased DFT results.Figure 9.8 The DFT for the first example (no leakage).Figure 9.9 The DFT for the second example (leakage).Figure 9.10 CFT approximation to the square wave.Figure 9.11 The Hanning window.Figure 9.12 The data from Equation 9.83.Figure 9.13 The windowed data.Figure 9.14 The DFT for the second example with windowing.