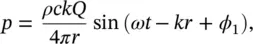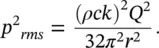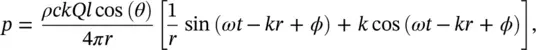In a certain factory space the noise level with an electric motor in operation is 93 dB. When the motor is turned off the background noise is 85 dB. What is the sound pressure level due to the motor?
Since 10 93/10= 10 85/10+ 10 Lp/10, then L p= 10log(10 9.3–10 8.5) = 92.2 dB.
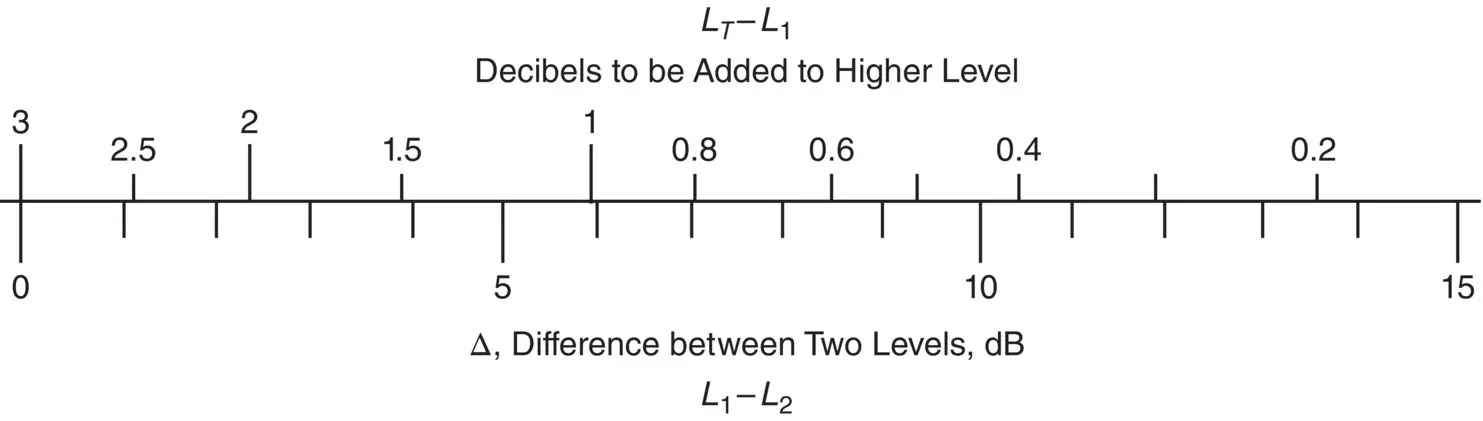
Figure 3.6 Diagram for combination of two sound pressure levels or two sound power levels of uncorrelated sources.
3.5 Three‐dimensional Wave Equation
In most sound fields, sound propagation occurs in two or three dimensions. The three‐dimensional version of Eq. (3.1)in Cartesian coordinates is
(3.29) 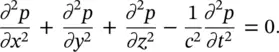
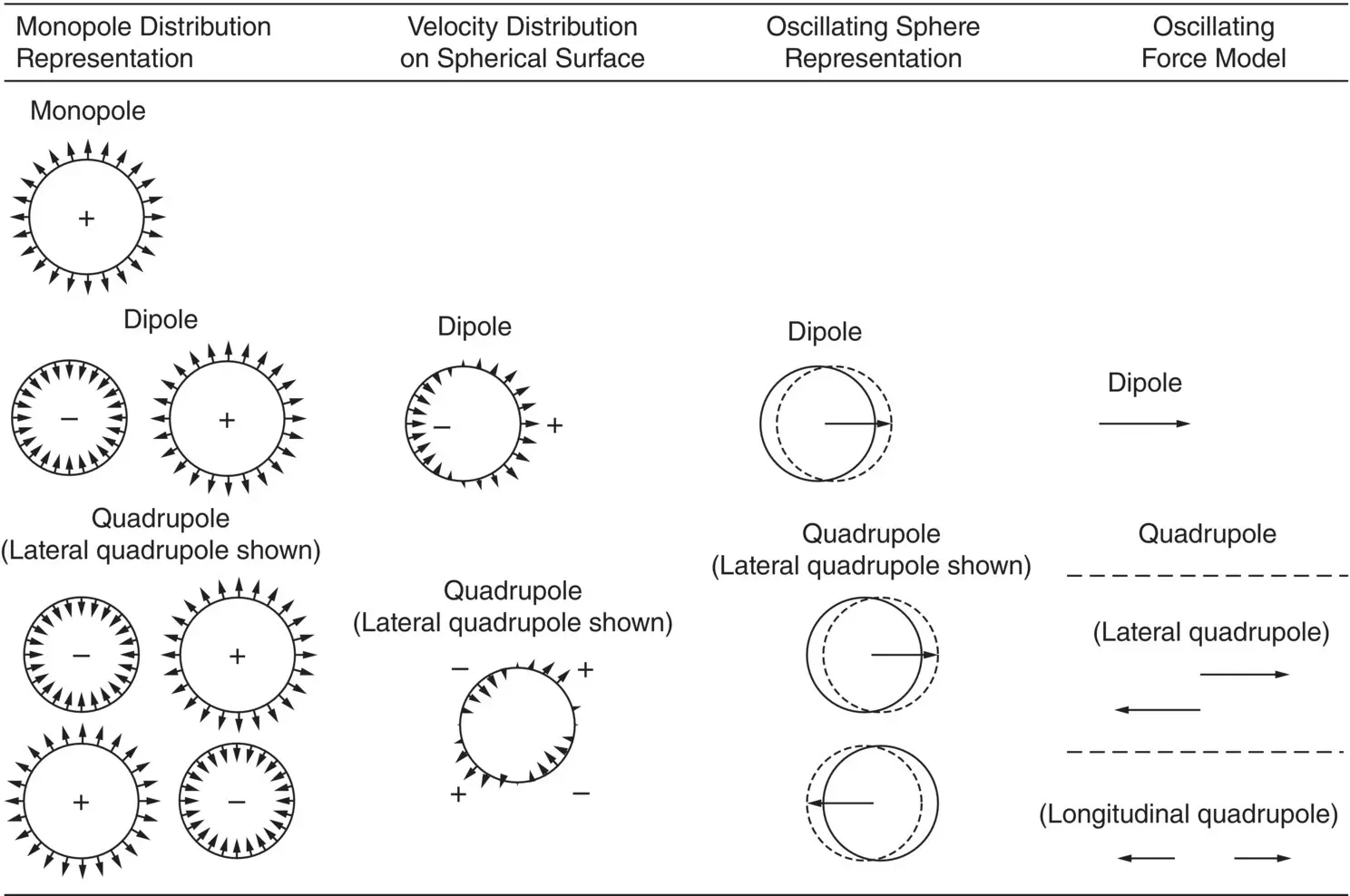
This equation is useful if sound wave propagation in rectangular spaces such as rooms is being considered. However, it is helpful to recast Eq. (3.29)in spherical coordinates if sound propagation from sources of sound in free space is being considered. It is a simple mathematical procedure to transform Eq. (3.29)into spherical coordinates, although the resulting equation is quite complicated. However, for propagation of sound waves from a spherically symmetric source (such as the idealized case of a pulsating spherical balloon known as an omnidirectional or monopole source) ( Table 3.1), the equation becomes quite simple (since there is no angular dependence):
(3.30) 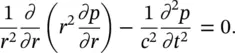
Table 3.1 Models of idealized spherical sources: Monopole, Dipole, and Quadrupole a.
aFor simple harmonic sources, after one half‐period the velocity changes direction; positive sources become negative and vice versa, and forces reverse direction with dipole and quadrupole force models.
After some algebraic manipulation Eq. (3.30)can be written as
(3.31) 
Here, r is the distance from the origin and p is the sound pressure at that distance.
Equation (3.30)is identical in form to Eq. (3.1)with p replaced by rp and x by r . The general and simple harmonic solutions to Eq. (3.30)are thus the same as Eqs. (3.4)and (3.5)with p replaced by rp and x with r . The general solution is
(3.32) 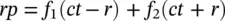
or
(3.33) 
where f 1and f 2are arbitrary functions. The first term on the right of Eq. (3.33)represents a wave traveling outward from the origin; the sound pressure p is seen to be inversely proportional to the distance r . The second term in Eq. (3.33)represents a sound wave traveling inward toward the origin, and in most practical cases such waves can be ignored (if reflecting surfaces are absent).
The simple harmonic (pure‐tone) solution of Eq. (3.31)is
(3.33) 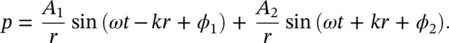
We may now write that the constants A 1and A 2may be written as 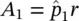 and
and 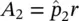 , where
, where 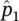 and
and  are the sound pressure amplitudes at unit distance (usually 1 m) from the origin.
are the sound pressure amplitudes at unit distance (usually 1 m) from the origin.
The second term on the right of Eq. (3.33), as before, represents sound waves traveling inward to the origin and is of little practical interest. However, the first term represents simple harmonic waves of angular frequency ω traveling outward from the origin, and this may be rewritten as [4]
(3.34) 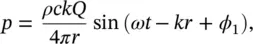
where Q is termed the strength of an omnidirectional ( monopole ) source situated at the origin, and Q = 4 πA 1 /ρck . The mean‐square sound pressure p 2 rmsmay be found [4] by time averaging the square of Eq. (3.34)over a period T :
(3.35) 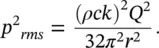
From Eq. (3.35), the mean‐square pressure is seen to vary with the inverse square of the distance r from the origin of the source, for such an idealized omnidirectional point sound source, everywhere in the sound field. Again, this is known as the inverse square law . If the distance r is doubled, the sound pressure level decreases by 20log(2) = 20(0.301) = 6 dB. If the source is idealized as a sphere of radius a pulsating with a simple harmonic velocity amplitude U , we may show that Q has units of volume flow rate (cubic metres per second). If the source radius is small in wavelengths so that a ≤ λ or ka ≤ 2 π , then we can show that the strength Q = 4 πa 2 U .
Many sources of sound are not like the simple omnidirectional monopole source just described. For example, an unbaffled loudspeaker produces sound both from the back and front of the loudspeaker. The sound from the front and the back can be considered as two sources that are 180° out of phase with each other. This system can be modeled [13, 14] as two out‐of‐phase monopoles of source strength Q separated by a distance l . Provided l ≪ λ , the sound pressure produced by such a dipole system is
(3.36) 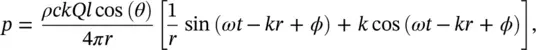
where θ is the angle measured from the axis joining the two sources (the loudspeaker axis in the practical case). Unlike the monopole, the dipole field is not omnidirectional. The sound pressure field is directional. It is, however, symmetric and shaped like a figure‐eight with its lobes on the dipole axis, as shown in Figure 3.11b.
Читать дальше


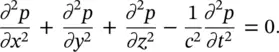
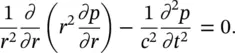

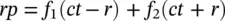

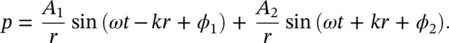
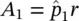 and
and 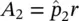 , where
, where 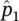 and
and  are the sound pressure amplitudes at unit distance (usually 1 m) from the origin.
are the sound pressure amplitudes at unit distance (usually 1 m) from the origin.