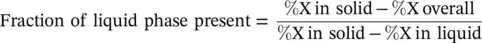Nirmal K. Sinha - Engineering Physics of High-Temperature Materials

Another key point on such phase diagrams is the end point of a phase equilibrium curve – called a “critical point.” Figure 2.3demonstrates a common critical point where the liquid and gaseous states become indistinguishable and are often referred to as a supercritical fluid. In the vicinity of a critical point, the physical properties of the liquid and vapor can dramatically change as they become more similar. Historically, a solid–liquid critical point has generally not been accepted (Landau and Lifshitz 1980); however, this has recently been challenged – largely through molecular dynamics simulations (Elenius and Dzugutov 2009; Mochizuki and Koga 2015).

It should be mentioned that the phase diagram of a single compound is not necessarily a single‐component system. Polymorphism can give rise to intricate phase diagrams for single compounds. For example, the phase diagram of water is quite complex and discussed further in Section 2.6.

Phase diagrams for mixtures of chemically independent components can quickly become increasingly complex. For a simple binary mixture, the open spaces in a pressure–temperature phase diagram would have C = 2 and P = 1 to give three degrees of freedom. The third degree of freedom is the composition of one of the two components. For this reason, phase diagrams for binary systems are generally expressed as temperature versus composition at a given pressure (often standard pressure), as shown in Figure 2.4. Engineers will often express the composition in terms of the weight percent of the components while chemists will often express it in terms of the mole fraction of the component.

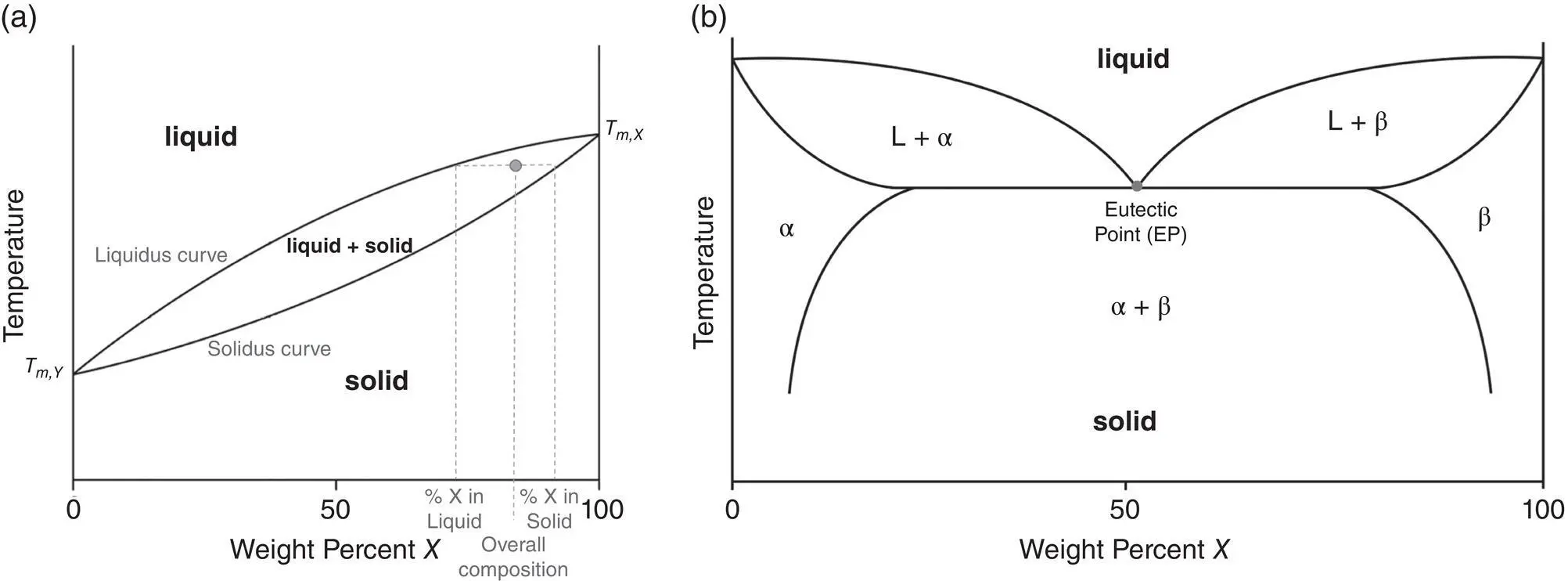
Figure 2.4 Sample phase diagram schematic for a binary XY system. (a) Simple phase diagram demonstrating how to determine the composition present in the different phases at a point in the mixed phase region. (b) Slightly more complex phase diagram of an XY system containing a eutectic point.

Phase diagrams for binary systems contain a large amount of information. Not only do they give an indication of the phases present, but also provide information about the composition of the phases and fraction of the phases present in the mixture. For example, the point of interest highlighted in Figure 2.4a has both a liquid phase and a solid phase present. The composition of the phases can be determined by drawing a tie‐line from the point to the liquidus and solidus curves and then reading the percent composition at the intersections. The ratio of the liquid/solid present can then be determined using the two phase compositions and the overall composition, using the lever rule:

(2.2)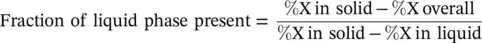

Figure 2.4b presents a typical phase diagram of a eutectic system. A eutectic system is a homogeneous mixture of substances that melts or solidifies at a single temperature that is lower than the melting point of any of the constituents. At a eutectic point, the liquid and two solid solutions all coexist in chemical equilibrium. Cooling through this temperature results in intricate macrostructures that can take several different forms, including lamellar, rod‐like, globular, or acicular (needle‐like) structures. Other critical points in a binary system include:

Eutectoid: Transformation of a solid phase to yield two solid phases.

Peritectic: Transformation of a liquid phase and a solid phase to yield a single solid phase.

Peritectoid: Transformation of two solid phases in an alloy system to yield a new solid phase.

Binary systems can be intricate with multiple types of transformations available to the system at different compositions. Further increasing the number of components can quickly make materials' systems very complex. Phase diagrams can thus be incredibly useful in designing materials to have specific phase compositions.

Phase diagrams can be incredibly important in engineering materials, but they do not always tell the whole story. Phase diagrams generally present equilibrium phases. Nonequilibrium phases, such as phases stabilized through quenching or even a more complex environmental history, can often be critical to achieving properties of interest. Time–temperature–transformation diagrams that plot the time required for isothermal transformation provide kinetic information about transformation processes and can be highly useful in developing synthesis processes; however, these diagrams must still be interpreted with the assistance of phase diagrams.

An individual crystal or grain in the solid phase consists of atoms aligned in space in definite patterns over long distances until it impinges on the border of another crystal. In reality, solids are not perfect. There are many different kinds of imperfections within a solid that play an important, and often determining, role in the properties of the material. Crystal imperfections thus play an important role in materials science and its use across disciplines.

Understanding imperfections and their impact on materials' properties is extraordinarily complex and dependent on the system under question. Here, we strive only to give a brief overview of some high‐level key features that will enable us to understand aspects presented later in this text.

Point defects are imperfections that arise from a single central site, but cause strain in a small volume around them and can impact the properties of the bulk. They include a variety of imperfections, such as those shown in Figure 2.5: (i) vacancies – empty lattice sites that can impact diffusion; (ii) interstitials – generally smaller atoms located in between normal lattice sites that impact a variety of properties, such as strength, hardness ductility, and yield strength; and (iii) substitutions – solute atoms located at a lattice site that can also impact strength and hardness.

Point defects can often occur in pairs or sets. For example, in ionic crystals, point defects can occur in pairs that maintain stoichiometry or charge neutrality, such as Schottky defects (vacancies of ions with opposite charge) or Frenkel defects (a vacancy and an interstitial). A high density of point defects, particularly vacancies, can give rise to nonstoichiometric compounds. Such compounds often consist of transition metals, lanthanides, and actinides paired with polarizable anions, such as oxides and sulfides, e.g. Fe xO and Cu xS. Point defects can be naturally occurring, such as the substitutional impurities that give rise to beautiful gems, e.g. sapphires and rubies. Point defects can also be designed into the material to tailor its properties, such as doping semiconductors to control their electrical conductivity.

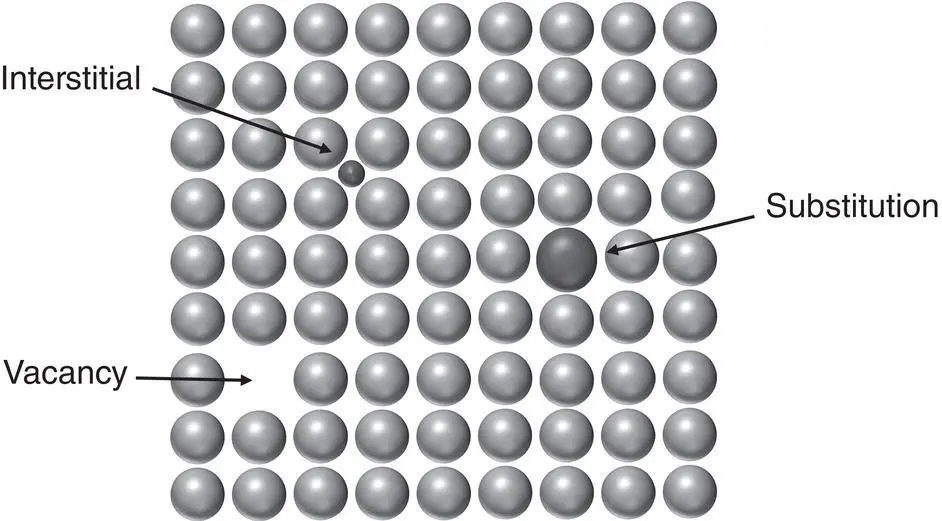
Figure 2.5 Schematic of common point defects in crystals, including a vacancy, an interstitial, and a substitution.

Line defects or dislocations are imperfections in the linear arrangement of atoms in the crystal. There are two main types of dislocations that represent the extreme forms of line defects: edge dislocations and screw dislocations. Dislocations play an important role in plastic deformation and allow deformation to occur at lower stresses than would be anticipated in a perfect crystal as only a few atoms are moved from their equilibrium positions at a given time.