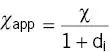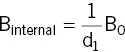
Figure 2.10 Domains in a ferromagnetic material as the external field is increased from zero to the saturation point.
The B‐field in the material increases in the presence of an external field H (or B). The slope of the curve gives the magnetic permeability μ, its value depending upon the strength of H applied. For low values of external field, the magnetization is reversible. Above the saturation point the material becomes unable to sustain a higher magnetization no matter how large the applied field is. This material is magnetically saturated . For most ferromagnetic materials this occurs below 1.5 T, so it is likely that a potential projectile will be saturated when very close to the magnet bore entrance. Figure 2.11shows the B‐H curve for series 416 stainless steel, a material commonly used in domestic goods. Also shown is the dependence of its magnetic permeability μ upon the external field H.

Figure 2.11 B‐H curve for 416 stainless steel (blue line) and its magnetic permeability μ (red line). μ is a function of H. The metal saturates at 1.85 T. The external field H = B 0/μ 0.
As the applied H‐field is reduced (or the material is removed from the external B 0‐field of your magnet) the internal B within the material decreases. Due to hysteresis , it does not decrease exactly along the path of its increase ( Figure 2.12). With a hard ferromagnetic material significant B remains once the external field has been reduced to zero. This is known as the remanence , B rem. Permanent magnets have high remanence. If the H is applied in the opposite direction (i.e. is made negative) the material’s B continues to fall. The intercept H con the ‐H axis is called the coercivity . Soft materials have low coercivity and remanence with a slim B‐H (or M‐H) curve. In hard ferromagnetic materials both are large and the hysteresis curve is broader. Above the Curie temperature , metals lose their ferromagnetic properties. Values of B sat, H cand Curie temperatures are shown in Table 2.2.
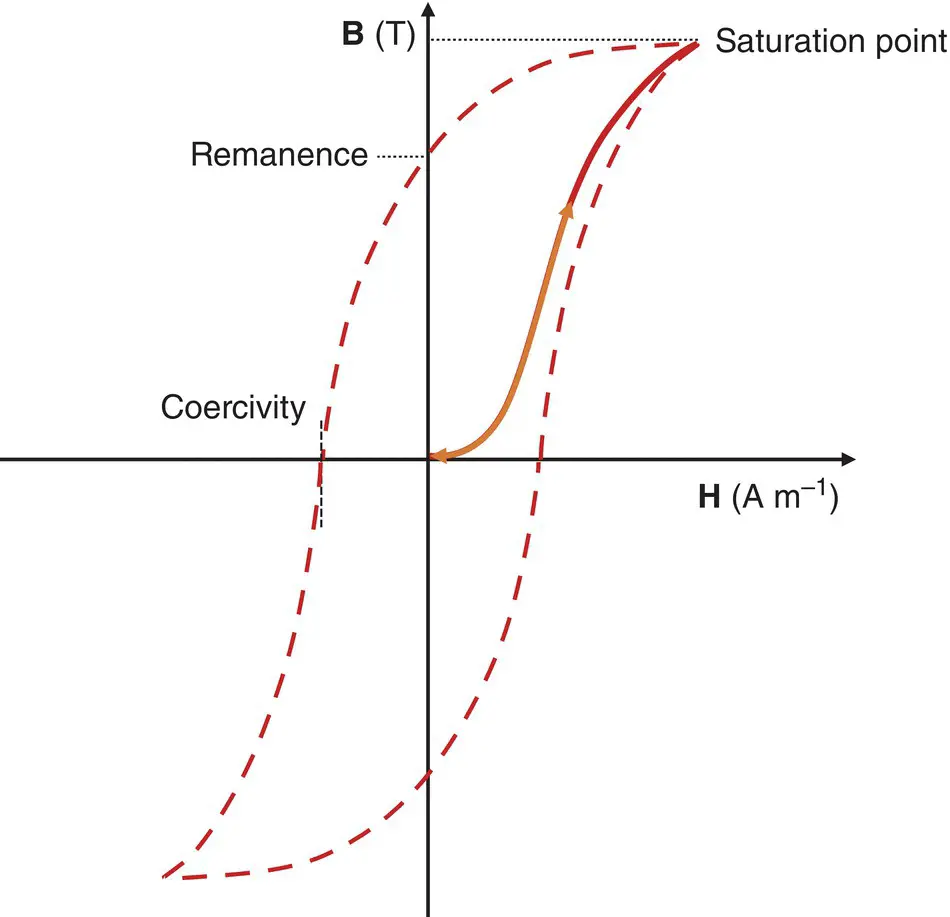
Figure 2.12 The hysteresis curve for a ferromagnetic material. The orange line represents increases in field which are reversible. Soft ferromagnetic materials have a slim curve with low remanence and coercivity. Hard ferromagnetic materials have a broader curve with higher remanence and coercivity.
Table 2.2 Properties of ferromagnetic metals.
| Soft Ferromagnetic |
H C(A M −1) |
B sat(T) |
Curie temperature (K) |
| Silicon steel |
40–70 |
2.0 |
750 |
| Mumetal (Ni-Fe alloy) |
0.6–1.0 |
0.77 |
350 |
| Iron |
12–400 |
1.7–2.2 |
770 |
| Nickel |
400 |
0.62 |
358 |
| 400 series stainless steels |
130–480 |
1.2–1.4 |
– |
| Hard Ferromagnetic |
| 5% Chromium steel |
5×10 3 |
0.94 |
760–850 |
| Alnico (Al-Ni-Co alloy) |
50×10 3 |
0.56–1.35 |
973–1133 |
| Supermagloy (Sm-Co alloy) |
700×10 3 |
1.50 |
993–1073 |
Demagnetizing field and factors
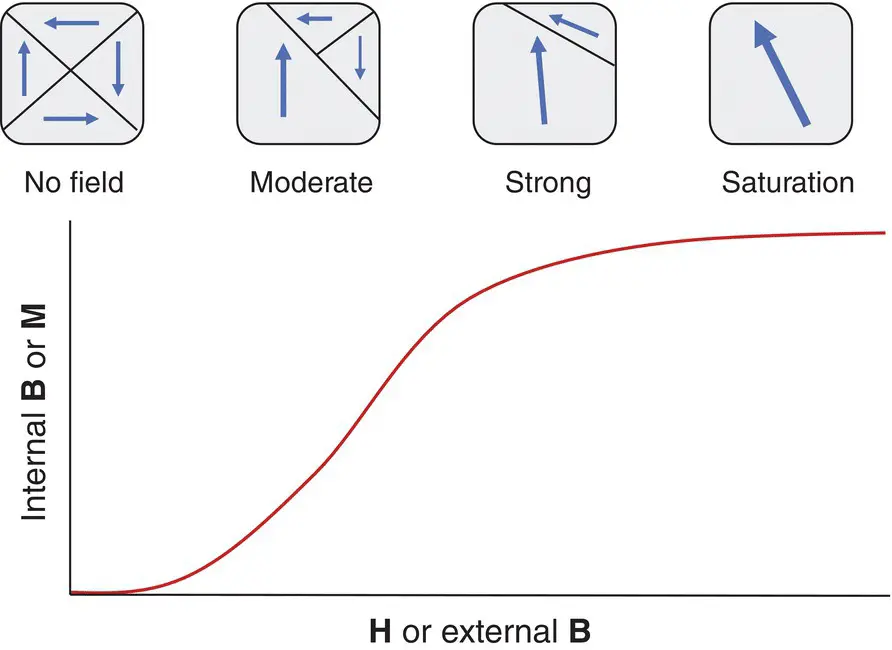
An object’s shape adds a further layer of complexity with great significance for magnetic forces and torques, and hence for MRI safety. Figure 2.13shows how magnetization of the object in an external B 0generates “virtual poles”, resulting in a “de‐magnetization field” which reduces the apparent value of susceptibility χ app:
(2.8) 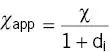

Figure 2.13 Demagnetization field within objects magnetized by an external magnetic field: the flatter object (left) creates more ‘virtual poles’ and develops a greater degree of demagnetization.
where d iis the demagnetizing factor . For simple shapes (ellipsoids of rotation, cuboids and cylinders) there are three demagnetizing factors: d 1along the principal axis, d 2and d 3along the minor axes. Representative values are shown in Table A1.1 in Appendix 1. Figure 2.14shows the values of 1/d 1for the axial and 1/d 2(=1/d 3) for the radial axes of cylindrical objects. It is the demagnetizing factor that determines the level of magnetization of a ferromagnetic object, rather than the value of χ. For an unsaturated sphere the internal B field is three times the external B 0. For elongated objects the internal B can be significantly greater. As a simple rule of thumb, for a cylinder whose length is aligned with B 0
(2.9a) 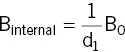
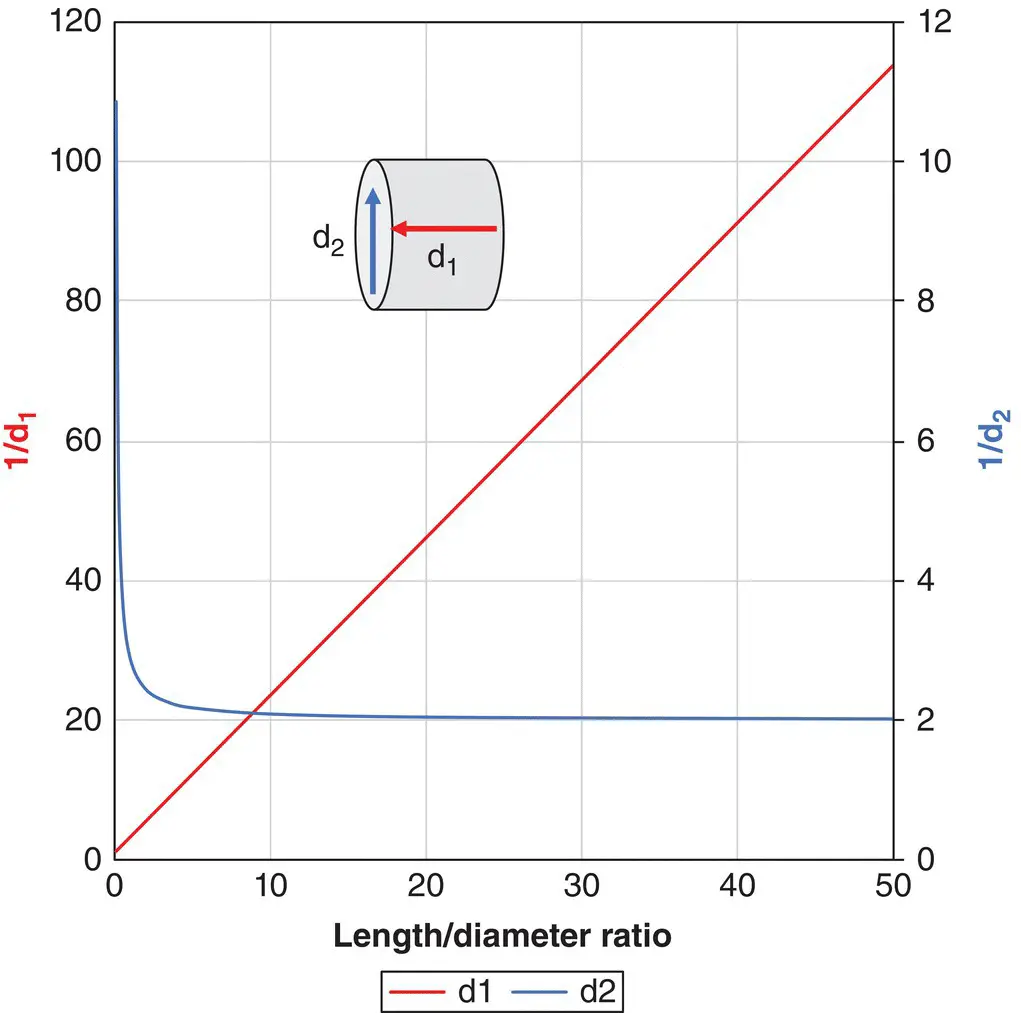
Figure 2.14 Reciprocal demagnetization factors for a cylinder: 1/d 1(axial, red line), 1/d 2(radial, blue line). For a cylinder d 3= d 2.
If the object is rotated 90° to B 0
(2.9b) 
Figure 2.15shows the theoretical internal B for ferromagnetic cylindrical objects of various length‐diameter (l/d) ratios and a sphere as they approach 1.5 and 3 T shielded magnets. In each case the saturation value B sat(=1.6 T) is reached at greater distances from the magnet for the more elongated objects.
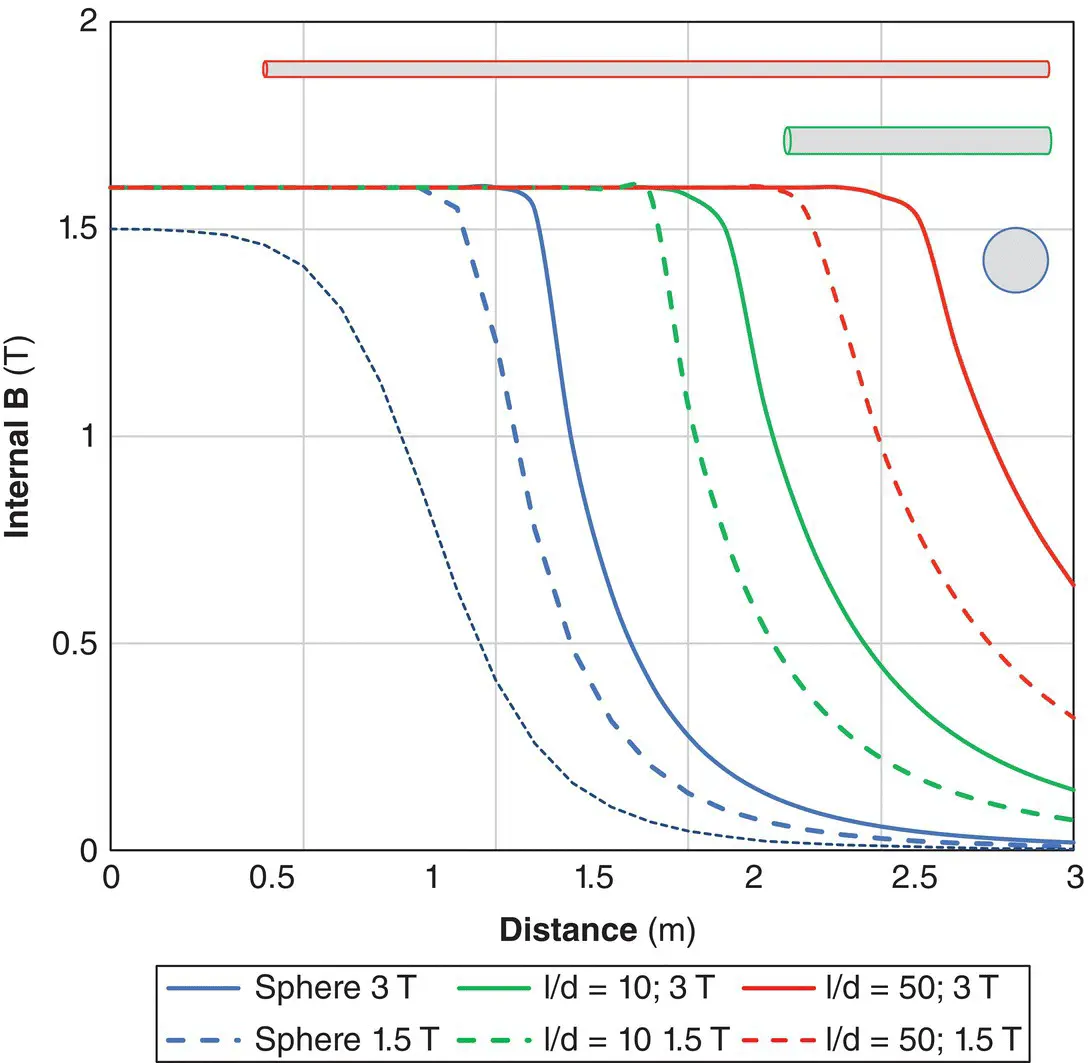
Figure 2.15 Predicted internal B for a ferromagnetic sphere and cylinders of differing length/diameter ratios in the approach to 1.5 and 3 T MRI magnets. The material saturates at 1.6 T. The bore entrance is at 0.8 m. The dotted gray line indicates the B 0field strength.
Demagnetizing factors and B satare crucial for determining the force and torque on different shaped objects within the scanner’s fringe field.
The internal magnetic field or degree of magnetization of a ferromagnetic object is not determined by its magnetic susceptibility but by its demagnetization factors and saturation status.
Example 2.1 Magnetization of a nickel coin
A nickel coin (length = 1 mm, diameter = 1 cm) is inadvertently brought into the MRI examination room. If the external field is 100 mT what is the field within the coin if it is: (a) lying face on to the magnet; (b) edge on to the magnet? Will the coin’s metal saturate?
Читать дальше