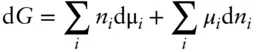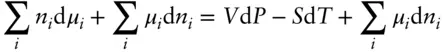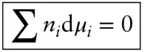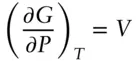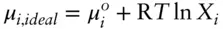William M. White - Geochemistry

The differential form of this equation (which we get simply by applying the chain rule) is:

(3.20)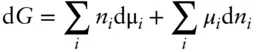

Equating this with eqn. 3.14, we obtain:

(3.21)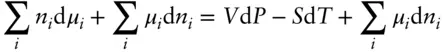

Rearranging, we obtain the Gibbs–Duhem relation :

(3.22)

The Gibbs–Duhem equation describes the relationship between simultaneous changes in pressure, temperature, and composition in a single-phase system. In a closed system at equilibrium, net changes in chemical potential will occur only as a result of changes in temperature or pressure. At constant temperature and pressure , there can be no net change in chemical potential at equilibrium:

(3.23)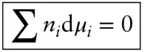

This equation further tells us that the chemical potentials do not vary independently but change in a related way . In a closed system, only one chemical potential can vary independently. For example, consider a two-component system. Then we have n 1d μ 1+ n 2d μ 2= 0 and d μ 2= −( n 1/ n 2)d μ 1. If a given variation in composition produces a change in μ 1then there is a concomitant change in μ 2.

For multiphase systems, we can write a version of the Gibbs–Duhem relation for each phase in the system. For such systems, the Gibbs–Duhem relation allows us to reduce the number of independently variable components in each phase by one. We will return to this point later in the chapter.

We can now state an additional property of chemical potential:

In spontaneous processes, components or species are distributed between phases so as to minimize the chemical potential of all components.

This allows us to make one more characterization of equilibrium: equilibrium is the point where the chemical potential of all components is minimized.

Another significant aspect of the Gibbs–Duhem equation is that the phase rule can be derived from it. We begin by recalling that the variance of a system (the number of variables that must be fixed or independently determined to determine the rest) is equal to the number of variables minus the number of equations relating them. In a multicomponent single-phase system, consisting of c components, there are c +2 unknowns required to describe the equilibrium state of the system: T, P, μ 1 , μ 2 , ...μ c. But in a system of φ phases at equilibrium, we can write φ versions of eqn. 3.23, which reduces the independent variables by φ . Thus, the number of independent variables that must be specified to describe a system of c components and φ phases is:

which is the Gibbs phase rule.

Specification of ƒ variables will completely describe the system, at least with the qualification that in thermodynamics we are normally uninterested in the size of the system, that is, in extensive properties such as mass and volume (though we are interested in their intensive equivalents), and outside forces or fields such as gravity, electric, or magnetic fields. Nevertheless, the size of the system is described as well, provided that only one of the ƒ variables is extensive.

Having placed another tool, the chemical potential, in our thermodynamic toolbox, we are ready to continue our consideration of solutions. We will begin with ideal solutions , which, like ideal gases, are fictions that avoid some of the complications of real substances. For an ideal solution, we make an assumption similar to one of those made for an ideal gas, namely that there are no forces between molecules. In the case of ideal solutions, which may be gases, liquids, or solids, we can relax this assumption somewhat and require only that the interactions between different kinds of molecules in an ideal solution are the same as those between the same kinds of molecules .

How does chemical potential vary in an ideal solution? Consider the vapor pressure of a gas. The derivative of G with respect to pressure at constant temperature is volume:

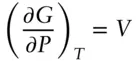
Written in terms of partial molar quantities:

If the gas is ideal, then:

(3.24)

and if we integrate from P° to P we obtain:

(3.25)

where μ P°is the chemical potential of the pure gas at the reference (standard state) pressure P° . This is the standard-state chemical potential and is written as μ° . If we let P° be the vapor pressure of pure i and P be the vapor pressure of i in an ideal solution, then we may substitute X for P/P° into Raoult's law ( eqn. 3.8) to obtain the following:

(3.26)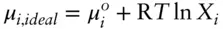

This equation describes the relationship between the chemical potential of component i and its mole fraction in an ideal solution.

We will be able to generalize a form of this equation to nonideal cases a bit later. Let's first consider some other properties of ideal mixtures. For real solutions, any extensive thermodynamic property such as volume can be considered to be the sum of the volume of the components plus a volume change due to mixing:

(3.27)

The first term on the right reflects the volume resulting from mechanical mixing of the various components. The second term reflects volume changes associated with solution. For example, if we mixed 100 ml of ethanol and 100 ml of water ( Figure 3.5), the volume of the resulting solution would be 193 ml. Here, the value of the first term on the right would be 200 ml, and the value of the second term would be −7 ml. We can write similar equations for enthalpy, and so on. But the volume change and enthalpy change due to mixing are both 0 in the ideal case. This is true because both volume and enthalpy changes of mixing arise from intermolecular forces, and, by definition, such intermolecular forces are absent in the ideal case. Thus: