William M. White - Geochemistry

Imagine a well-insulated box (an isolated system) somewhere in the universe ( Figure 2.4). Imagine that within the box are two gases, separated by a removable partition. If we remove the partition, what happens? You know: the two gases mix completely. The process is entirely spontaneous. We have neither added energy to nor taken energy from the system, hence the first law says nothing about this process. Nor did removing the partition “cause” the reaction. This is apparent from the observation that if we reinsert the partition, the gases do not unmix. That you knew that the gases would mix (and knew as well that they would not unmix upon reinserting the partition) suggests there is something very fundamental and universal about this. We need a physical law that describes it. This is the second law.

The second law may be stated in a number of ways:

Figure 2.4 A gas-filled box with a removable partition. When the partition is removed, the gases mix. Entropy increases during this process.

It is impossible to construct a machine that is able to convey heat by a cyclical process from one reservoir at a lower temperature to another at a higher temperature unless work is done by some outside agency (i.e., air conditioning is never free) . †

Heat cannot be entirely extracted from a body and turned into work (thus car engines always have cooling systems) .

Every system left to itself will, on average, change toward a condition of maximum probability .

Introducing a new state function Scalled entropy , we may state the second law as:

The entropy of the universe always increases .

In colloquial terms we could say:

You can't shovel manure into the rear end of a horse and expect to get hay out its mouth .

The second law codifies some of our everyday experience. The first law would not prevent us from using a horse to manufacture hay from manure. It only says we cannot get more joules worth of hay out than we put in as manure. We would search in vain for any other physical law that prohibited this event. Yet our experience shows that it will not happen. Indeed, this event is so improbable that we find it comical. Similarly, we know that we can convert gasoline and oxygen to carbon dioxide and water in an internal combustion engine and use the resulting energy to drive a vehicle down the road. But adding CO 2and water to the engine and pushing the car backwards down the street does not produce gasoline and oxygen, although such a result violates no other law of physics. The second law states that there is a natural direction in which reactions will tend to proceed . This direction is inevitably that of higher entropy of the system and its surroundings.

Whereas energy is a property for which we gain an intuitive feel through everyday experience, the concept of entropy is usually more difficult to grasp. Perhaps the best intuitive understanding of entropy can be obtained from the microscopic viewpoint of statistical mechanics. So for that reason, we will make the first of several brief excursions into the world of atoms, molecules, and quanta.

Let's return to our box of gas and consider what happens on a microscopic scale when we remove the partition. To make things tractable, we'll consider that each gas consists of only two molecules, so there are four all together, two red and two black. For this thought experiment, we will keep track of the individual molecules so we label them 1red, 2red, 1black, 2black. Before we removed the partition, the red molecules were on one side and the black ones on the other. Our molecules have some thermal energy, so they are free to move around. So by removing the partition, we are essentially saying that each molecule is equally likely to be found in either side of the box.

Before we removed the partition, there was only one possible arrangement of the system; this is shown in Figure 2.5a. Once we remove the partition, we have four molecules and two subvolumes, and a total of 2 4= 16 possible configurations ( Figure 2.5b) of the system. The basic postulate of statistical mechanics is: a system is equally likely to be found in any of the states accessible to it . Thus, we postulate that each of these configurations is equally likely. Only one of these states corresponds to the original one (all red molecules on the left). Thus, the probability of the system being found in its original state is 1/16. That is not particularly improbable. However, suppose that we had altogether a mole of gas (≈6 × 10 23molecules). The probability of the system ever being found again in its original state is then ≈2×10 24, which is unlikely indeed.

Now consider a second example. Suppose that we have two copper blocks of identical mass at different temperatures and separated by a thermally insulating barrier ( Figure 2.6). Imagine that our system, which is the two copper blocks, is isolated in space so that the total energy of the system remains constant. What happens if we remove the insulating barrier? Experience tells us that the two copper blocks will eventually come into thermal equilibrium, and their temperatures will eventually be identical.

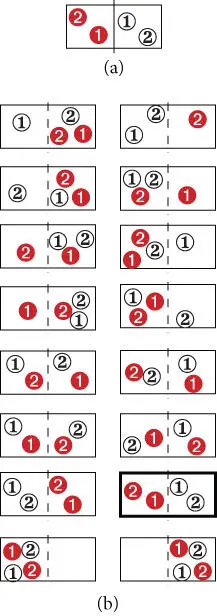
Figure 2.5 Possible distribution of molecules of a red and a black gas in a box before (a) and (b) after removal of a partition separating them.

Now let's look at this process on a microscopic scale. We have already mentioned that temperature is related to internal energy. As we shall see, this relationship will differ depending on the nature and mass of the material of interest, but since our blocks are of identical size and composition, we can assume that temperature and energy are directly related in this case. Suppose that before we remove the insulation, the left block has one unit of energy and the right one has five units (we can think of these as quanta, but this is not necessary). The question is, how will energy be distributed after we remove the insulation?

Figure 2.6 Two copper blocks at different temperatures separated by an insulator. When the insulator is removed and the blocks brought in contact, the blocks come to thermal equilibrium. Entropy increases in this process.

In the statistical mechanical viewpoint, we cannot determine how the energy will be distributed; we can only compute the possible ways it could be distributed. Each of these energy distributions is then equally likely according to the basic postulate. So let's examine how it can be distributed. Since we assume that the distribution is completely random, we proceed by randomly assigning the first unit to either the left or right block, then the second unit to either, and so on. With six units of energy, there are already more ways of distributing it (2 6= 64) than we have space to enumerate here. For example, there are six ways energy can be distributed so that the left block has one unit and the right one has five units. This is illustrated in Figure 2.7. However, since we can't actually distinguish the energy units, all these ways are effectively identical. There are 15 ways, or combinations, to distribute the energy so that the left block has two units and the right has four units. Similarly, there are 15 combinations where the left block has four units and the right has two units. For this particular example, the rule is that if there are a total of E units of energy, e of which are assigned to the left block and ( E–e ) to the right, then there will be Ω( e ) identical combinations where Ω( e ) is calculated ‡as: