Mickael Launay - It All Adds Up

Pythagoras was born at the start of the sixth century BC on the island of Samos, situated off the coast of what is now Turkey, and less than 40 kilometres away from the town of Miletus. As a young man he gained experience through his travels in the ancient world, and chose to settle in the town of Croton, in the south-east of present-day Italy. It was there that he founded his school in 532 BC.

Pythagoras and his disciples were not just mathematicians and scholars, but also philosophers, monks and politicians. Yet it must be said that if we were to transpose it to our time, the community begun by Pythagoras would undoubtedly be perceived to be among the most obscure and most dangerous of sects. The life of the Pythagoreans was governed by a set of precise rules. For example, anyone wishing to join the school had to go through a period of five years of silence. The Pythagoreans had no individual possessions: all their belongings were shared. They used various symbols such as the tetractys or the pentagram in the shape of a star with five branches to recognize one another. Moreover, the Pythagoreans thought of themselves as enlightened people, and thought it normal that political power should come to them. They firmly repressed the rebellions of towns that refused to accept their authority. In fact, it was in one such riot that Pythagoras died at the age of eighty-five.

The number of myths of all kinds that were invented around Pythagoras is also impressive. His disciples were scarcely lacking in imagination, as we can now see. According to them, Pythagoras was the son of the god Apollo. The name Pythagoras also means literally ‘he who was announced by the Oracle’: the Oracle of Delphi was in fact the oracle of the temple of Apollo, and it is she who is said to have told Pythagoras’ parents about the forthcoming birth of their child. According to the Oracle, Pythagoras would be the most handsome and wisest of men. After such a birth, the Greek scholar was predestined for great things. Pythagoras remembered all his previous lives. According to this, he had in particular been one of the heroes of the Trojan War called Euphorbus. In his youth, Pythagoras took part in the Olympic Games and took the laurels in all the pygmachia events (pugilism, the ancestor of our boxing). Pythagoras invented the very first musical scales. Pythagoras was able to walk in the air. Pythagoras died and was resuscitated. Pythagoras was a talented soothsayer and healer. Pythagoras had control over animals. Pythagoras had a golden thigh.

While most of these legends are so far-fetched that no one believes them, in other cases the jury is still out. Is it true, for example, that Pythagoras was the first to use the word ‘mathematics’? The facts are so sketchy that some historians have even come to speculate that Pythagoras was a purely fictional person, dreamed up by the Pythagoreans to serve as their tutelary figure.

Therefore, since it is not possible to learn more about the man, let us return to the subject for which he is still known to schoolchildren the whole world over more than 2,500 years after his death: Pythagoras’ theorem. What does this famous theorem tell us? Its statement may seem astonishing, because it establishes a link between two apparently unconnected mathematical concepts: right-angled triangles and the square numbers.

Let us return to our favourite triangle, the 3-4-5. From the lengths of the three sides we can construct three square numbers: 9, 16 and 25.

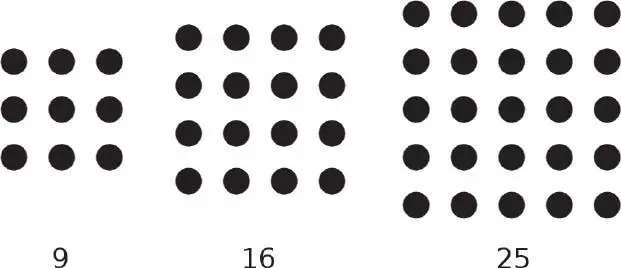
We now spot a curious coincidence: 9 + 16 = 25. The sum of the squares of sides 3 and 4 is equal to the square of side 5. This might be put down to chance, yet if we try to reproduce this calculation for a different right-angled triangle, it still works. Consider, for example, the triangle 65-72-97, which appears on the Babylonian Plimpton tablet. The three corresponding squares are 4,225, 5,184 and 9,409. And Pythagoras’ theorem does not fail: 4,225 + 5,184 = 9,409. For such large numbers, it becomes difficult to believe in a simple coincidence.

You can try with all the right-angled triangles you like, small or large, fat or thin, it always works. In a right-angled triangle, the sum of the squares of the two sides that form the right angle is always equal to the square of the third side (which we call the hypotenuse). And it also works the other way round: if, in a given triangle, the sum of the squares of the two smallest sides is equal to the square of the largest side, then the triangle is right-angled. That is Pythagoras’ Theorem.

Of course, we do not know for certain whether Pythagoras or his disciples actually contributed to this theorem. Even though the Babylonians never expressed it in the general form that we have just seen, it is highly likely that they already knew this result more than one thousand years earlier. For otherwise, how would they have been able to discover all the right-angled triangles that appear on the Plimpton tablet with such accuracy? The Egyptians and the Chinese probably also knew the theorem, which was also clearly stated in the commentaries that were added to the Nine Chapters in the centuries after it was written.

Some accounts claim that Pythagoras was the first to give a proof of the theorem. However, there is no reliable source to confirm this, and the oldest proof that has come down to us is only the one in Euclid’s Elements , which was written three centuries later.

Текст предоставлен ООО «ЛитРес».

Прочитайте эту книгу целиком, купив полную легальную версию на ЛитРес.

Безопасно оплатить книгу можно банковской картой Visa, MasterCard, Maestro, со счета мобильного телефона, с платежного терминала, в салоне МТС или Связной, через PayPal, WebMoney, Яндекс.Деньги, QIWI Кошелек, бонусными картами или другим удобным Вам способом.