Katherine Kula - Cephalometry in Orthodontics

One of the issues in determining craniofacial changes with early cephalometrics was the selection of cephalometric reference parameters called planes to compare skeletal and dental changes. For example, early in the development of cephalometry, William B. Downs 19realized that numerous measures were being used to describe the face. He sought to determine the range and the correlations of cephalometric values for individuals with excellent occlusions by comparing various cephalometric values from a group of 10 male and 10 female potentially growing adolescents (12 to 17 years old). Downs eventually came to the conclusion that he should evaluate the face by dividing the facial skeleton from the teeth and the alveolar processes. He would classify the skeletal pattern (maxilla versus mandible) alone and then determine the relationship of the teeth and alveoli to the facial skeleton. He suggested that the variability in values comparing the facial plane to sella-nasion (SN), the Frankfort horizontal, and the Bolton plane was so small that he was not sure why one was used instead of another to evaluate the face ( Fig 1-7). Downs used the Frankfort horizontal because he felt that the SN and Bolton-nasion planes separated the cranium from the face, whereas the Frankfort horizontal allowed comparison of relationships involving only the face, the structures that orthodontic treatment (with or without surgery) could control. Using four faces, he demonstrated how the facial angle (facial plane to Frankfort horizontal) described the facial type better than the facial plane to SN or the facial plane to the Bolton plane when the position of the mandible was assessed (see Fig 1-7). Contrary to many cephalometric analyses that reported the mean measure, his comparisons for angle of convexity, AB plane, and mandibular plane angle were the numerical differences from the mean of the control group. For example, if the mean angle of convexity of the control group was reported as 180 degrees, a measurement of 185 degrees in a test patient would be reported as +5.0 degrees (a negative difference indicating concave profile and a positive difference indicating convex profile), not 185 degrees. Similarly, deviations from the means of the AB plane or mandibular plane angle were read as the difference from that mean without considering the variation within the control group. Downs thought that these differences would show the difficulty of treating the case. While Downs’s study was small, did not look at sex differences, and used growing individuals for whom some cephalometric values could change with age, his cephalometric parameters and values are still used today. Numerous parameters have been introduced following his study. 15

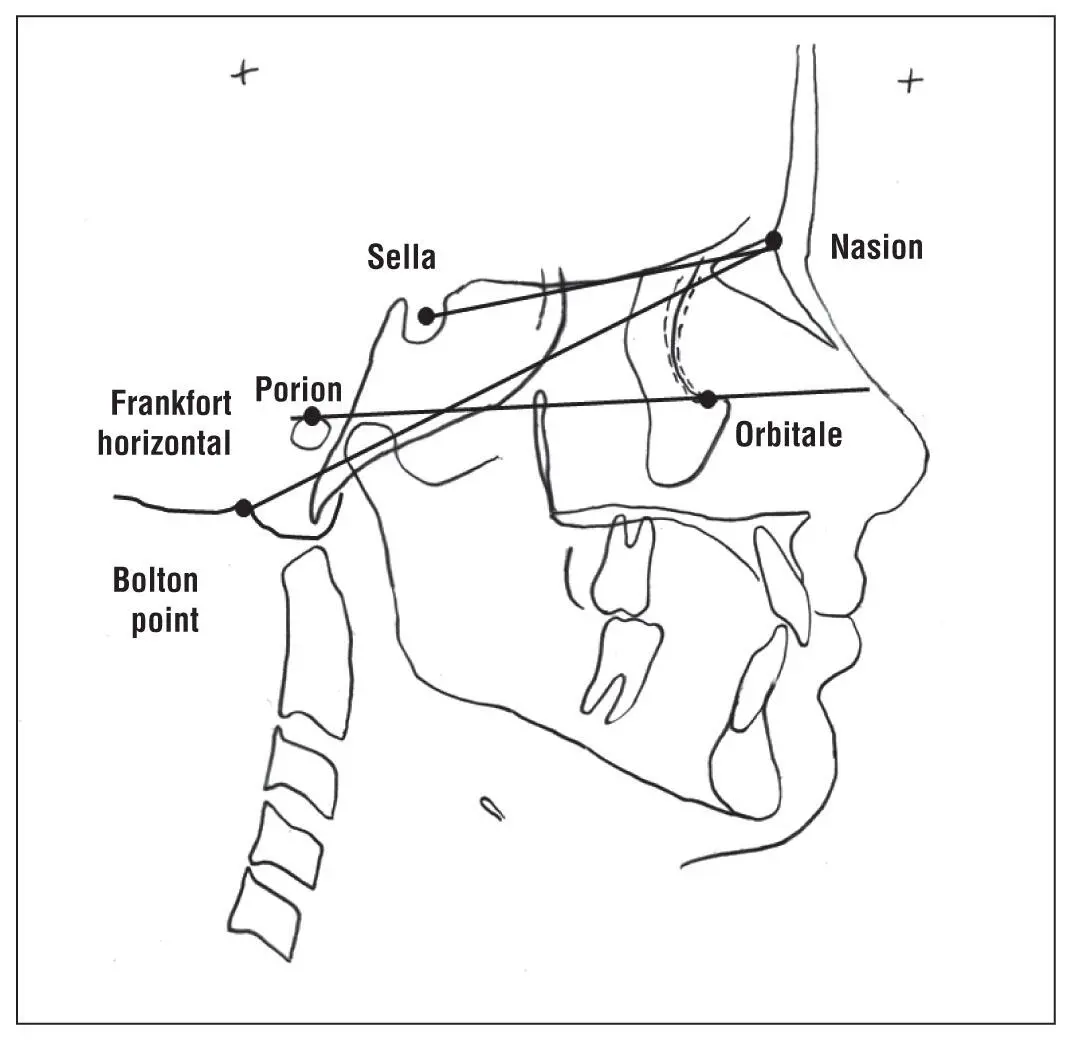
Fig 1-7Sella-nasion, Frankfort horizontal, and Bolton-nasion reference planes.

During the next few decades, numerous studies emphasized the importance of reliable and standardized landmarks, parameters, and references points to determine (1) the outcome of treatment for a single patient, (2) comparison of outcomes from multiple patients undergoing similar treatment, or (3) growth prediction with or without treatment. Unfortunately, some of these landmarks have changed slightly in definition or emphasis through the century of 2D cephalometry and will change for 3D cephalometry because of the addition of the third dimension. For example, in light of 3D investigation, it is currently in question whether A-point can be considered the most forward position of the maxilla. 20

3D CBCTs

3D CBCTs were first reported in 1994 and originally introduced commercially in Europe in 1996. However, it was not until 2001 that the first 3D CBCT was introduced commercially to the United States. Prior to 2007, few articles linked 3D CBCTs to orthodontics. 21Initial concerns about the high radiation dosage and its cost probably limited its use in orthodontics for a while but drove reengineering of the technology, which significantly reduced the radiation exposure and cost. Since 2007, hundreds of articles relating the use of 3D CBCTs to orthodontics have been published. The applicability of 3D CBCTs for associated orthognathic surgery and dental implants as well as need-specific orthodontics (eg, impacted teeth, craniofacial anomalies, bone thickness) has increased their usage in orthodontics and general dentistry. However, significant issues remain, including whether the landmarks and measures used in 2D cephalometry can be used in 3D cephalometry as well as the clinical relevance and use of 3D cephalometry for all orthodontic patients.

The evolution of 3D cephalometry has occurred more quickly than 2D cephalometry, probably due to worldwide digital communications. More than 50 years after the inception of lateral radiographs in orthodontics, Steiner 22indicated that cephalometrics was still not being used for clinical applications but was primarily a tool for academic studies of growth and development. On the other hand, it is predicted that in just 5 years, the global CBCT market will increase from $494.4 million in 2016 to $801.2 million in 2021, although the growth will probably not be limited to orthodontics. 23

Conclusion

Patient care is performed best by educated and discerning clinicians, so it is essential that clinicians not only understand the basics of 2D cephalometry and how it relates to 3D cephalometry but also keep up to date with the evolution of cephalometry and its associated technology, software, and applications.

References

1.American Association of Orthodontists. Clinical Practice Guidelines for Orthodontics and Dentofacial Orthopedics [amended 2014]. https://www.aaoinfo.org/system/files/media/documents/2014%20Cllinical%20Practice%20Guidelines.pdf. Accessed 25 August 2017.

2.Finlay LM. Craniometry and cephalometry: A history prior to the advent of radiography. Angle Orthod 1980;50:312–321.

3.Todd TW. The orthodontic value of research and observation in developmental growth of the face. Angle Orthod 1931;1:67.

4.American Dental Association. History of Dentistry Timeline. http://www.ada.org/en/about-the-ada/ada-history-and-presidents-of-the-ada/ada-history-of-dentistry-time-line. Accessed 25 August 2017.

5.NDT Resource Center. https://www.nde-ed.org/index_flash.htm. Accessed 25 August 2017.

6.Rowland S. Archives of Clinical Skiagraphy. London: Rebman, 1896.

7.Broadbent BH Sr, Broadbent BH Jr, Golden WH. Bolton Standards of Dentofacial Developmental Growth. St Louis: C.V. Mosby, 1975:166.

8.Pacini AJ. Roentgen ray anthropometry of the skull. J Radiol 1922;42: 230–238,322–331,418–426.

9.Basyouni AA, Nanda SR. An Atlas of the Transverse Dimensions of the Face, vol. 37 [Craniofacial Growth Series]. Ann Arbor: University of Michigan Center for Human Growth and Development, 2000:235.

10.Dewey MN, Riesner S. A radiographic study of facial deformity. Int J Orthod 1948;14:261–267.

11.Broadbent BH. A new x-ray technique and its application to orthodontia. Angle Orthod 1931;1:45.

12.Broadbent BH. The orthodontic value of studies in facial growth. In: Physical and Mental Adolescent Growth [The Proceedings of the Conference on Adolescence, 17–18 October 1930, Cleveland, OH].

13.Hofrath H. Die Bedeutung der Rontgenfern und Abstandsaufname für die Diagnostic der Kieferanomalien. Fortschr Orthod 1931;1:232–258.

14.Broadbent BH. Investigations of the orbital plane. Dental Cosmos 1927;69:797–805.

15.Krogman WM, Sassouni V. Syllabus in Roentgenographic Cephalometry. Philadelphia: College Offset, 1957:363.

16.Tweed CH. The Frankfort mandibular incisor angle (FMIA) in orthodontic diagnosis, treatment planning, and prognosis. Angle Orthod 1954;24:121–169.