Douglas C. Montgomery - Introduction to Linear Regression Analysis
Здесь есть возможность читать онлайн «Douglas C. Montgomery - Introduction to Linear Regression Analysis» — ознакомительный отрывок электронной книги совершенно бесплатно, а после прочтения отрывка купить полную версию. В некоторых случаях можно слушать аудио, скачать через торрент в формате fb2 и присутствует краткое содержание. Жанр: unrecognised, на английском языке. Описание произведения, (предисловие) а так же отзывы посетителей доступны на портале библиотеки ЛибКат.
- Название:Introduction to Linear Regression Analysis
- Автор:
- Жанр:
- Год:неизвестен
- ISBN:нет данных
- Рейтинг книги:4 / 5. Голосов: 1
-
Избранное:Добавить в избранное
- Отзывы:
-
Ваша оценка:
Introduction to Linear Regression Analysis: краткое содержание, описание и аннотация
Предлагаем к чтению аннотацию, описание, краткое содержание или предисловие (зависит от того, что написал сам автор книги «Introduction to Linear Regression Analysis»). Если вы не нашли необходимую информацию о книге — напишите в комментариях, мы постараемся отыскать её.
New exercises and data sets New material on generalized regression techniques The inclusion of JMP software in key areas Carefully condensing the text where possible
skillfully blends theory and application in both the conventional and less common uses of regression analysis in today's cutting-edge scientific research. The text equips readers to understand the basic principles needed to apply regression model-building techniques in various fields of study, including engineering, management, and the health sciences.
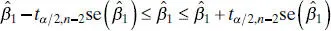
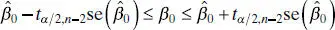
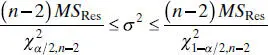
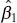 is
is  and t 0.025,18= 2.101. Therefore, from Eq. (2.35), the 95% CI on the slope is
and t 0.025,18= 2.101. Therefore, from Eq. (2.35), the 95% CI on the slope is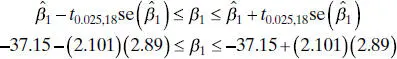

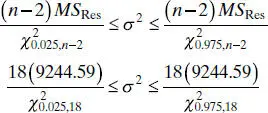
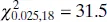 and
and 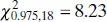 . Therefore, the desired CI becomes
. Therefore, the desired CI becomes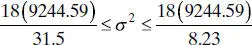

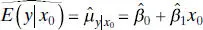
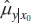 is a normally distributed random variable because it is a linear combination of the observations yi . The variance of
is a normally distributed random variable because it is a linear combination of the observations yi . The variance of 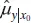 is
is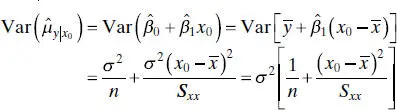
 . Thus, the sampling distribution of
. Thus, the sampling distribution of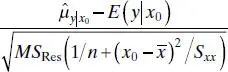
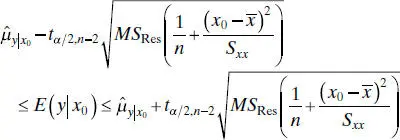
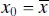 and widens as
and widens as 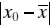 increases. Intuitively this is reasonable, as we would expect our best estimates of y to be made at x values near the center of the data and the precision of estimation to deteriorate as we move to the boundary of the x space.
increases. Intuitively this is reasonable, as we would expect our best estimates of y to be made at x values near the center of the data and the precision of estimation to deteriorate as we move to the boundary of the x space.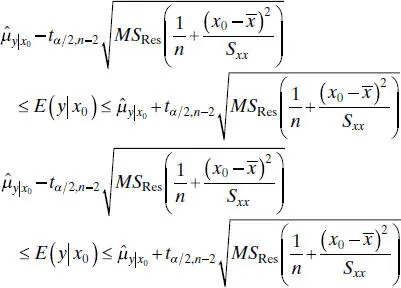
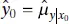 at the value of x 0into this last equation, we will obtain the 95% CI on the mean response at x = x 0. For example, if
at the value of x 0into this last equation, we will obtain the 95% CI on the mean response at x = x 0. For example, if  , then
, then 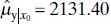 , and the CI becomes
, and the CI becomes









