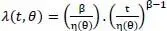The most commonly used mathematical object for modeling failure rate is the Weibull distribution. According to this hypothesis, the latter is defined by:
[1.2] 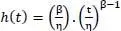
where η is a scale factor (generally time-dependent) and represents typical service life, characterized by the fact that the failure rate is ~ 63.2% (1 – exp(-1)), irrespective of the value taken by the parameter β and therefore of the type of failure.
This modeling is interesting for the following three reasons:
– the mathematical formulation is simple, as it involves a versatile power function (differentiable, integrable, etc.);
– depending on the parameter β, this function is decreasing (β < 1), constant (β = 1) or increasing (β > 1). In other terms, it can represent the three types of previously defined failures;
– the parameter β has a physical significance as it represents the aging dynamics of the observed failure mechanism. Indeed, as already noted, failure instants are characterized by randomness (components tested are assumed to be identical). This means that instead of having a single real value, if failures were purely deterministic, we see a constant dispersion of failure instants. In fact, the parameter β is the image of this dispersion, and the greater it is, the less dispersed the instants of failure are. Ultimately, if β was infinite, all the failure instants would be identical, which is obviously never the case in practice.

Figure 1.2. Fall leaves illustrating aging. For a color version of this figure, see www.iste.co.uk/bayle/maturity1.zip
This figure clearly shows that all of the components – in this case, the leaves – are subject to aging, yet not all of them fail at the same time (not all the leaves have fallen at the instant shown).
As an illustration, let us assume a Weibull distribution whose scale factor is η = 1,000 (this value is a purely conventional value and could be quite different without changing the conclusions obtained). Furthermore, let us assume that there are 30 components in a test and failure instants are generated for each of them in a purely virtual manner for two values of β (3 and 10).
The following figures are obtained, with time on the ordinate (horizontal) axis and the number of components on the abscissa (vertical) axis.
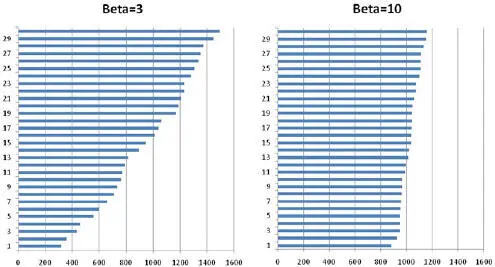
Figure 1.3. Failure instants for β = 3 and β = 10
It can be noted that failure instants are more dispersed for β = 3 (on the left) than for β = 10 (on the right). On the other hand, for β = 1, equation [1.2]is written as:  or, more frequently, as:
or, more frequently, as:
[1.3] 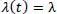
This represents the exponential distribution law modeling catastrophic failures. The failure rate for this category of failures is constant, which means that failure instants do not depend on the elapsed time. This specificity of the exponential law is known as the “memoryless property” (it is the only continuous law with this property). Indeed, returning to the analogy with human beings, a catastrophic failure is, for example, a car accident occurring when a driver cuts off another driver. This “failure” does not depend on the distance traveled, but is due solely to the recklessness of another person. This is entirely different from an aging failure, for which the failure instant directly depends on the distance traveled, because this relates to driver fatigue.
It is important to note that the concept of maturity has no qualitative meaning for non-maintained products. Indeed, the objective of reliability is a probability of success; the mission is achieved by the survival function, which for a Weibull distribution is defined as:
[1.4] 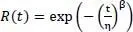
This survival function – and this is the case regardless of the law used – is a strictly decreasing function of time. Therefore, the concept of constant reliability is not applicable. For most non-maintained industrial applications, exponential distribution is preferred to Weibull distribution; this is because the reliability objective is a probability of achieving the mission, whose value is obviously high (generally such that R ∈[90% ; 99%].
In this case, we can return to an exponential distribution because, for these values of the survival function, it is conservative, with respect to a Weibull distribution, whose shape parameter is greater than 1. Indeed, from a mathematical perspective, the ratio of the two survival functions can be calculated as follows:
[1.5] 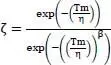
with β > 1 and Tm = mission duration.
To obtain a sufficiently high probability of success in the mission (survival function) requires Tm/η ≪ 1. Consequently, β being greater than 1, 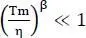 . Using an expansion up to the first order of the exponential function leads to:
. Using an expansion up to the first order of the exponential function leads to:
[1.6] 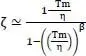
Since Tm/η is greater than 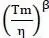 , the numerator is smaller than the denominator and therefore ζ < 1. Hence, the exponential survival function is lower than that of Weibull, which proves that it is conservative.
, the numerator is smaller than the denominator and therefore ζ < 1. Hence, the exponential survival function is lower than that of Weibull, which proves that it is conservative.
Another, more physical way to view this result is to remember that the shape parameter β represents the dispersion of time until failure. The greater β is, the less dispersed the time until failure. Since the Weibull shape parameter is > 1, the corresponding failure instants are less dispersed around the scale parameter η.
Consequently, failures following an exponential distribution with an identical scale parameter occur earlier than those following the Weibull distribution. Therefore, the survival function of the exponential distribution at any instant “t” is weaker than that of the Weibull distribution, which proves the conservative character of this approach.
Temperature is systematically involved in component failure mechanisms. The Arrhenius law is generally used in order to model its effect on the reliability of components. Based on an empirical research method, that is, studied through a number of experiments, the Arrhenius law is used to model the variation in the speed of certain chemical reactions under the influence of temperature. With respect to the previously described Weibull law, the following formulation is obtained:
[1.7] 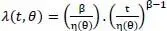
Читать дальше

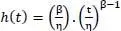


 or, more frequently, as:
or, more frequently, as: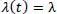
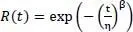
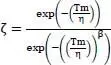
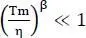 . Using an expansion up to the first order of the exponential function leads to:
. Using an expansion up to the first order of the exponential function leads to: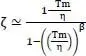
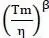 , the numerator is smaller than the denominator and therefore ζ < 1. Hence, the exponential survival function is lower than that of Weibull, which proves that it is conservative.
, the numerator is smaller than the denominator and therefore ζ < 1. Hence, the exponential survival function is lower than that of Weibull, which proves that it is conservative.