The layered nature of CdI 2is emphasised further in a model of polyhedra: CdI 6octahedra link at their edges to form infinite sheets, Fig. 1.39(d), but there are no direct polyhedral linkages between adjacent sheets. A self‐supporting, 3D model of octahedra cannot be made for CdI 2, therefore. Some compounds which have the CdI 2structure are listed in Table 1.16. It occurs mainly in transition metal iodides, bromides, chlorides and hydroxides. TiS 2has the CdI 2structure and was considered as a potential intercalation host cathode for use in lithium batteries (see Section 8.4): Li +ions are able to diffuse into the empty layers that separate adjacent TiS 2sheets at the same time as electrons enter, and migrate through, the 3 d band composed of d xyorbitals on Ti.
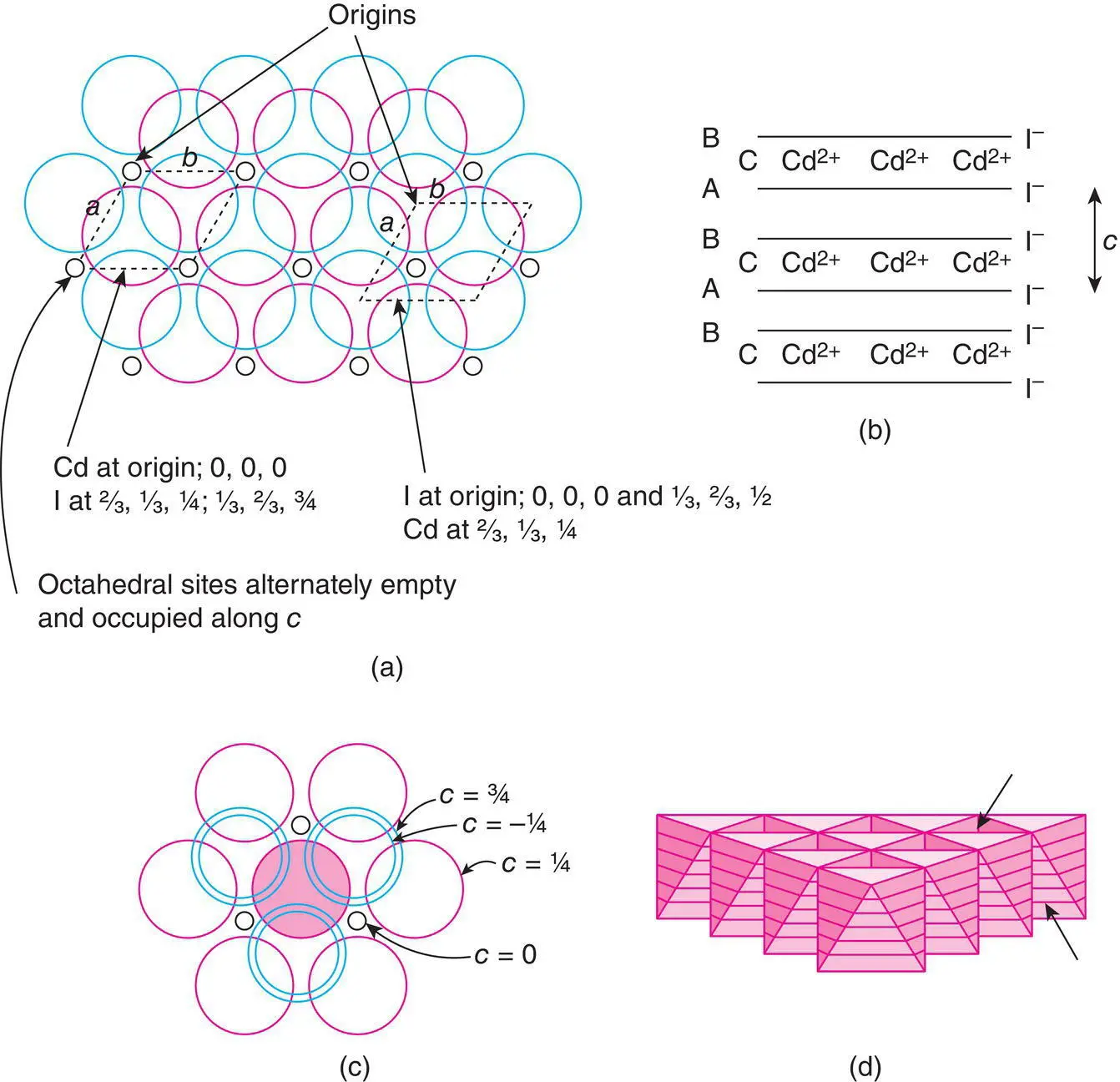
Figure 1.39 The CdI2 structure: (a) the basal plane of the hexagonal unit cell is outlined, with two possible choices of origin; (b) the layer stacking sequence; (c) the coordination environment of I; (d) a layer of close packed octahedra; empty tetrahedral sites are arrowed.
Table 1.16 Some compounds with the CdI2 structure
| Compound |
a /Å |
c /Å |
Compound |
a /Å |
c/ Å |
| CdI 2 |
4.24 |
6.84 |
VBr 2 |
3.768 |
6.180 |
| CaI 2 |
4.48 |
6.96 |
TiBr 2 |
3.629 |
6.492 |
| CoI 2 |
3.96 |
6.65 |
MnBr 2 |
3.82 |
6.19 |
| FeI 2 |
4.04 |
6.75 |
FeBr 2 |
3.74 |
6.17 |
| MgI 2 |
4.14 |
6.88 |
CoBr 2 |
3.68 |
6.12 |
| MnI 2 |
4.16 |
6.82 |
TiCl 2 |
3.561 |
5.875 |
| PbI 2 |
4.555 |
6.977 |
VCl 2 |
3.601 |
5.835 |
| ThI 2 |
4.13 |
7.02 |
Mg(OH) 2 |
3.147 |
4.769 |
| TiI 2 |
4.110 |
6.820 |
Ca(OH) 2 |
3.584 |
4.896 |
| TmI 2 |
4.520 |
6.967 |
Fe(OH) 2 |
3.258 |
4.605 |
| VI 2 |
4.000 |
6.670 |
Co(OH) 2 |
3.173 |
4.640 |
| YbI 2 |
4.503 |
6.972 |
Ni(OH) 2 |
3.117 |
4.595 |
| ZnI 2(l) |
4.25 |
6.54 |
Cd(OH) 2 |
3.48 |
4.67 |
R. W. G. Wyckoff, Crystal Structures , Vols 1 to 6, Wiley (1971).
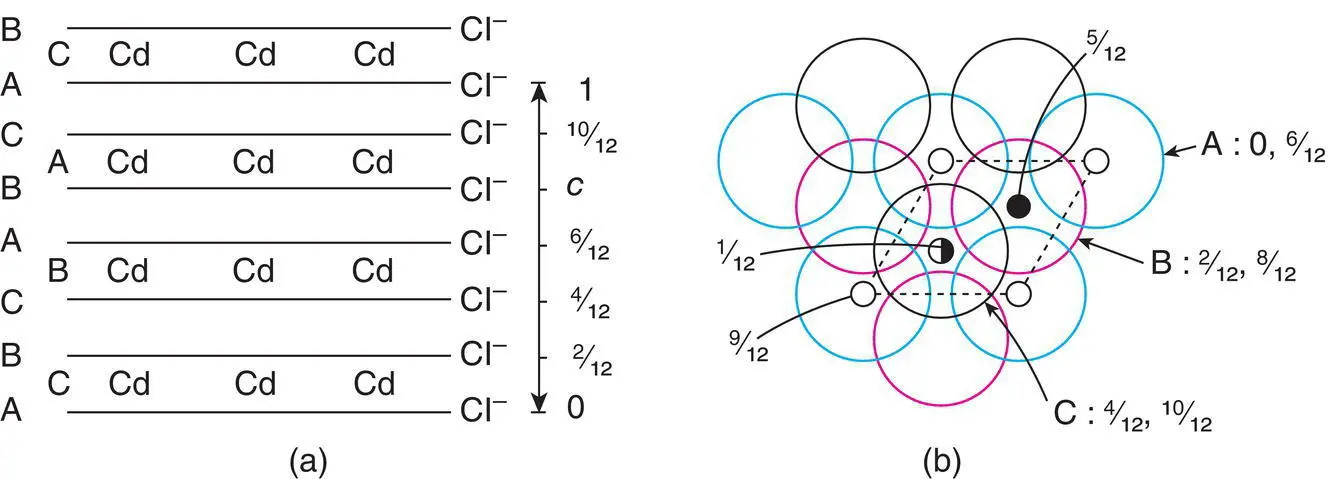
Figure 1.40 The CdCl2 structure.
The CdCl 2structure is closely related to that of CdI 2and differs only in the nature of the anion packing: Cl –ions are ccp in CdCl 2whereas I –is hcp in CdI 2.
The CdCl 2structure may be represented by a hexagonal unit cell, although a smaller rhombohedral cell can also be chosen. The base of the hexagonal cell is of similar size and shape to that in CdI 2but its c axis is three times longer than c in CdI 2. This is because in CdCl 2, the Cd positions, and the CdCl 6octahedra, are staggered along c and give rise to a three‐layer repeat for Cd (CBA) and a six‐layer repeat for Cl (ABCABC), Fig. 1.40. In contrast, in CdI 2, the Cd positions and the CdI 6octahedra are stacked on top of each other and the c repeat contains only two I layers (AB) and one Cd layer (C).
The unit cell of CdCl 2in projection down c is shown in Fig. 1.40(b). Cl layers occur at c = 0 (A), 2/12 (B) and 4/12 (C), and this sequence is repeated at c = 6/12, 8/12 and 10/12. Between those Cl layers at 0 and 2/12, Cd occupies octahedral sites at 1/12. However, the octahedral sites between Cl layers at 2/12 and 4/12 are empty (these sites, at c = 3/12, are directly below Cd at 9/12).
The CdCl 2structure is layered, similarly to CdI 2, and many of the comments made about structure and bonding in CdI 2apply equally well. It also occurs with a variety of transition metal halides (Table 1.17).
The structure of Cs 2O is most unusual as it is anti‐CdCl 2. Cs forms ccp layers and O occupies the octahedral sites between alternate pairs of Cs layers. This raises some interesting questions because Cs is the most electropositive element and Cs salts are usually regarded as highly ionic. However, the structure of Cs 2O clearly shows that Cs is not surrounded by oxygens, as expected for an ionic structure, but has only three O neighbours, all located at one side. The structure is held together, in 3D, by bonding between Cs in adjacent layers.
Table 1.17 Some compounds with the CdCl2 structure
| Compound |
a /Å |
c /Å |
Compound |
a /Å |
c/ Å |
| CdCl 2 |
3.854 |
17.457 |
NiCl 2 |
3.543 |
17.335 |
| CdBr 2 |
3.95 |
18.67 |
NiBr 2 |
3.708 |
18.300 |
| CoCl 2 |
3.544 |
17.430 |
NiI 2 |
3.892 |
19.634 |
| FeCl 2 |
3.579 |
17.536 |
ZnBr 2 |
3.92 |
18.73 |
| MgCl 2 |
3.596 |
17.589 |
ZnI 2 |
4.25 |
21.5 |
| MnCl 2 |
3.686 |
17.470 |
Cs 2O a |
4.256 |
18.99 |
a Cs 2O has an anti‐CdCl 2structure.
R. W. G. Wyckoff, Crystal Structures , Vols 1 to 6, Wiley (1971).
It may be that the structure of Cs 2O does not reflect any peculiar type of bonding but rather that it is the only structural arrangement which is feasible for a compound of this formula and for ions of this size. Thus, from the formula, the coordination numbers of Cs and O must be in the ratio of 1:2; since Cs +is considerably larger than O 2–, the maximum possible coordination number of O by Cs may be six, which then leads to a coordination number of three for Cs.
A related question arises with the structures of the other alkali metal oxides, in particular K 2O and Rb 2O. These are antifluorites with coordination numbers of four and eight for M and O, respectively. These are unusual since Rb is normally far too large to enter into tetrahedral coordination with O. However, if there is no feasible alternative structure, then perhaps Rb has no choice but to enter the tetrahedral sites. With Cs 2O, tetrahedral coordination of Cs by O is probably impossible, hence its structure is anti‐CdCl 2rather than antifluorite. Thermodynamic data qualitatively support these observations; neither Cs 2O nor Rb 2O is very stable: instead, they oxidise readily to give peroxides, M 2O 2, and superoxides, MO 2, which contain much larger anions. Further details of alkali oxides, including suboxides, are given in Chapter 16.
This very important structure type, of general formula ABX 3and typified by SrTiO 3, has a primitive cubic unit cell, shown in Fig. 1.41 as a projection down one axis (a, b) and as an oblique projection (c, d). There are two ways of drawing the unit cell of perovskite. One, (a), contains Ti at the cube corners (coordinates 0, 0, 0), Sr at the body centre 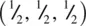 and O at the edge centres
and O at the edge centres 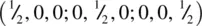 . The other, (b), contains Sr at the corners, Ti at the body centre and O at the face centres. These two descriptions are interchangeable and are simply related by translation of the origin half way along the cube body diagonal. The octahedral coordination environment of Ti is seen in (c, d) and interatomic distances calculated by simple geometry. The bond distance
. The other, (b), contains Sr at the corners, Ti at the body centre and O at the face centres. These two descriptions are interchangeable and are simply related by translation of the origin half way along the cube body diagonal. The octahedral coordination environment of Ti is seen in (c, d) and interatomic distances calculated by simple geometry. The bond distance 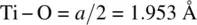 . Sr is equidistant from 12 oxygens and the Sr–O distance equals half the diagonal of any cell face, i.e.
. Sr is equidistant from 12 oxygens and the Sr–O distance equals half the diagonal of any cell face, i.e. 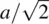 or 2.76 Å.
or 2.76 Å.
Читать дальше



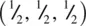 and O at the edge centres
and O at the edge centres 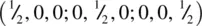 . The other, (b), contains Sr at the corners, Ti at the body centre and O at the face centres. These two descriptions are interchangeable and are simply related by translation of the origin half way along the cube body diagonal. The octahedral coordination environment of Ti is seen in (c, d) and interatomic distances calculated by simple geometry. The bond distance
. The other, (b), contains Sr at the corners, Ti at the body centre and O at the face centres. These two descriptions are interchangeable and are simply related by translation of the origin half way along the cube body diagonal. The octahedral coordination environment of Ti is seen in (c, d) and interatomic distances calculated by simple geometry. The bond distance 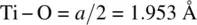 . Sr is equidistant from 12 oxygens and the Sr–O distance equals half the diagonal of any cell face, i.e.
. Sr is equidistant from 12 oxygens and the Sr–O distance equals half the diagonal of any cell face, i.e. 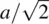 or 2.76 Å.
or 2.76 Å.










