Richard J. Rossi - Applied Biostatistics for the Health Sciences

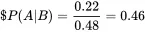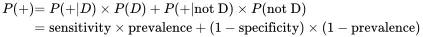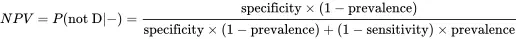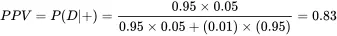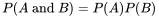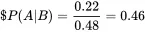
In this example, knowing that event B has occurred increases the probability that event A will occur from 0.35 to 0.46. That is, event A is more likely to occur if it is known that event B has occurred.

Since conditional probabilities are probabilities, the rules associated with conditional probabilities are similar to the rules of probability. In particular,

1 conditional probabilities are always between 0 and 1. That is, 0≤P(A|B)≤1.

2 P(A does not occur|B)=1−P(A|B).

3 P(A or B|C)=P(A|C)+P(B|C)−P(A and B|C).

Conditional probabilities play an important role in the detection of rare diseases and the development of screening tests for diseases. Two important conditional probabilities used in disease detection are the sensitivity and specificity . The sensitivity is defined to be the conditional probability of a positive test for the subpopulation of individuals having the disease (i.e., P(+|D)), and the specificity is defined to be the conditional probability of a negative test for the subpopulation of individuals who do not have the disease (i.e., P(−|not D)). Thus, the sensitivity of a diagnostic test measures the accuracy of the test for a individual having the disease, and the specificity measures the accuracy of the test for individuals who do not have the disease.

The probability that an individual will test positive in a diagnostic test is a weighted average of the proportion of correct diagnoses (i.e., true positives) and the false diagnoses (i.e., false positives). The formula for computing the probability that an individual tests positive is

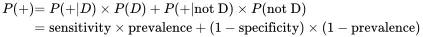
A good diagnostic test for detecting a disease must have high sensitivity and specificity to ensure that it is unlikely that the test will report a false positive or a false negative result. The probability that an individual has the disease given the test is positive is known as the positive predictive value (PPV), and the PPV of a test can be computed from the prevalence of the disease, the sensitivity of the diagnostic test, and the specificity of the test. The formula for the PPV associated with a diagnostic test is

The probability that an individual does not have the disease given the test is negative is known as the negative predictive value (NPV). The formula for computing the NPV is

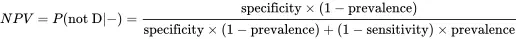
The positive predictive value measures the accuracy of a positive test result and the negative predictive value measures the accuracy of a negative test result. The closer PPV and NPV are to one, the better a diagnostic test is.

Example 2.23

Suppose the prevalence of HIV/AIDs is 5% among a particular population and an Enzyme-Linked Immuno Sorbent Assay (ELISA) test for diagnosing the presence of the HIV/AIDS virus has been developed. If the sensitivity of the ELISA test is 95% and the specificity is 99%, then the probability that an individual has the HIV/AIDS virus given the ELISA test is positive (i.e., PPV) is

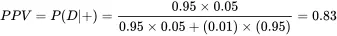
Thus, an individual having a positive ELISA test has a 83% chance of having the HIV/AIDS virus.

The negative predictive value for this ELISA test is

Thus, an individual having a negative ELISA test has a 99.7% chance of not having the HIV/AIDS virus.

Example 2.24

Suppose a new infectious disease has been identified, and the prevalence of this disease is P(D)=0.001. Given that a person has the disease, the probability that the diagnostic test used to diagnose this disease is positive is P(+|D)=0.999, and given that a person does not have the disease, the probability that the test is positive is P(+|not D)=0.01. Then,

1 The sensitivity of the test is 0.999 and the specificity of the test is 0.99.

2 The probability of a positive test result isThus, there is only a 1.1% chance that an individual will test positive for the disease. The reason why a positive test is so rare is that this disease is extremely rare since P(D)=0.001.

3 The positive predictive value associated with this test isThus, there is only a 9% chance that an individual who tests positive for the disease will actually have the disease. This test has very low positive predictive value and is not very reliable for people who test positive.

4 The negative predictive value associated with this test isThus, this test has extremely high negative predictive value and is extremely reliable for people who test negative.

Thus, the probability of having the disease given the test is positive is roughly 100 times the unconditional probability of having the disease. Furthermore, in this example it is still highly unlikely that an individual has the disease even when the test is positive (P(D|+)=0.09, and hence, this is not a very informative diagnostic test.

In some cases, the unconditional probability and the conditional probability will be the same. In this case, knowing that the event B occurred does not change the likelihood that the event A will occur. When the unconditional probability of an event A is the same as the conditional probability of the event A given the event B , the events A and B are said to be independent events .

Two events A and B are independent if and only if one of the following conditions is met:

1 The probability of event A occurring is the same whether B occurred or not. That is,

2 The probability of event B occurring is the same whether A occurred or not. That is,

When two events are not independent, they are said to be dependent events . For example, the event that an individual has lung cancer and the event an individual has smoked a pack of cigarettes a day for several years are dependent events. On the other hand, the event an individual has lung cancer and the event an individual has blonde hair would be expected to be independent events.

If the events A and B are known to be independent, then the probability that A and B both occur is simply the product of their respective probabilities. That is, when A and B are independent

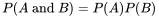
Example 2.25

The genders of successive offspring of human parents are known to be independent events. If the probability of having a male offspring is 0.48, then probability of having