Yves Tille - Sampling and Estimation from Finite Populations
Здесь есть возможность читать онлайн «Yves Tille - Sampling and Estimation from Finite Populations» — ознакомительный отрывок электронной книги совершенно бесплатно, а после прочтения отрывка купить полную версию. В некоторых случаях можно слушать аудио, скачать через торрент в формате fb2 и присутствует краткое содержание. Жанр: unrecognised, на английском языке. Описание произведения, (предисловие) а так же отзывы посетителей доступны на портале библиотеки ЛибКат.
- Название:Sampling and Estimation from Finite Populations
- Автор:
- Жанр:
- Год:неизвестен
- ISBN:нет данных
- Рейтинг книги:4 / 5. Голосов: 1
-
Избранное:Добавить в избранное
- Отзывы:
-
Ваша оценка:
Sampling and Estimation from Finite Populations: краткое содержание, описание и аннотация
Предлагаем к чтению аннотацию, описание, краткое содержание или предисловие (зависит от того, что написал сам автор книги «Sampling and Estimation from Finite Populations»). Если вы не нашли необходимую информацию о книге — напишите в комментариях, мы постараемся отыскать её.
Sampling and Estimation from Finite Populations Provides an up-to-date review of the theory of sampling Discusses the foundation of inference in survey sampling, in particular, the model-based and design-based frameworks Reviews the problems of application of the theory into practice Also deals with the treatment of non sampling errors
is an excellent book for methodologists and researchers in survey agencies and advanced undergraduate and graduate students in social science, statistics, and survey courses.

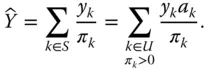
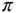 ‐estimator or estimator by dilated values.
‐estimator or estimator by dilated values.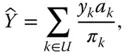
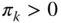 , for all
, for all  If any inclusion probabilities are zero, then
If any inclusion probabilities are zero, then 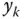 is divided by 0. Of course, if an inclusion probability is zero, the corresponding unit is never selected in the sample.
is divided by 0. Of course, if an inclusion probability is zero, the corresponding unit is never selected in the sample.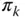 is often denoted by
is often denoted by 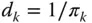 and can be interpreted as the number of units that unit
and can be interpreted as the number of units that unit  represents in the population. The value
represents in the population. The value 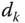 is the weight assigned to unit
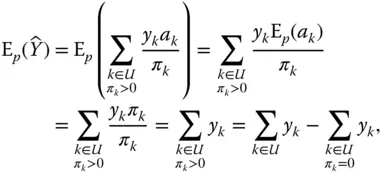
is the weight assigned to unit 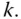 The expansion estimator can also be written as
The expansion estimator can also be written as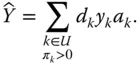
 to be unbiased is that
to be unbiased is that  for all
for all 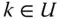 .
.
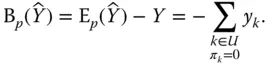
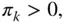 for all
for all 
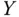 without bias. It is said that the sampling design does not cover the population or is vitiated by a coverage problem. We sometimes hear that a sample is biased, but this terminology should be avoided because bias is a property of an estimator and not of a sample. In what follows, we will consider that all the sampling designs have nonzero first‐order inclusion probabilities.
without bias. It is said that the sampling design does not cover the population or is vitiated by a coverage problem. We sometimes hear that a sample is biased, but this terminology should be avoided because bias is a property of an estimator and not of a sample. In what follows, we will consider that all the sampling designs have nonzero first‐order inclusion probabilities.
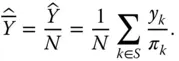
 is unbiased, but it suffers from a serious problem. If the variable of interest
is unbiased, but it suffers from a serious problem. If the variable of interest 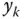 is constant, in other words,
is constant, in other words, 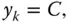 then
then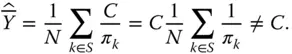
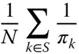
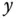 . Even if
. Even if 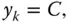
 remains random.
remains random.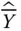 is said to be linearly invariant if, for all
is said to be linearly invariant if, for all 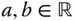 , when
, when 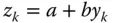 then
then