George Domingo - Semiconductor Basics

At this point, I would like to clarify the concept of bands, which can sometimes be confusing. The bands are not a physical location where electrons reside, just as earth's orbit is not a railroad track or a circular road on top of which the earth travels. A cannonball follows a parabolic path even though there is no “path,” pipe, road, or track that the ball rolls over. The concept of energy bands is similar. The electrons are anywhere in the material, but they have energies with values restricted to certain allowed ranges – energy ranges that we call bands . The electrons are not allowed, under any circumstances, to have energy between the highest energy of the valence band and the lowest energy of the conduction band.

Now consider the situation where the valence band is not full of electrons (case 2), as I show in Figure 2.6, or where the bands expand so much that the conduction band encroaches into the valence band (case 5), as I show in Figure 2.7. These two cases are very similar.

Even at absolute zero (as I show on the left), there is lots of space for the electrons to move. At room temperature, which is quite an increase in energy from absolute zero, the electrons have more than enough energy to move all over the place. It is like having a half‐full garage with two doors at each end connected directly to a freeway, and it is warm enough that people want to get moving. If we apply a voltage (the motivating force) across this material, the electrons have no problem going where they want to go, i.e. to the positive terminal. Also note that the cars that moved up to the freeway left empty spaces in the garage, so some cars in the garage can also move from one location to another inside the parking structure. As you may expect, this is a conductor . Tons of electrons are free to move as soon as a voltage is applied.

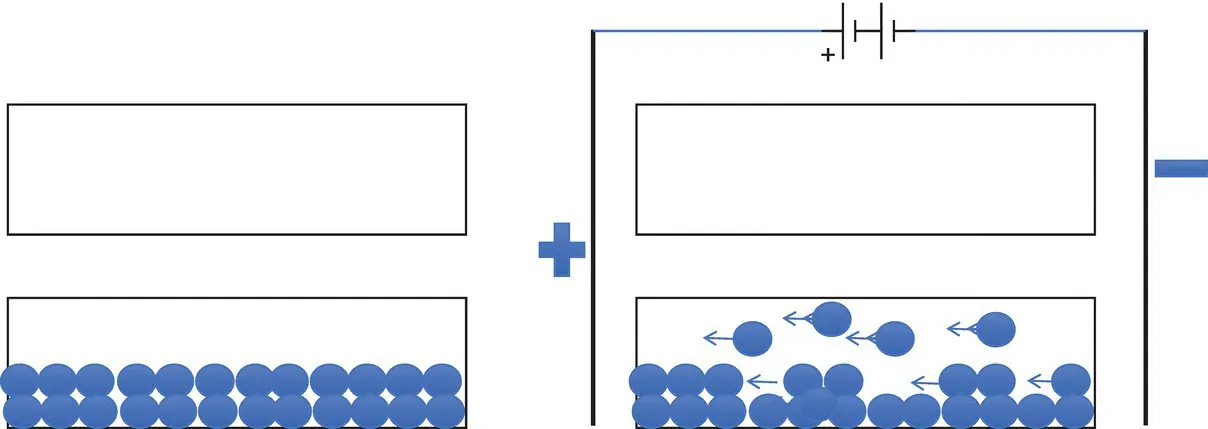
Figure 2.6 If the valence band is not full of electrons, there is a lot of space even at the lowest temperatures for electrons to move easily in the valence band.

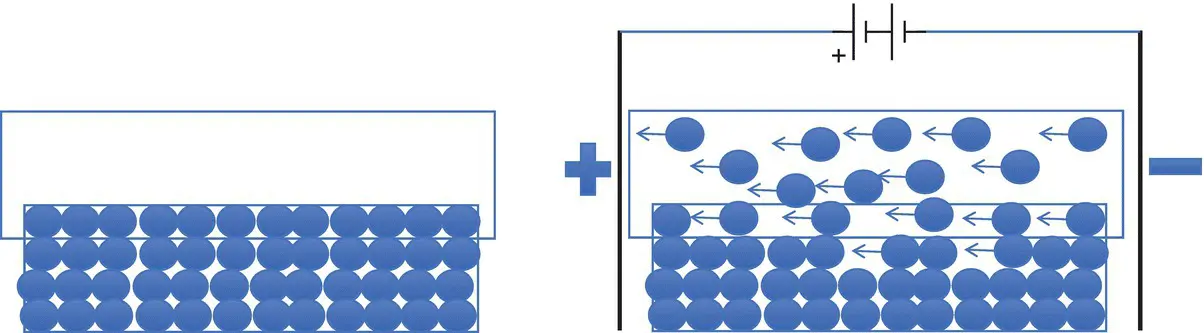
Figure 2.7 Even if the valence band is full, if the conduction band encroaches on the valence band, there is plenty of space for the electrons to move freely when a force (a voltage) is applied.

Now consider the situation where the valence band is full (case 1), but there is a small gap between the conduction and valence bands (case 3). At absolute zero, all the electrons are in the valence band, the lowest allowed energy, and thus the valence band is completely full. But at room temperature, there is enough thermal energy in the system that, statistically, a few electrons can make the jump from the valence band to the conduction band. I show this in Figure 2.8.

In pure silicon, as I said earlier, there are 5 × 10 22silicon atoms per cm 3; but only a tiny number (1.45 × 10 10electrons per cm 3) have, statistically, sufficient energy at room temperature to jump from the valence band to the conduction band. Although 10 10electrons per cm 3looks very large, this is only a single solitary electron out of 3.4 × 10 12silicon atoms (1 out of 3 400 000 000 000) that gain the energy needed to jump to the conduction band. This situation is exemplified in Figure 2.8. Note that as the very few electrons jump to the conduction band, they leave behind an empty spot, which we call a hole . The holes are called p‐particles ( p for positive ) because they appear to have a positive charge. (Remember, each silicon atom is neutral, that is, it has as many protons – positive charges – in the nucleus as it has electrons in the orbits. If you remove one electron, the atom becomes positively charged.) Notice also that in an intrinsic (i.e. perfectly pure) semiconductor, the number of electrons, n i, and the number of holes, p i, have to be exactly the same, since every electron that jumps to the conduction band leaves behind an empty space (a hole) in the valence band. We refer to these intrinsic electrons and holes using the subscript i.

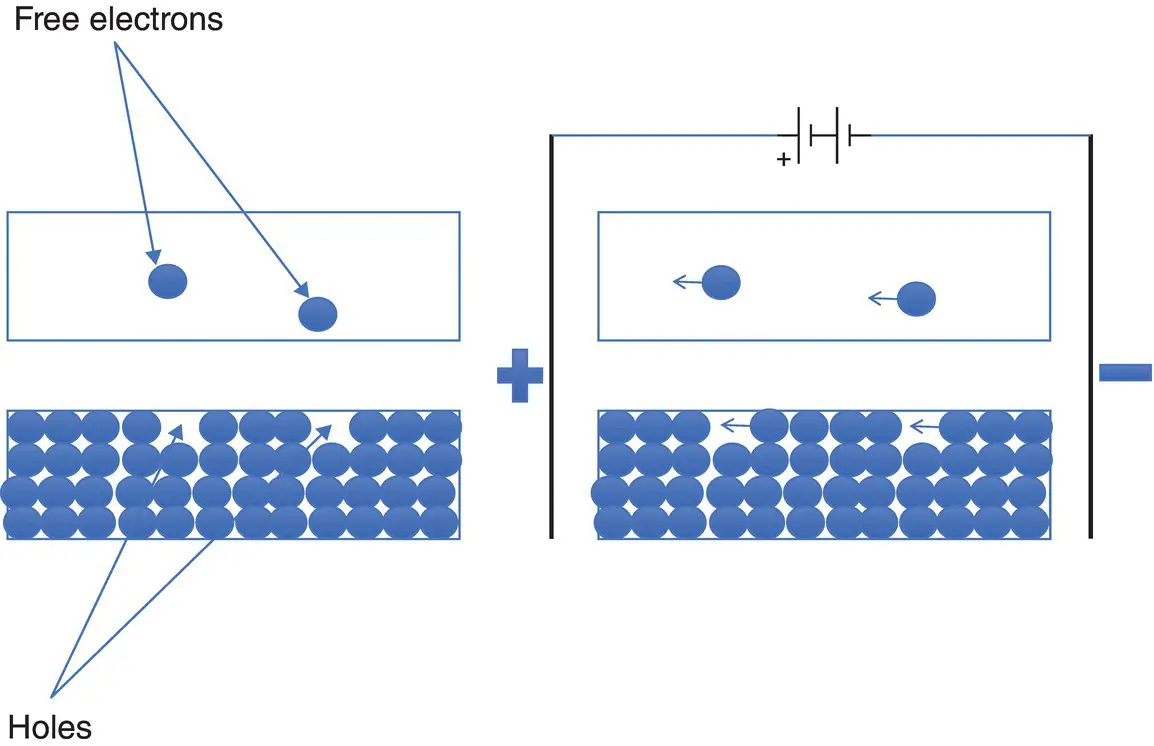
Figure 2.8 The valence band in a semiconductor is completely full, the conduction band is empty, and the separation between the two bands is small, but at room temperature, which is what I show here, there is sufficient energy to kick a few electrons from the valence band to the conduction band, thus generating a small current when we apply a voltage.

Consider what happens when we apply a voltage, as I show on the right in Figure 2.8. Electrons move left toward the positive side: both the electrons in the conduction band and those electrons in the valence band that have an empty space to their left. We like to say, and you will see why later, that the hole (the empty space) is moving in the opposite direction – to the right, toward the negative side – so the hole acts like a positive particle moving to the negative terminal.

Since it is the thermal energy that determines how many electrons can jump to the conduction band and are free to move, and how many holes are left behind, you can expect that the current in a semiconductor is highly dependent on the temperature, that is, the thermal energy. Figure 2.9shows the number of free electrons in silicon and gallium‐arsenide (GaAs) in the conduction band as a function of temperature.

Note that the number of intrinsic electrons and holes in the plot (the vertical y axis) is logarithmic, and the temperature ( T , on the horizontal x ‐axis) is not the temperature but 1000/temperature in absolute units (Kelvin). The actual temperature in degrees Celsius is at the right side of the plot. The advantage of plotting it this way is that the change in the number of free electrons versus 1/ T is very linear. For convenience, I mark the values at room temperature (27 °C), at freezing (0 °C) and at the boiling point of water (100 °C).

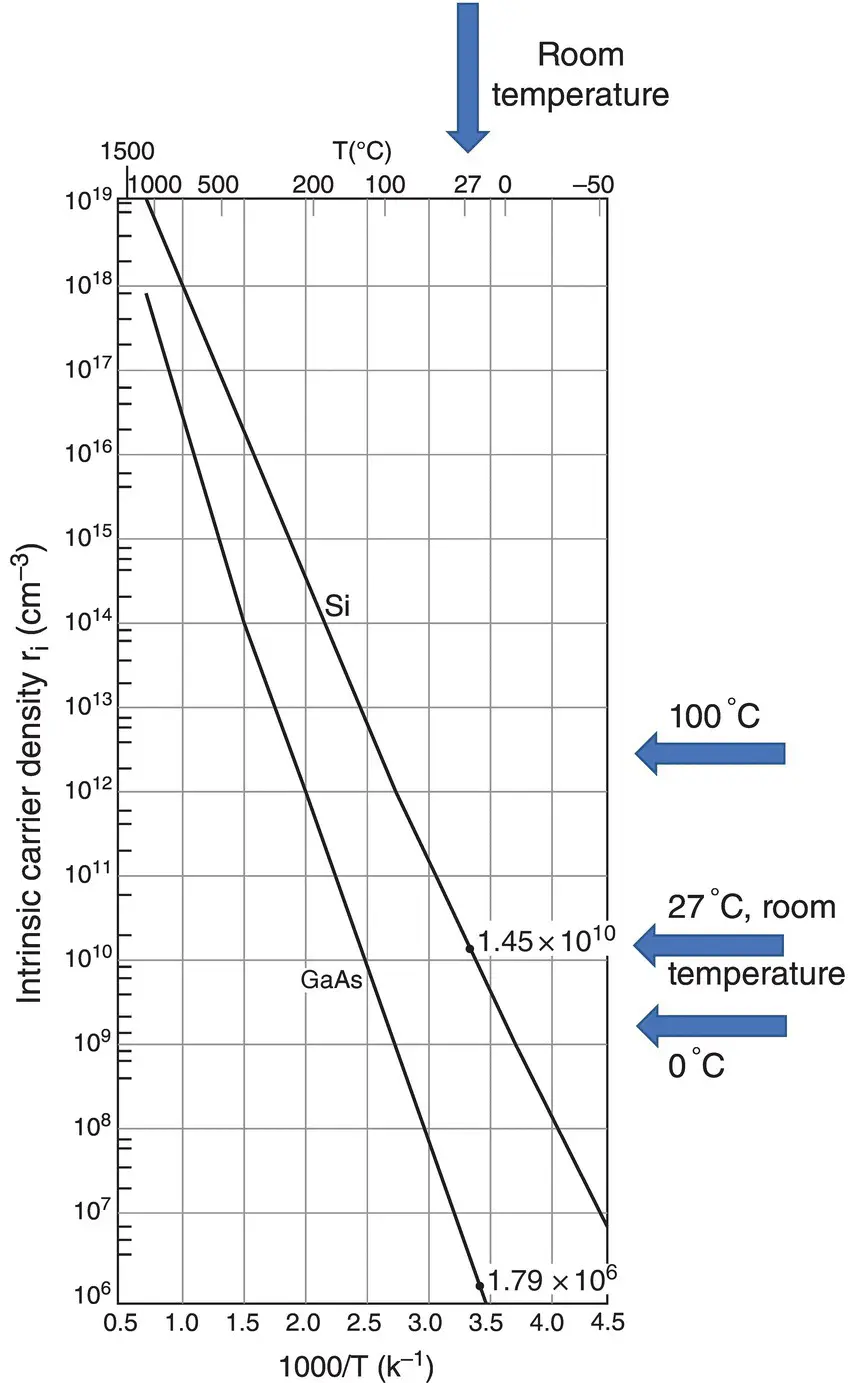
Figure 2.9 Electron and hole concentrations in Si and GaAs change drastically as a function of temperature. Temperature is an indication of the energy of the system, and the more energy in the system, the more electrons can move from the valence band to the conduction band, leaving behind the same number of holes.

The number of free charges is also dependent on the light. Photons can hit the silicon crystal and give their energy to an electron that can use this energy to jump to the conduction band.

Looking at Figure 2.9, at room temperature (27 °C, 300 K), the number of electrons and holes in silicon is 1.45 × 10 10cm −3, as I said earlier; but if we cool it down to 0 °C (the temperature of ice water), the number decreases rapidly to 2 × 10 9cm −3, a factor of 10 lower. If we do the opposite and immerse a semiconductor in boiling water (100 °C), the number of free charges increases to 3 × 10 12cm −3, 300 times larger than at room temperature. As a rule of thumb, the number of free charges in silicon doubles every 7°C.