Myron B. Allen, III - The Mathematics of Fluid Flow Through Porous Media

hyperbolic at any point of the ‐plane where ;

parabolic at any point of the ‐plane where ;

elliptic at any point of the ‐plane where .

Extending this terminology, we say that a first‐order PDE of the form

is hyperbolic at any point where .

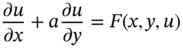
Exercise 1.1 Verify the following classifications, where 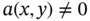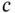and are real‐valued with :

Mathematicians associate the wave equation with time‐dependent processes that exhibit wave‐like behavior, the heat equation with time‐dependent processes that exhibit diffusive behavior, and the Laplace equation with steady‐state processes. These associations arise from applications, some of which this book explores, reinforced by theoretical analyses of the three exemplars in Exercise 1.1. For more information about the classification of PDEs, see [65, Section 2‐6].

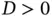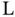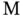In contrast to most texts on pure mathematics, in this book physical dimensionsplay an important role. We adopt the basic physical quantities length, mass, and time, having physical dimensions , , and 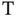, respectively. All other physical quantities encountered in this book—except for one case involving temperature in Chapter 7—are derived quantities, having physical dimensions that are products of powers of 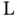, , 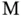and .

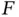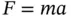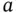For example, the physical dimension of force arises from Newton's second law , where denotes mass and denotes acceleration: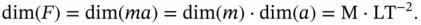

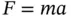Analyzing the physical dimensions of quantities that arise in physical laws can yield surprisingly powerful mathematical results. Subsequent chapters exploit this concept many times.

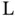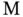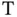Physical laws such as require a way to assign numerical values to the physical quantities involved. We do this by comparison with standards, a process called measurement. For example, to assign a numerical value to the length of an object, we compare it to a length to which we have assigned a numerical value by fiat. A choice of standards for measuring , , and , applied consistently for all occurrences of length, mass, and time, defines a system of units. Changing the system of units typically changes the numerical values that we measure, the exception being dimensionlessquantities, which have dimension 1.

Where practical, this book uses the Système Internationale (SI) as the preferred system of units. The current standards for time, length, and mass in the SI are as follows:

Time: One second (s) is the duration of 9 192 631 770 periods of the radiation emitted by the transition between the two hyperfine levels of the ground state of cesium‐133. This period of time is approximately 1/86 400 of one Earth day.

Length: One meter (m) is the distance traveled in a vacuum by light in 1/299 792 458 s. This distance is approximately times the distance from the Earth's geographic north pole to the equator along a great circle.

Mass: One kilogram (kg) is the mass required to fix the value of the Planck constant as kg , given the definition of one second and 1 m. This mass is approximately that of (1 liter) of water at room temperature and pressure.

In some cases, non‐SI units are more convenient for measuring physical quantities that arise in the bench‐ or field‐scale study of fluid flows in porous media. When these cases arise, we give the factor that enables conversion to SI units. The fact that scientists and engineers prefer non‐SI units in some instances highlights the inherently subjective nature of units: Humans tend to prefer standards that yield numerical values not far from 1 in our everyday experience. One advantage of using dimensionless quantities—a technique employed frequently in this book—is that we avoid this subjectivity.

Three limitations in scope are worth noting. First, we treat only isothermal flows in porous media, that is, flows at constant temperature. This restriction conveniently allows us to ignore the energy balance equation in deriving governing PDEs. On the other hand, it also eliminates several types of flows that have important applications, including flows in geothermal reservoirs and thermal methods of enhanced oil recovery, such as steam flooding.

Also glaringly absent from the table of contents is the topic of flows in fractured porous media. Geoscientists correctly point out that most geologic porous media possess fractures, which exert significant influences on fluid flows. Yet the mathematics of flow in fractured porous media remains poorly delineated, owing not so much to the absence of mathematical models (see [21] for a recent overview and [8, 15, 86, 153] for prominent examples) but, more importantly, to the observation that fractures exist at many scales of observation. In some underground formations, one must know something about the geometry of individual fractures to model fluid flows accurately. In these settings, the modeler's challenge is to represent the discrete fracture system (or statistical realizations) on tractably coarse computational grids. In other geologic settings, it suffices to treat the pore network and the fracture network as overlapping porosity systems, and the challenge is to model how fluids move within and between them. This spectrum of modeling approaches deserves a monograph of its own.