Mantle Convection and Surface Expressions

In Handy’s formulation the strength of a two‐phase mixture depends not only on the rheological properties of the individual phases but also on the microstructure and phase proportions. In Handy’s formalism bounds can be place on the mechanical behavior of a two phase aggregate as the bulk properties lie between two end members. These end members are based on the degree of interconnectivity of the weaker or the stronger phase. If the weak phase is interconnected, the microstructure is termed an interconnected weak layer (IWL) ( Figure 2.6a), and if the strong phase is connected, it is termed a load‐bearing framework (LBF) ( Figure 2.6b).

In the case of an IWL microstructure, the aggregate approaches an iso‐stress state. That is to say that both phases experience similar stress levels but strain at very different rates. For a pure iso‐stress condition, the soft phase modulates the stress levels and strain is partitioned into the weak phase ( Figure 2.6c). Thus, the bulk properties of the aggregate approach those of the softer phase. In cases where yield strength contrast is large, stress levels in the hard phase may be too low to result in yielding as stress levels in the strong phase are limited by yielding of the soft phase. Although IWL approaches that of an iso‐stress state, these are not identical and there are several important differences. For IWL deformation, if the aggregate is composed of small to moderate amounts of the weak phase and/or the strong phase has a significantly higher stress exponent than the weak phase, then the weak phase will deform at higher stresses and strain rates than the iso‐stress condition and the stronger phase will deform at lower stress and strain rate than the iso‐stress condition. However, if there are large proportions of the softer phase and/or the stress exponent of the harder phase is similar or smaller than the soft phase, then stress levels in the harder phase will exceed the soft phase.

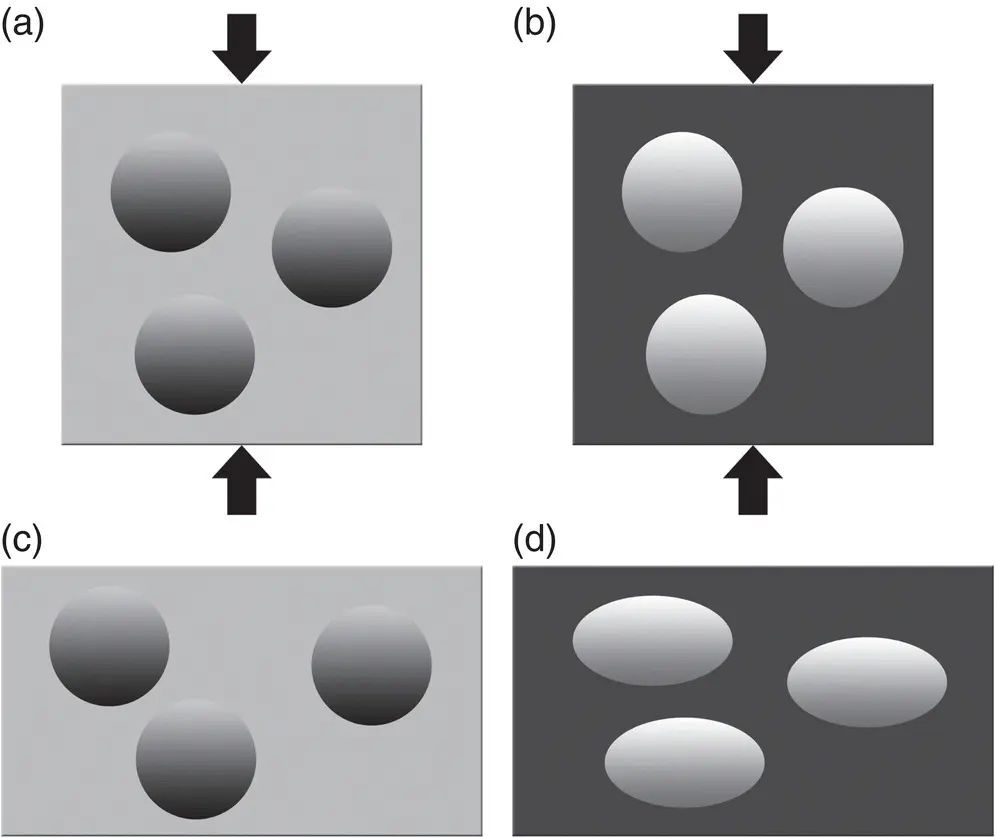
Figure 2.6 Schematic of (a) interconnected weak layer and (b) load‐bearing framework microstructures. Light gray indicates the weaker phase and dark gray/black indicates the stronger phase. During deformation of the interconnected weak layer microstructure (c), strain is partitioned into the weaker phase and strong inclusions remain relatively undeformed. Stresses in the two phases will be similar but large strain heterogenaity is induced in the weak phase as the weaker material must flow around the rigid inclusions. During deformation of the load‐bearing framework microstructure (d) strain is distributed ~homogeneously as the strong matrix imposes its strain and strain rate on the weaker inclusions. Stresses will be lower in the weaker phase as it flows at lower stress than the stronger matrix.

In contrast, when a LBF microstructure exists, the soft phase is not interconnected and the rheology of the aggregate is controlled by the harder phase. Stress is supported primarily by the stronger phase and the stronger phase imposes its strain rate on the softer phase ( Figure 2.6d). In this case, stress levels in the two phases diverge. Although local heterogeneous strain rate occurs at phase boundaries, the aggregate as a whole behaves in a manner very close uniform strain rate in the two phases.

Determining conditions where IWL versus LBF are stable depends on phase proportions, strength contrast and total strain. Strength contrast will be determined by the contrast in parameters in the flow laws for the phases (e.g., activation energy and volume and stress exponent). Larger strength contrasts between the phases and a lower volume fraction of the softer phase promotes stability of a LBF. Generally speaking IWL dominates when the volume fraction of the weak phase exceeds ~30–40% (i.e., percolation threshold). However, large strains also tend to favor IWL microstructures as the weaker inclusions coalesce to form interconnected microstructures resulting in a transition from LBF to IWL behavior (Handy, 1990, 1994).

Given the discussion above, the difficulty in interpreting stress data from high pressure experiments on polyphase materials becomes clear. Unless one knows if a material deforms in an IWL or LBF style, interpretation of stresses measured in individual phases becomes problematic. In high‐pressure experiments that use lattice strain to calculate stresses, the stresses are measured in the individual phases and there is not currently a technique that is used to independently measure the bulk strength of the material. Looking at the two deformation experiments on Brg and Fp that are shown in Figures 2.2and 2.3, a large discrepancy is observed. Miyagi & Wenk (2016) find room‐temperature strength of Brg to be ~1.4 times that of Fp, while in contrast Girard et al. (2016) find Brg to be about four times stronger. Notable differences exist between these two experiments, primarily that the work of Girard et al. (2016) is performed at high temperature and under steady‐state conditions. Computations by Kraych et al. (2016) find the strength contrast to be ~4 at 30 GPa and room temperature, but find that this decreases at high temperatures. Although the calculated strength of Brg in this study is similar to those of experiments at room temperature and high temperature, the strength contrast trend is inconsistent with the two‐phase experiments. However, in the experiments this may be due to microstructure and the effect of IWL versus LBF‐style deformation. Miyagi & Wenk (2016) did not recover samples for microstructural analysis; however, the larger sample in Girard et al. (2016) is amenable to documentation of microstructure. Microstructural analysis from this experiment seems to indicate that although Brg appears to be interconnected, as would be expected for LBF behavior, strain seems to be largely partitioned into the softer Fp, which is expected for IWL behavior. Based on the description of Handy, an LBF should result in large differences in stresses measured in each phase whereas the stresses measured in each phase for IWL should be close. One possibility for this discrepancy is that the samples in Girard et al. (2016) are transitioning between LBF and IWL, and if this is the case this transition would occur at strains greater than 60–70%. Indeed, this study concluded that at high strains shear weakening and strain localization is expected to occur. In contrast to Girard et al. (2016), a study on 72% CaGeO 3Pv + 28% MgO as an analog for Brg + Fp found a strength contrast of ~2 and found that the stresses in CaGeO 3in the two‐phase experiments were higher than in single‐phase experiments. Based on this, it was concluded that a LBF behavior dominates (Y. Wang et al., 2013). Another analog study on NaMgF 3Pv + NaCl analogs with a strength contrast of ~10 found systematically lower stresses in NaMgF 3with the addition of 15‐70% NaCl (Kaercher et al., 2016). In contrast to NaMgF 3, stress levels remained ~ constant in NaCl independent of volume fraction. Deformation behavior of samples with volume fractions of NaCl > 50% were consistent with an IWL. Samples with less than 50% NaCl were not consistent with either an IWL or an LBF and it was concluded that the behavior of the samples were somewhere intermediate to IWL and LBF.

There are several reasons why high‐pressure experiments may not match stress levels expected for either IWL or LBF microstructures. IWL and LBF are bounds on stress and strain partitioning, so it is not surprising that experiments may deviate from these two end‐member behaviors. In Handy’s formulation, constant volume deformation is assumed, and this may not necessarily be the case for high pressure (and high temperature) experiments, particularly if pressure or temperature changes occur during deformation, such as in DAC experiments and to a lesser extent in large volume deformation. Although nonlinearity of deformation is accounted for, the behavior predicted by Handy (1990, 1994) assumes plastically isotropic phases. This is not strictly true for most minerals particularly those deforming via dislocations. Finally, given that the percolation threshold for a phase is ~30–40% by volume, there is a range of phase proportions where interconnectedness of both phases occurs. Although simultaneous interconnectedness of phases may not be stable to higher strains, it may be stable over relatively small strains experienced in experiments, and one would expect that this would result in stress and strain partitioning that is intermediate to IWL and LBF end members.