Paromita Mazumdar - Manual of Laboratory Testing Methods for Dental Restorative Materials

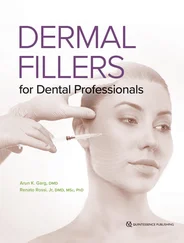
Figure 1.2 Tooth sample testing.

When a body is subjected to axial forces in a straight line and in opposite directions, it results in tension. The resistance of the material to this load is called tensile strength. The length alteration that results from the application of a tensile force on a body before its rupture is defined as elongation. Nominal value of tensile strength is determined by the equation of load and cross‐sectional area (Kgf/cm 2). Values of stress–strain determine a curve, characterizing the performance of the material under tensile test. From this curve, elastic modulus, ultimate tensile strength, resilience and toughness of such product can be registered [7]. Tensile testing is normally applied to materials which are placed under loading that is generally applied in different directions, as the opposing cusps move over the restoration surface. Loads that stretch or elongate a material cause tensile stresses (as shown in Figures 1.3– 1.5).

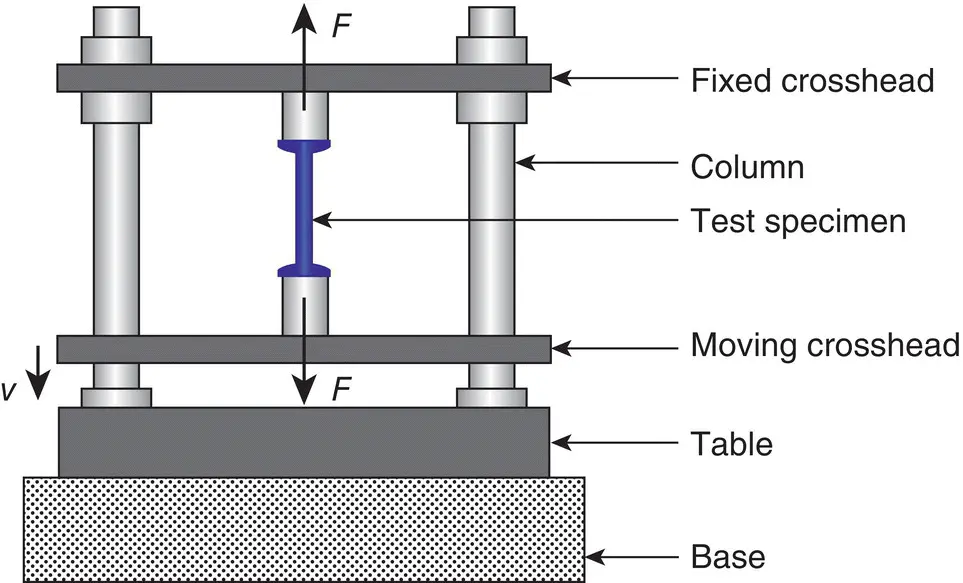
Figure 1.3 Tensile strength assessment.

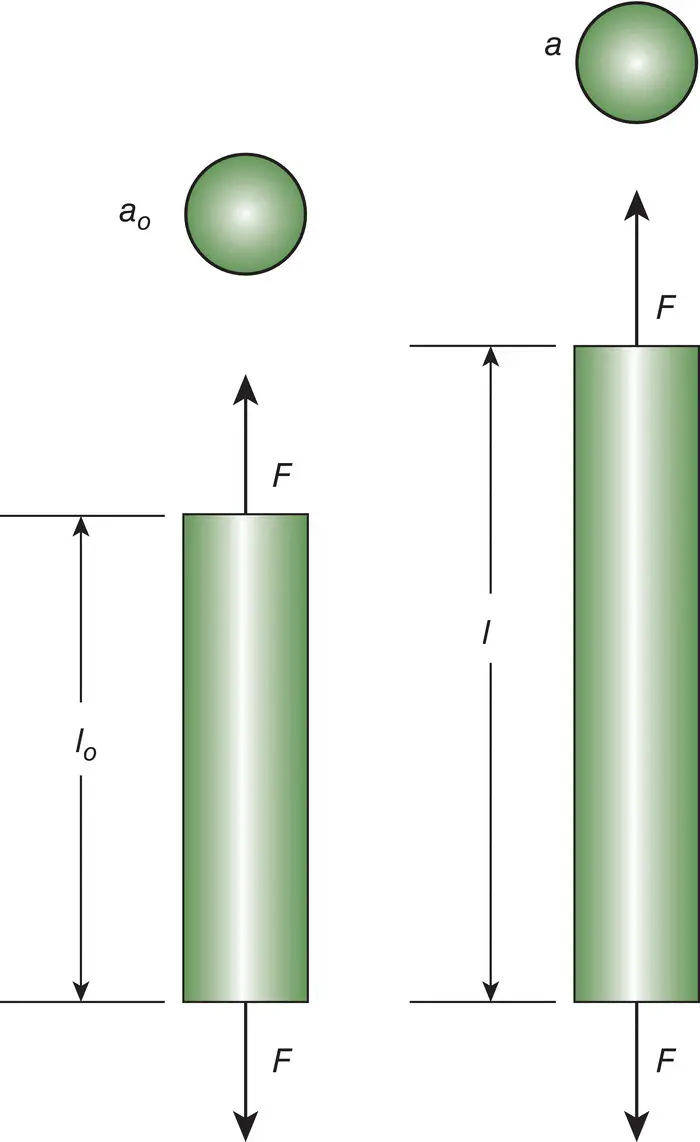
Figure 1.4 Tensile strength assessment.

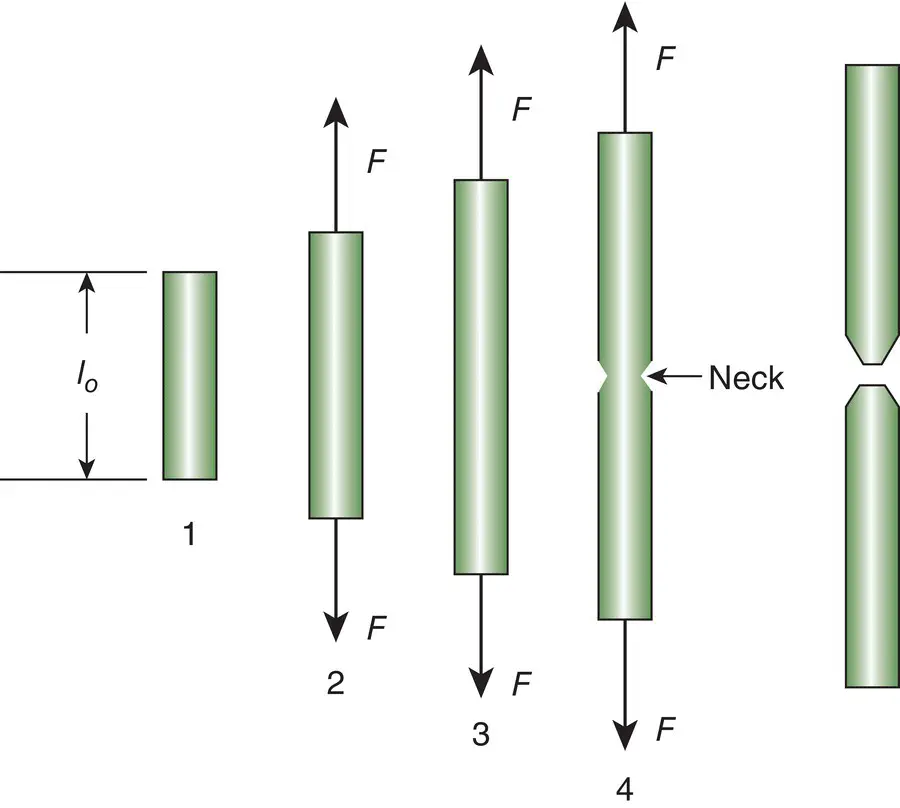
Figure 1.5 1, No load; 2, uniform elongation; 3, maximum load; 4, necking.

For a DTS test, the fracture line doesn't always occur along the central line, thus giving false results and making the test inaccurate.

The diametral tensile strength (DTS) test is useful for materials that exhibit very limited plastic deformation and where information regarding stretching or elongation resistance is required. The DTS is a property described by American Dental Association (ADA)/American National Standards Institute (ANSI) Specification 27 for characterizing dental composite restoratives (DCR). It represents the minimal stress that a body will withstand without rupture when tensile loads are applied. The DTS test is considered useful because masticatory forces are frequently applied obliquely and tend to create tensile stress. A UTM is generally used to determine the tensile strength of a material. Materials which plastically deform would produce erroneous DTS values and also would be expected to display strain rate sensitivity. Composite resins are subjected to complex intraoral forces during mastication and parafunctional habits. Of the three tests used in this study to replicate intraoral forces, diametral tensile is the most difficult to interpret. Failure must occur in the center of the specimen along the diameter due to tensile forces if the diametral test is to yield useful results. The method was used to evaluate the influence of different cross‐head speeds on DTS of a resin composite material (Tetric N‐Ceram) by Anubhav Sood et al. in 2015 where they found that the cross‐head speed variations did not have a significant effect on the DTS of the resin composite [8].

Rupture under low tension characterizes fragile materials, susceptible to brittleness. In these cases, tensile strength is not indicated to evaluate material reaction, because of the low cohesive condition. An alternative method of tensile strength is calculated by compressive testing. It is a relatively simple and reproducible test. It is defined as diametral compression test for tension or indirect tension. Disk sample is necessary to conduct this test, where it is compressed diametrically introducing tensile stress in the material in the plane of the force application by the test. This is calculated by the formula: 2 P /π × D × T , where: P = load applied, D = diameter of the disk, T = thickness of the disk, π = constant [9, 10] (as shown in Figure 1.6).

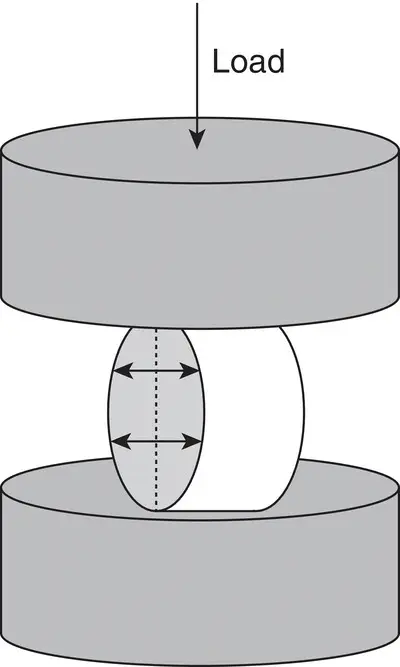
Figure 1.6 Diametral compressive strength assessment.

Source: Cefaly [9], Cattani‐Lorente [10].

Compressive testing is normally applied to materials that are expected to be placed in situations of occlusal loading. Since most of mastication forces are compressive in nature, it is important to investigate materials under this condition. To test compressive strength of a material, two axial sets of force are applied to a sample in an opposite direction, in order to approximate the molecular structure of the material. Here, cylindrical‐shaped specimens are tested. The dimensions of the samples should have a relation of length to diameter of 2 : 1. When this proportion is exceeded, it can result in undesirable bending of the specimen. In the same manner of tensile strength, nominal value of compressive strength also is determined by the reason of load by cross‐sectional area (Kgf/cm 2). Stress–strain curve of investigated material is determined in the same manner as established to tensile tests. Thus, the elastic modulus can also be determined by the stress–strain ratio in the elastic region [11] (as shown in Figures 1.7and 1.8).

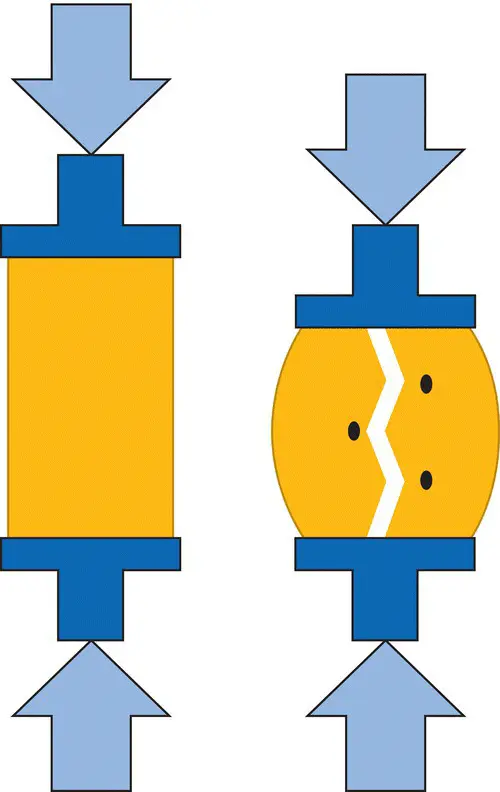
Figure 1.7 Direction of force is perpendicular to the object.

The flexural strength of a material is its ability to bend before it breaks. It is obtained when the ultimate flexibility of one material is achieved before its proportional limit. This is a measure of the strength of a beam of restorative material supported at each end and subjected to a static load. Stresses on the upper surface of the beam tend to be compressive, whilst those on the lower surface are tensile. This test may be considered to combine elements of tensile and compressive testing. Flexural forces are the result of forces generated in clinical situations and the dental materials need to withstand repeated flexing, bending, and twisting. A high flexural strength is desired once these materials are under the action of chewing stress that might induce permanent deformation. To evaluate flexural strength of a dental material, bar‐shaped specimens with dimension of 25 mm in length,2 mm in width and 2 mm in height (ISO 9917 – 212) are generally used. Specimens are placed on two supports and a load is applied at the center. This test is known as three‐point bending test. The load at yield is the sample material's flexural strength that is calculated by the following formula:

(1.1)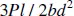

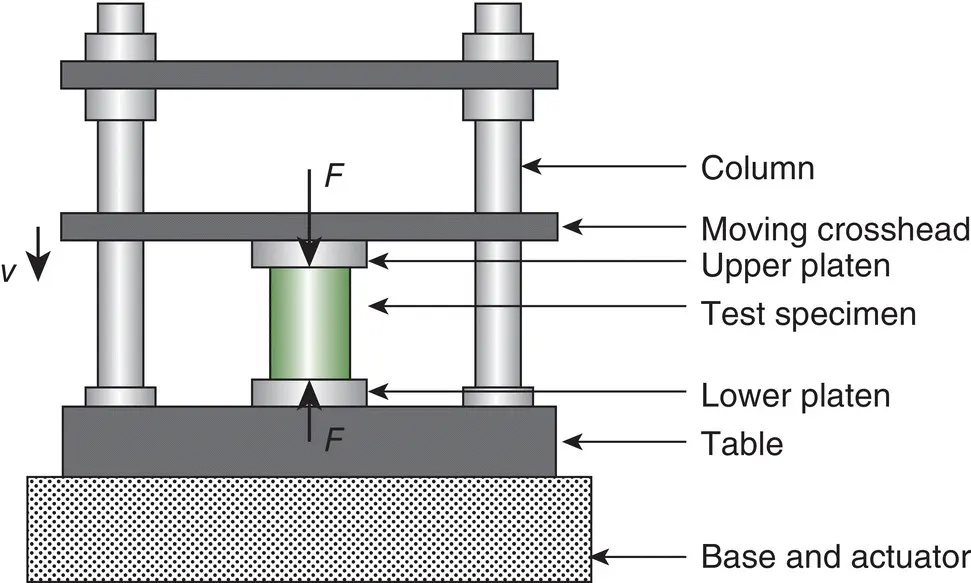
Figure 1.8 Schematic representation of the set‐up for compressive strength.