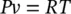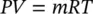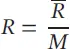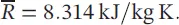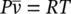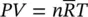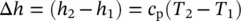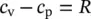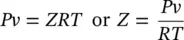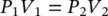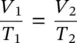1 ...8 9 10 12 13 14 ...27 where, s liq,vap= s vap− s liq.
A pure substance is defined as the one that has a homogeneous and invariable chemical composition. Despite having the same chemical composition throughout, it may be in more than one phase, namely, liquid, a mixture of liquid and vapor (e.g. steam), and a mixture of solid and liquid. Each phase has the same chemical composition. However, a mixture of liquid air and gaseous air cannot be considered a pure substance because the composition of each phase differs from that of the other. A thorough understanding of the pure substance is of significance, particularly for TES applications. Thermodynamic properties of water and steam can be obtained from tables and charts that are present in most thermodynamics books, based on experimental data or real‐gas equations of state, or obtained through computer calculations. It is important to note that the properties of low‐pressure water are of great significance in TES systems for cooling applications, since water vapor existing in the atmosphere typically exerts a pressure less than 1 psi (6.9 kPa). At such low pressures, it is known that water vapor exhibits ideal‐gas behavior.
In many practical thermodynamic calculations, gases such as air and hydrogen can often be treated as ideal gases, particularly for temperatures much higher than their critical temperatures and for pressures much lower than their saturation pressures at given temperatures. An ideal gas can be described in terms of three parameters: the volume it occupies, the pressure it exerts, and its temperature. In fact, all gases or vapors, including water vapor, at very low pressures exhibit ideal‐gas behavior. The practical advantage of treating real gases as ideal is that a simple equation of state with only one constant can be applied in the following form:
(1.16) 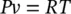
and
(1.17) 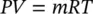
The ideal‐gas equation of state was originally established from experimental observations, and is also called a P – v – T relationship for gases. It is generally considered as a concept rather than a reality. It requires only a few data values to define a particular gas over a wide range of its possible thermodynamic equilibrium states.
The gas constant R is different for each gas depending on its molecular weight M :
(1.18) 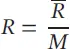
where, 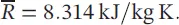
Equations (1.17)and (1.18)may be written on a mole‐basis as follows:
(1.19) 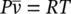
and
(1.20) 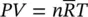
The other simplifying feature of ideal‐gas behavior is that, if assumed that the constant‐pressure and constant‐volume specific heats are constant, changes in specific internal energy and specific enthalpy can be calculated simply without referring to thermodynamic tables and graphs from the following expressions:
(1.21) 
and
(1.22) 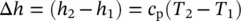
The following is another useful relation for ideal gases obtained from the expression, h = u + Pv = u + RT :
(1.23) 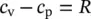
For the entire range of states, the ideal‐gas model may be found unsatisfactory. Therefore, the compressibility factor ( Z ) is introduced to measure the deviation of a real substance from the ideal‐gas equation of state. The compressibility factor is defined by the relation:
(1.24) 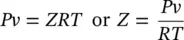
Figure 1.4shows a generalized compressibility chart for simple substances. In the chart, we have two important parameters: the reduced temperature ( T r= T / T c) and the reduced pressure ( P r= P / P c). To calculate the compressibility factor, the values of T rand P rshould be calculated using the critical temperature and pressure values of the respective substance, which can easily be obtained from thermodynamics books. As can be seen in Figure 1.4, at all temperatures, Z → 1 as P r→ 0. This means that the behavior of the actual gas closely approaches ideal‐gas behavior, as the pressure approaches zero. For real gases, Z takes on values between 0 and 1. If Z = 1, Eq. (1.24)becomes Eq. (1.16). In the literature, there are also several equations of state for accurately representing the P – v – T behavior of a gas over the entire superheated vapor region, for example, the Benedict–Webb–Rubin equation, the van der Waals equation, and the Redlich and Kwong equation. However, some of these equations of state are complicated due to the number of empirical constants they contain, and are more conveniently used with computer software to obtain results.
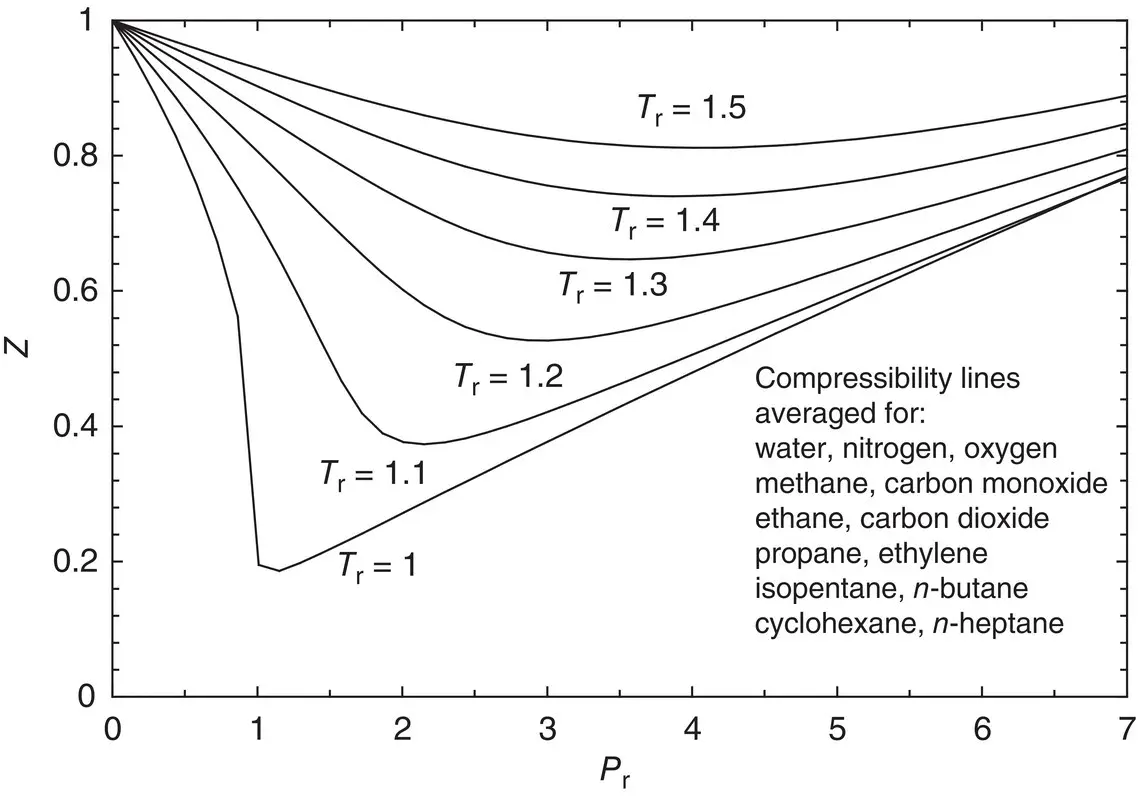
Figure 1.4 A generalized compressibility chart obtained for 13 fluids (generated through engineering equation solver [EES] software which is used for Z prediction with real gas equations of state). See Ref. [2] for details.
There are some special cases if either one of P , v , and T is constant. At a fixed temperature, the volume of a given quantity of ideal gas varies inversely with the pressure exerted on it (in some books, this is called Boyle's law ), describing compression as
(1.25) 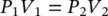
where the subscripts refer to the initial and final states.
Equation (1.25)is employed by designers in a variety of situations: when selecting an air compressor, for calculating the consumption of compressed air in reciprocating air cylinders, and for determining the length of time required for storing air. Nevertheless, use of Eq. (1.25)may not always be practical due to temperature changes. If temperature increases with compression, the volume of a gas varies directly with its absolute temperature in K as:
(1.26) 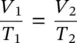
Читать дальше