Joseph R. Badick - Flight Theory and Aerodynamics

The air approaching the leading edge of an airfoil is first slowed down and then speeds up again as it passes over or beneath the airfoil. Figure 3.19compares two local velocities with the flight path velocity V 1and with each other. As the velocity changes, so does the dynamic pressure “ q ” and, according to Bernoulli’s principle, so does the static pressure “ P .” Air near the stagnation point at the leading edge of the wing has slowed down, so the static pressure in this region is higher than the ambient static pressure. Air that is passing above and below the airfoil, and thus has speeded up to a value higher than the flight path velocity (both V 2and V 3are greater than V 1), will produce static pressures that are lower than ambient static pressure. So as “ q ” increases, “ P ” static pressure decreases and a greater pressure differential is realized.

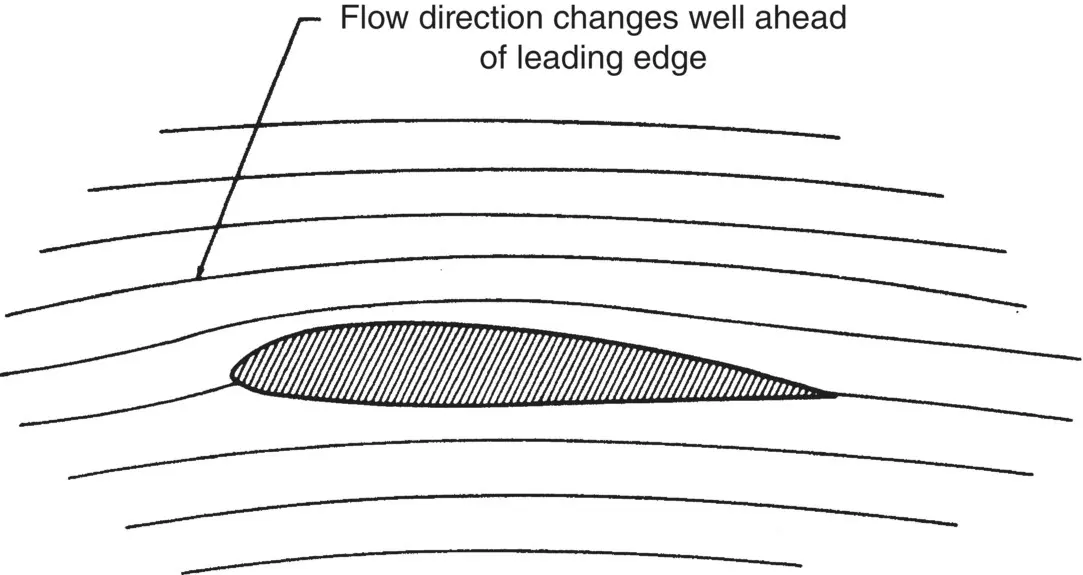
Figure 3.18 Effect of pressure disturbances on airflow around an airfoil.

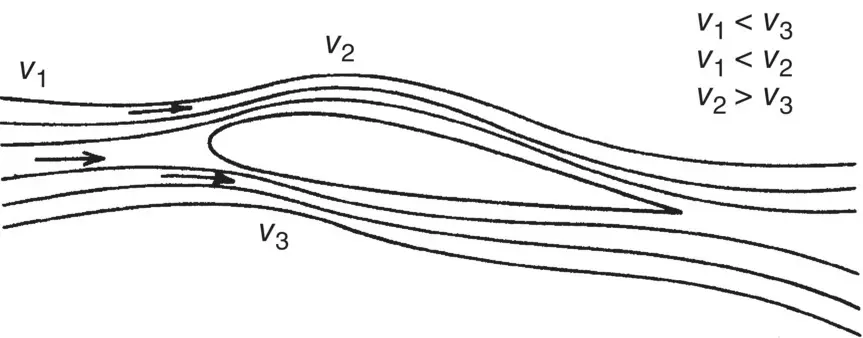
Figure 3.19 Velocity changes around an airfoil.

Figure 3.20 Static pressure on an airfoil (a) at zero AOA, and (b) at a positive AOA.

At a point near maximum thickness, maximum velocity and minimum static pressure will occur, note that V 2is greater than V 3. This difference in velocity results in pressure differential, and is due to the cambered nature of the airfoil, increased angle of attack, or both. If the airfoil was a symmetrical airfoil at zero angle of attack then the pressure would be equal on both the top and the bottom of the wing. Because air has viscosity, some of its energy will be lost to friction and a “wake” of low‐velocity, turbulent air exists near the trailing edge, resulting in a small, high‐pressure area.

Figure 3.20shows a symmetrical airfoil (a) at zero AOA and the resulting pressure distribution (b) at a positive AOA and its pressure distribution. Arrows pointing away from the airfoil indicate static pressures that are below ambient static pressure; arrows pointing toward the airfoil indicate pressures higher than ambient. Note in (b) that the largest magnitude arrow pointing away from the symmetrical airfoil is near the point of the highest velocity (dynamic pressure), so this is the point of greatest pressure differential between the static pressure over the wing and ambient pressure, as static pressure is at its lowest point.

Aerodynamic force (AF) is the resultant of all static pressures acting on an airfoil in an airflow multiplied by the planform area that is affected by the pressure. The line of action of the AF passes through the chord line at a point called the center of pressure (CP). It is convenient to consider that the forces acting on an aircraft, or on an airfoil, do so in some rectangular coordinate system. One such system could be defined by the longitudinal and vertical axes of an aircraft. Another could be defined by axes parallel to and perpendicular to the earth’s surface. A third rectangular coordinate system is defined by the relative wind direction and an axis perpendicular to it. This last system is chosen to define lift and drag forces. Aerodynamic force (AF) is resolved into two components: one parallel to the relative wind, called drag ( D ), and the other perpendicular to the relative wind, called lift ( L ). Figure 3.21shows the resolution of AF into its components L and D .

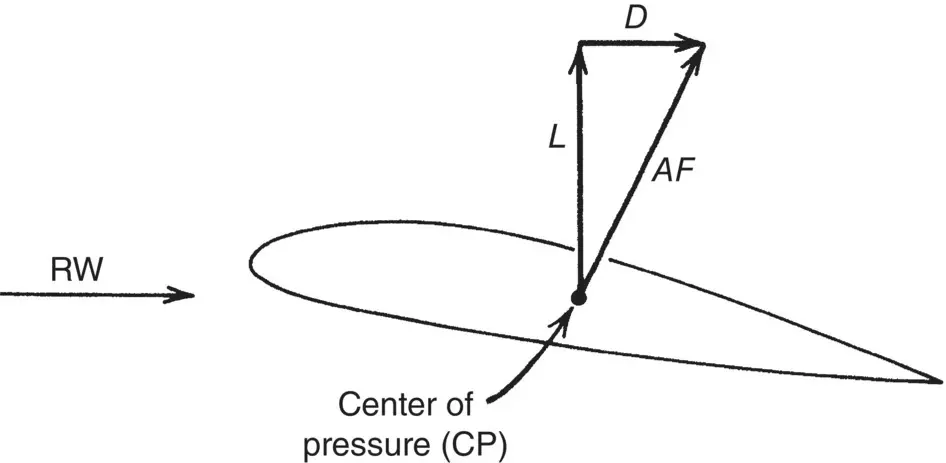
Figure 3.21 Components of aerodynamic force.

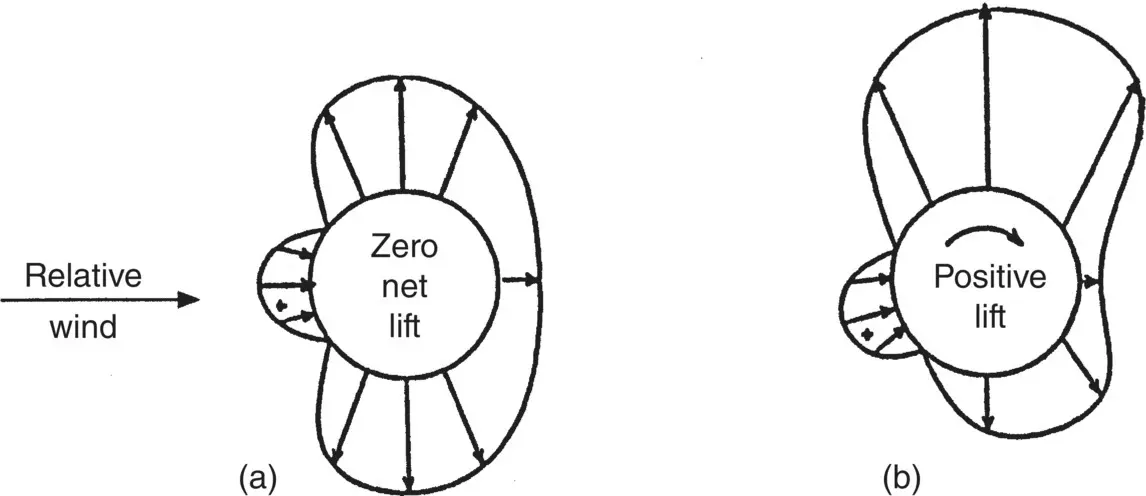
Figure 3.22 Pressure forces on (a) nonrotating cylinder and (b) rotating cylinder.

A stationary (nonrotating) cylinder is located in a wind tunnel as shown in Figure 3.22a. The cylinder is equipped with static pressure taps. These measure the local static pressure with respect to the ambient static pressure in the test chamber. When the tunnel is started, the airflow approaches the cylinder from the left as shown by the relative wind vector. Arrows pointing toward the cylinder show pressures that are higher (+) than ambient static pressure; arrows pointing away from the cylinder show pressures that are less (−) than ambient static pressure. Figure 3.22a shows that the upward forces are resisted by the downward forces and no net vertical force (lift) is developed by the cylinder.

Now consider if the wind tunnel is stopped, and the cylinder begins to rotate in a motionless fluid. We begin to see the factors of viscosity and friction at work. The more viscous the fluid, the more it is resistant to flow, and since air has viscosity properties it will resist flow. Similar to a wing, the surface of the cylinder has some “roughness” to it, so as the cylinder turns some molecules adhere to the surface. The closer to the surface of the cylinder (airfoil), the greater the possibility the molecules are drawn in a clockwise direction by viscosity, so now substituting air we see the velocity increase in the direction of rotation above the cylinder. This circular movement of the air is called circulation .

Finally, let us consider a rotating cylinder in a moving fluid as the cylinder continues rotating in the clockwise direction when the wind tunnel is once again started ( Figure 3.22b). The air passing over the top of the cylinder will be speeded up by circulation, while the air passing over the bottom of the cylinder will be retarded. According to Bernoulli’s equation, the static pressure on the top will be reduced and the static pressure on the bottom will be increased, similar to an airfoil with a positive angle of attack. The new pressure distribution will be as shown in Figure 3.22b, where a low‐pressure area produces an upward force. This is called the Magnus effect , named after Gustav Magnus, who discovered it in 1852. It explains why you slice (or hook ) your golf ball or why a good pitcher can throw a curve. The principles of the Magnus effect are even utilized in space as a spinning satellite can maintain its orbit longer due to the Magnus force, minimizing orbital decay.

Consider the pressure distribution about a symmetrical airfoil at zero angle of attack (AOA) ( Figure 3.23a). The large arrows show the sum of the low pressures on the top and bottom of the airfoil. They are at the center of pressure (CP) of their respective surfaces. The CP on the top of the airfoil and the CP on the bottom are located at the same point on the chord line. The large arrows indicate that the entire pressure on the top and bottom surfaces is acting at the CP. Because these two forces are equal and opposite in direction, no net lift is generated. Note also that the lines of action of these forces coincide, so there is no unbalance of moments about any point on the airfoil. Figure 3.23b shows the pressure distribution about a symmetrical airfoil at a positive angle of attack (AOA). There is now an imbalance in the upper surface and lower surface lift vectors, and positive lift is being developed. However, the two lift vectors still have the same line of action, passing through the CP. There can be no moment developed about the CP. We can conclude that symmetrical airfoils do not generate pitching moments at any AOA. It is also true that the CP does not move with a change in AOA for a symmetric airfoil.