Ziada et al (1989) propose a resonance parameter to assess susceptibility to resonance. For staggered arrays (triangular), this parameter has the form
(2‐43a) 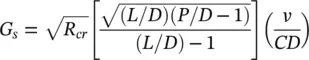
where R cris the Reynolds number based on the critical flow velocity at resonance, and v is the kinematic viscosity for the shell-side gas. The resonance parameter, G s, is correlated versus the flow path parameter, L/h , in Fig. 2-19b for both resonance and non‐resonance data. The parameter L/h is defined in Ziada et al (1989).
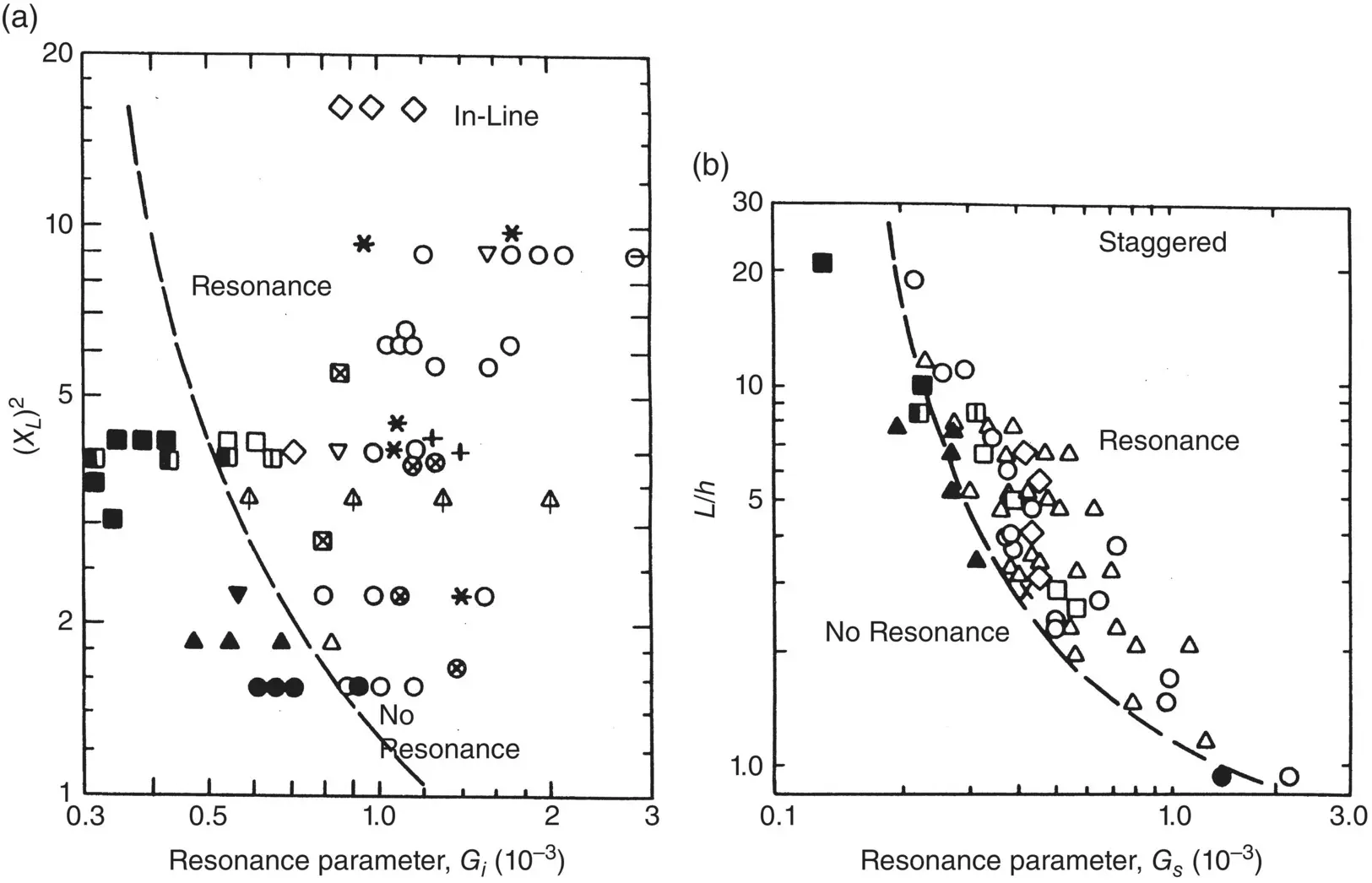
Fig. 2-19 Proposed Damping Criteria (Resonance Parameter) for: a) In‐Line (Square) Arrays; b) Staggered (Triangular) Arrays (Ziada et al, 1989 / with permission of Elsevier).
Similarly, for in‐line arrays (square)
(2‐43b) 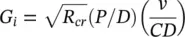
The parameter G iis plotted against ( XL ) 2in Fig. 2-19a, where XL = L / D . For a satisfactory design, it must be shown that the heat exchanger tube bundle is in the non‐resonance parts of Fig. 2-19.
2.5 Vibration Response Prediction
Due to the complexity of a multi‐span steam generator tube, a computer code is used to predict vibration response accurately and to determine the susceptibility of a tube to periodic‐wake‐shedding resonance and fluidelastic instability. Such a computer code must be capable of calculating modeshapes and natural frequencies for a number of modes at least equal to the number of tube spans.
Generally, the code will be in two parts: the first part calculates the free-vibration tube characteristics such as mode shapes and natural frequencies, while the second part is a forced vibration analysis that calculates the tube response to turbulence‐induced excitation and periodic wake shedding, and the fluidelastic instability ratios. A forced vibration analysis involves the application of the distributed flow velocities and densities that are determined using the methods outlined in Chapter 3.
From a mechanics point‐of‐view, the tubes are simply multi‐span beams clamped at the tubesheet and held at the supports with varying degrees of constraint. To predict tube response, it is convenient and appropriate to assume that the intermediate supports are hinged (or pinned). With this assumption, the analysis is linear and either a finite‐element code or an analytical code can be used, as discussed in Chapter 4.
If the tube‐to‐support clearances are too large, the tube supports will not be effective and the assumption of hinged supports will not be valid. Tube‐to‐support diametral clearances of 0.38 mm (0.015 in.) or less are typically used in nuclear heat-exchanger design. Effective supports can become ineffective if a heat exchanger is subjected to significant corrosion or a chemical cleaning technique is used to clean a fouled unit. The effects of future chemical cleaning and corrosion should be considered in the vibration response analysis.
The ultimate goal of vibration analyses is to ensure that neither fretting wear nor fatigue damage occurs in heat exchangers. Although a linear analysis does not predict fretting wear, fretting‐wear damage can be estimated, as discussed in Section 2.6.1.
Within vibration prediction codes, the tube response y ( x , t ) at any point x along the tube and at any time t may be expressed as a normal mode expansion in terms of the generalized coordinates q i (t) as follows:
(2‐44) 
where ϕ i( x ) is the mode shape of the i thmode and n is the number of modes to be used in the analysis. Using Lagrange’s equation and assuming that the damping is small and that it does not introduce coupling between modes, the equation of motion for the i thmode is as follows:
(2‐45) 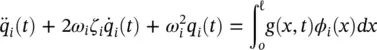
where ζ iis the damping ratio, ℓ is the tube length and ω iis the angular frequency of the i thnatural mode. The natural modes are normalized so that
(2‐46) 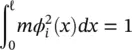
where m is the mass per unit length.
Knowing m , ζ i, the support locations and the flexural rigidity of the tube, the response to different types of forcing functions, g ( x , t ), is obtained by solving the family of equations described in Eqs. (2-44)and (2-45). Assumptions that can be used within this type of code include uniform mass distribution and flexural rigidity, negligible effect of shear and rotary inertia, no axial motion of any point along the tube, homogeneous boundary conditions and continuity at the intermediate supports.
2.5.1 Fluidelastic Instability
A fluidelastic instability ratio is determined by calculating an effective velocity based on the velocity and density profiles and the important mode shapes. For the i thmode, the critical velocity, U pc, at which instability occurs, may be expressed as:
(2‐47) 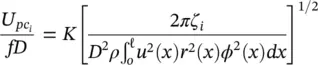
where non‐uniform flow velocities and densities are represented as U p( x ) = U p u ( x ) and ρ ( x ) = ρr ( x ) This is a generalized form of Eq. (2-25).
2.5.2 Random Turbulence Excitation
Tube response to turbulence‐induced excitation forces must be calculated using random vibration theory, and the response must be summed over all significant modes of vibration. The mean square amplitude response, 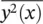 , of a continuous uniform cylindrical tube to distributed random forces, g(x,t) , may be expressed by:
, of a continuous uniform cylindrical tube to distributed random forces, g(x,t) , may be expressed by:
(2‐48) 
where H r *and H sare complex complementary frequency response functions for the r thand s thmodes, R(x,x ′ ,f) is the spatial correlation density function and x and x ′ are points along the length of the tube. More detail is found in Pettigrew et al (1978), Axisa et al (1990) and in Chapters 9and 10.
Читать дальше

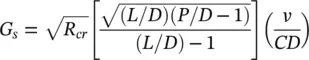

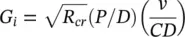

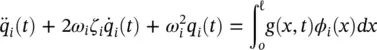
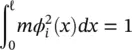
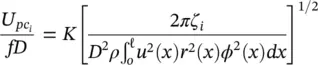
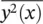 , of a continuous uniform cylindrical tube to distributed random forces, g(x,t) , may be expressed by:
, of a continuous uniform cylindrical tube to distributed random forces, g(x,t) , may be expressed by:











