Nirmal K. Sinha - Engineering Physics of High-Temperature Materials

Figure 1.6 Strain–time curves, showing rise time to apply full load (<1 s), during creep for five SRRTs, including the longer duration results of Figure 1.4, on a polycrystalline nickel‐base superalloy Waspaloy at 1005 K (732 °C) for initial stresses 450–650 MPa. Note the delayed elastic recovery and viscous strains after unloading.

Source: N. K. Sinha.

Figure 1.6points out that the time to mcr, t m, decreased significantly, over an order of magnitude, with increase in engineering stress from 450 to 650 MPa. The dependence of t mon stress, σ , may be expressed by the following relationship:

(1.2)

where M = 1.61 × 10 29and p = 9.36. Such a relationship has commonly been seen for the dependence of rupture or fracture time on stress, leading to popular ideas on relating mcr to failures at high temperatures (see Chapter 6). Note that the total strain, ε min(which includes the elastic strain, ε e), corresponding to mcr increased only from 0.38% for 450 MPa to about 0.64% for 650 MPa. The corresponding values of the creep strain ( ε min− ε e) increased from 0.12 to 0.27%. This type of diminished stress dependency of ε minagrees with general observations available in the literature on the relatively small increase in strain at mcr with increase in stress. In fact, the elongation at fracture hardly varies with stress. However, note the increase in the permanent or viscous strain, ε v, at unloading time of the tests, with increase in stress. Viscous strain thus provides a measure of the permanent change in the structure.

The analysis in Figure 1.7clearly demonstrates that (i) the amount of delayed elastic strain, des, accumulated during the tests is not negligible and that (ii) it is not consumed within the mass and is recoverable at the end of the tests even well within the tertiary stages. The set of these types of creep and recovery curves illustrates the potentials of the SRRTs.

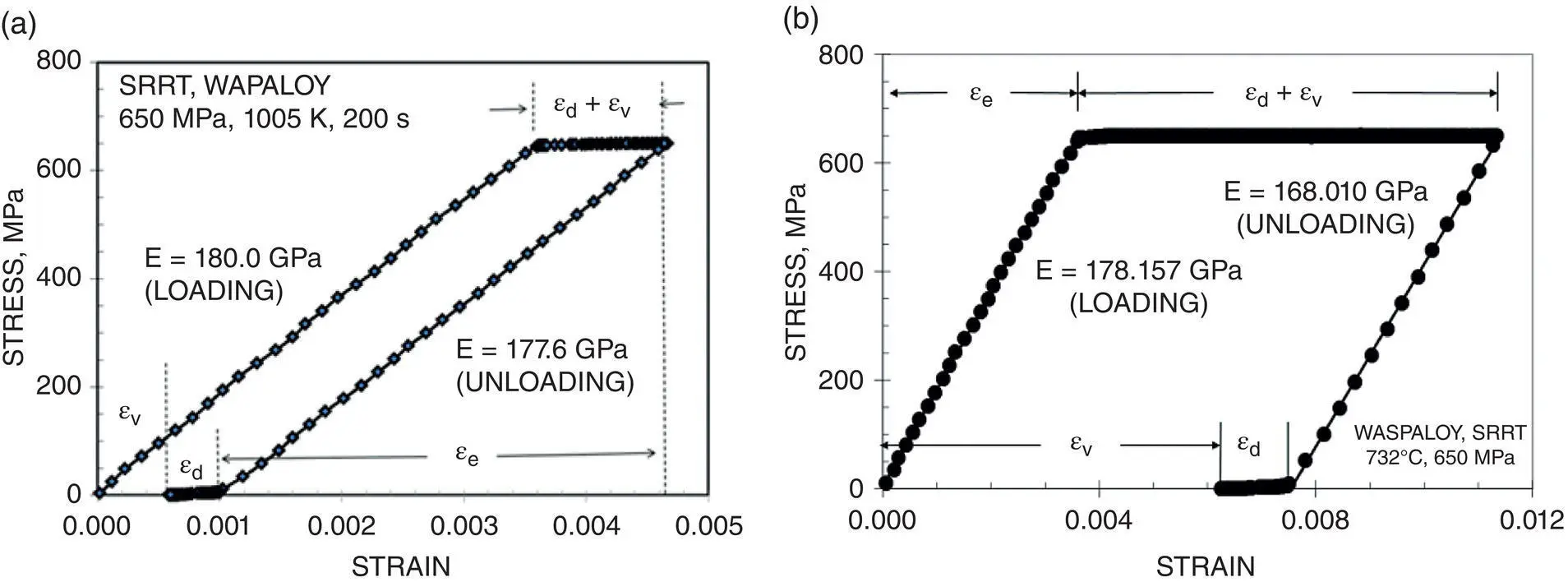
Figure 1.7 Stress–strain diagram. (a) Two values of elastic modulus, E , and strain trinity (elastic, delayed elastic, and viscous) for loading and unloading the 200 s SRRT of Figure 1.5on Waspaloy at 1005 K and 650 MPa. (b) Stress–strain diagram, and the strain components for the longer‐term (2341 s) test in Figures 1.4and 1.5on Waspaloy at 732 °C (1005 K) and 650 MPa; the lower value of E (168 GPa) during unloading compared to 178 GPa during loading indicates structural damage during tertiary creep with slight increase in true stress.

Source: N. K. Sinha.

Stress–strain ( σ – ε ) diagrams for engineering materials are commonly “reserved” for constant displacement (nominally constant strain rate) tests from which some sort of “effective elastic modulus” is evaluated. Most textbooks on mechanics talk about this aspect and define engineering properties of materials accordingly. Hysteresis loop or stress–strain diagrams for constant stress (load) creep tests are unheard or not commonly discussed, perhaps because of uncertainty of the loading and unloading phases of the common creep tests using dead loads.

Computer‐controlled SRRTs provide unique opportunities for examining the effective elastic modulus during both loading and unloading times, and examine the differences (if any) associated with creep damages. An example is shown in Figure 1.7a for the 200 s SRRT of Figures 1.4and 1.5and this illustrates the possibilities of determining E during loading and unloading sequences. Such stress–strain diagrams for creep tests are unheard of to most materials scientists.

Comparing the E values of 180.0 GPa during the rise time to apply the full load and that of 177.6 GPa obtained for fall time (unloading) demonstrates that these values are complimentary and close to the dynamic values of previously undeformed and undamaged Young's modulus E of Waspaloy at comparable temperatures determined from seismic methods.

Figure 1.7b shows a stress–strain diagram for the longer (2341 s) duration SRRT of Figure 1.5. In this case, the E value of 178.2 GPa during the loading sequence is very close to those for short‐term loading and unloading periods in Figure 1.7a, but noticeably lower E value of 168.0 GPa obtained during the unloading period. This lower E value after longer‐term creep is indicative of a measure of the structurally “damaged” state of the material undergoing minimum creep rate and subsequent tertiary creep stage.

Figure 1.7also reveals a particularly important aspect of constant‐stress creep tests by noting the experimentally determined amounts of the three components of strain: elastic, delayed elastic, and viscous. Most important is the fact that the delayed elastic contribution to the total strain is measurable and not negligible. In the case of the 200 s test, ε dis comparable to ε v, whereas it is significantly lower in the case of the longer‐term 2341 s test. There was, therefore, a significant contribution of delayed elastic (anelastic) strain to the total “inelastic strain” at the time of the minimum creep rate. Realistic rheological models for high‐temperature engineering applications cannot ignore the contributions of delayed elastic strain, known to be associated with grain‐boundary shearing processes (Sinha 1979).

Rheological models must be able to quantify the trinity or three‐component aspects of creep and relate to failure processes; this is covered in Chapters 5– 8. It is shown in Chapter 5that the delayed elastic effect could vary from a linear to a highly nonlinear response, but during primary creep the ratio, n v/ s , of stress exponents, n v, for viscous flow (dislocation creep) and, s , for delayed elasticity could be very similar for crystalline materials in general. Since delayed elasticity has been linked strongly to grain‐boundary shearing processes, metallurgical and process engineering for superalloys may be directed toward (grain‐boundary engineering) increasing this n v/ s ratio to decrease the propensity for generating intergranular voids and cracks.

Figure 1.8presents five sets of results for different specimens on the stress dependence of viscous strain rate during the primary creep obtained from short‐term SRRTs, and the corresponding minimum creep rate obtained from the long‐term tests. The same (or numerically very close) power law with a stress exponent of 11.0 applies for both n vfor viscous strain rate during primary creep and n minfor the minimum creep rate. These results and more are discussed in detail in Chapter 6for a number of different materials in conjunction with development of rheological equations for the primary or transient stage of creep.

Creep theories for polycrystals are essentially based on idealized microstructures. This is essential to keep the number of material parameters as low as possible. To check the theoretical predictions, ideally experiments should be performed on specimens with exactly the same microstructure and chemical characteristics. In reality, it is impossible to have polycrystalline samples with exactly the same microstructure, i.e. the same number of grains, sizes, distribution, orientations, etc. CL creep tests are mostly carried out. Tests aimed at exploring the stress exponent ( n minor n m) characterizing the dependence of steady state or minimum creep rate (mcr) on stress and temperature are therefore performed on different specimens with similar physical qualities. However, mcr may occur at a relatively large strain with evolved microstructure depending on the initial structures, stress, temperature, and test environment (air, gas, or vacuum). All the tests are different even though performed under similar conditions. Is it possible to use one specimen (i.e. constant microstructure) to perform a number of very short‐term creep tests and determine n vfor viscous flow (dislocation creep)? The answer will be a quick “no,” “impossible.” This quick answer is based on classical approaches that have been taken for many decades. This is where a change in paradigm is required; Figures 1.4– 1.7are illustrations of this possibility using SRRT – a stylized name for a simple creep and recovery test originally developed for ice (Sinha 1978a), later coined by Sinha (2001), and used extensively for advanced aerospace alloys.