So far, we have considered point groups that have a single symmetry element. The three orthorhombic point groups shown in Fig. 1.55 all have combinations of symmetry elements. For each, again, two diagrams are used. First, point group 222 has the three, mutually perpendicular twofold axes shown in (b): one is perpendicular to the plane of the projection, passes through the centre and is represented by the lens‐shaped symbol in the centre of the projection; one runs vertically in the plane of the projection and is represented by two symbols on the circumference of the circle at the top and bottom; one runs horizontally in the plane of the projection and is represented by the two symbols at the left and right on the circle circumference.
In order to generate equivalent positions in point group 222, it is necessary to first, identify a starting position, shown in Fig. 1.56(a), and operate on that position with one of the symmetry elements, for example the 2‐fold axis perpendicular to the plane of the projection. This generates a new equivalent position shown in (b). The operation is then repeated until arriving back at the starting position. The next step, 2 is to consider the effect of the symmetry operations associated with one of the other symmetry elements, which generates an additional set of equivalent positions. This is shown in (c) for the 2‐fold axis running horizontally and in the plane of the paper; each of the equivalent positions in (b), above the plane of the projection, generates a new position below the plane. Finally, the effect of the third symmetry element, step 3 is considered.
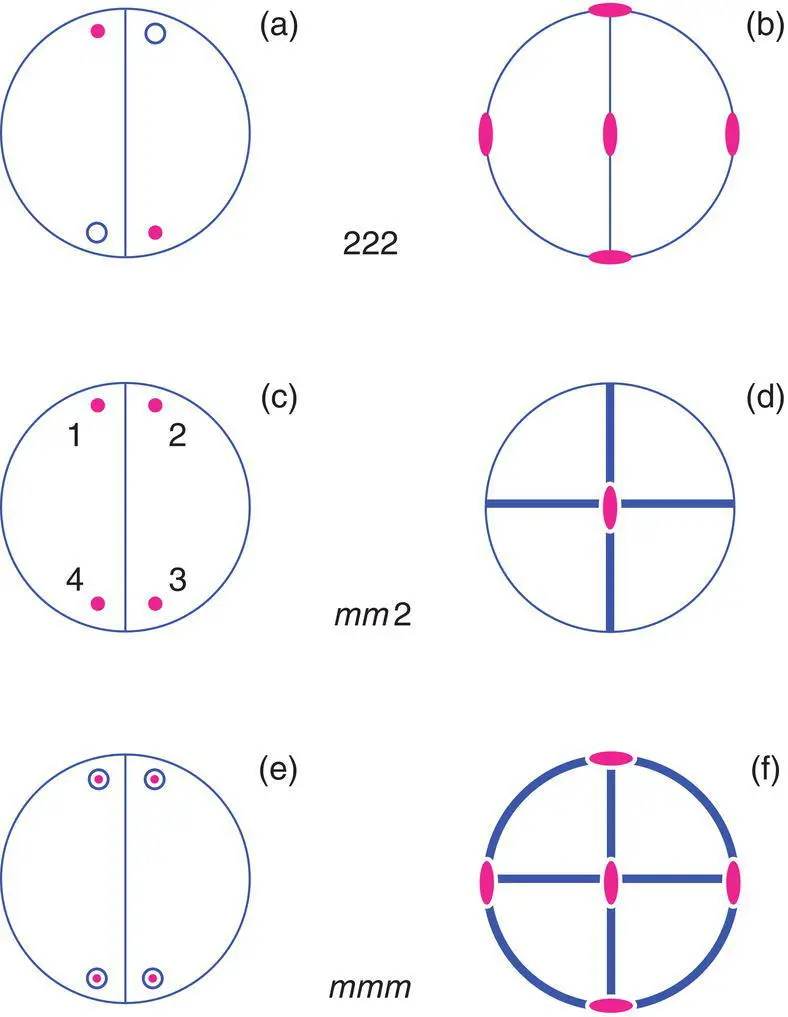
Figure 1.55 The three orthorhombic point groups 222, mm2, and mmm.
In this case, however, no new equivalent positions are generated in (d). The third 2‐fold axis, shown vertically in the plane of the paper, is essentially redundant since it arises automatically from the presence of the other 2‐fold axes. The point group 222 could therefore be represented in the shortest possible notation as 22 because the third twofold axis is not independent. The longer notation is used in order to show consistency with the essential symmetry requirements (Table 1.1) for orthorhombic unit cells. The order in which the symmetry axes are considered in Fig. 1.56 is unimportant and any two of the 2‐fold axes together generate the third axis automatically.
Orthorhombic point group mm 2, Fig. 1.55 contains two mirror planes at right angles to each other with a 2‐fold axis passing along the line of intersection of the mirror planes (d). The 2‐fold axis is perpendicular to the paper and the mirror planes are indicated in projection as the thick lines lying horizontally and vertically. This point group also has four equivalent positions, but all are at the same height relative to the plane of the paper (c). As in the previous example, the third symmetry element is not independent but is generated by the combined operation of any of the other two elements. The choice of order of the symmetry elements is again immaterial; any two out of the three, in combination, will generate the third element.
Orthorhombic point group mmm , Fig. 1.55, contains, as essential symmetry elements, three mirror planes mutually perpendicular to each other; as a consequence of the mirror planes, three mutually perpendicular 2‐fold axes are generated. (Note: the reverse process does NOT occur; the three 2‐fold axes in 222 do not lead to the automatic generation of mirror planes) These symmetry elements are shown in (f) with the 8 equivalent positions in (e). In this case, all three mirror planes are essential for the point group mmm .
Only three orthorhombic point groups are possible. If other combinations of 2‐fold axes and mirror planes are considered, they will turn out to be equivalent to one of the three allowed point groups. For instance, the combination 22 m can be shown to yield the same set of positions and symmetries as mmm . Of the three orthorhombic point groups, only mmm possesses a centre of symmetry (the equivalent positions created by a centre of symmetry are shown in Fig. 1.54(a) for the triclinic point group 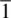 ). Each position in mmm has a centrosymmetrically related partner, but this is not the case for 222 and mm 2. The relevance of centrosymmetry is discussed in Section 1.18.4.
). Each position in mmm has a centrosymmetrically related partner, but this is not the case for 222 and mm 2. The relevance of centrosymmetry is discussed in Section 1.18.4.
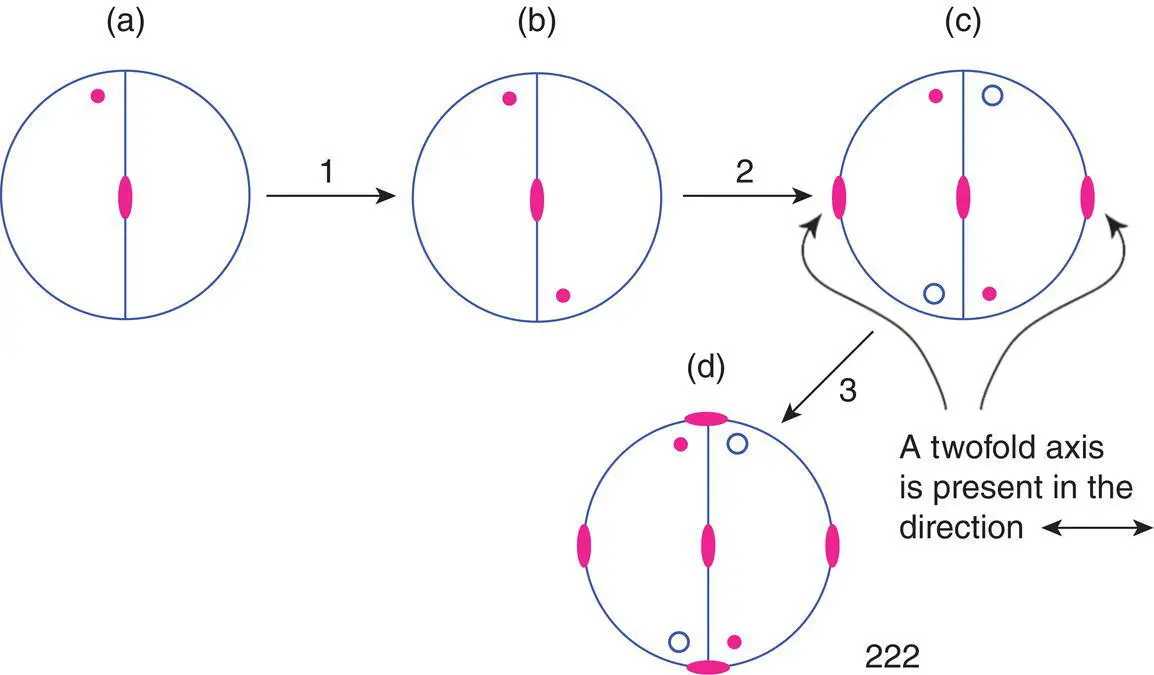
Figure 1.56 Equivalent positions in the point group 222. In step 1, a 2‐fold axis perpendicular to the plane of the paper is added. In step 2, a second 2‐fold axis, running horizontally in the plane of the paper is added. In step 3, a third 2‐fold axis, running vertically in the plane of the paper has been added but is created automatically by step 2.
As a final example, consider the trigonal point group 32 which is characterised by a single 3‐fold axis with a perpendicular 2‐fold axis, Fig. 1.57. The 3‐fold axis is oriented perpendicular to the plane of the paper (b). There are three 2‐fold axes lying in the plane of the paper and at 60° to each other, but only one of these is independent. To demonstrate this and find the equivalent positions, start with position 1 and consider the effect of the 3‐fold axis (rotation by 120°). Positions 3 and 5 result (a). Then consider the effect of one of the 2‐fold axes, say XX′ in (b). This generates three new positions: 1 → 4, 3 → 2 and 5 → 6; also, two more 2‐fold axes YY′ and ZZ′ are automatically generated, e.g. YY′ relates positions 1 and 6, 2 and 5, 3 and 4.
Of the 32 crystallographic point groups, 27 are non‐cubic and we have looked at 9 of these. The remaining 18, Appendix E, can be treated along similar lines and should cause no problem for the reader. The main difficulty likely to be encountered concerns the orientation of the different symmetry elements in a point group. Some guidelines are as follows: (i) in monoclinic, hexagonal, trigonal and tetragonal point groups, the unique axis is shown perpendicular to the plane of the paper (stereogram); (ii) an oblique line, as in 4 /mmm , indicates that, in this case, the 4‐fold axis has a mirror plane perpendicular to it: it would be clearer if the symbol were written as (4 /m ) mm ; (iii) in tetragonal, trigonal and hexagonal point groups, the 2‐fold axes, as in  2 m, are always in the plane perpendicular to the unique axis (i.e. perpendicular to
2 m, are always in the plane perpendicular to the unique axis (i.e. perpendicular to 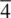 ).
).
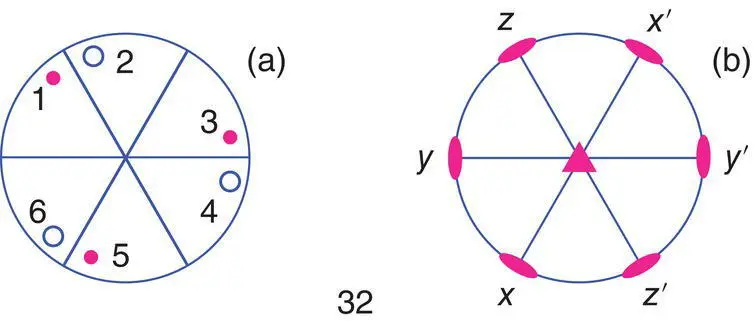
Figure 1.57 Trigonal point group 32.
The 5 cubic point groups are rather more complicated to work with as they are difficult to represent by simple 2D projections. This is because (i) there are so many symmetry elements present and more importantly, (ii) many are not perpendicular to each other. Whereas for non‐cubic point groups, the symmetry axes are either in the plane or perpendicular to the plane of the stereograms, this is not generally possible for cubic point groups and oblique projections are needed to represent the 3‐fold axes. No further discussion of cubic point groups, which are shown in Appendix E, is given.
Читать дальше


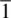 ). Each position in mmm has a centrosymmetrically related partner, but this is not the case for 222 and mm 2. The relevance of centrosymmetry is discussed in Section 1.18.4.
). Each position in mmm has a centrosymmetrically related partner, but this is not the case for 222 and mm 2. The relevance of centrosymmetry is discussed in Section 1.18.4.
 2 m, are always in the plane perpendicular to the unique axis (i.e. perpendicular to
2 m, are always in the plane perpendicular to the unique axis (i.e. perpendicular to 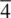 ).
).











