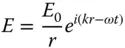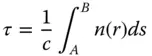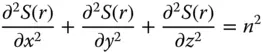Stephen Rolt - Optical Engineering Science

Of course, in reality, the local electric field is a vectorquantity and the scalar theorypresented here is a useful initial simplification. Breakdown of this approximation will be considered later when we consider polarisation effects in light propagation. If one imagines waves propagating from a central point, the wave equation offers solutions of the following form:

(1.2)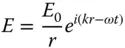

Equation (1.2)represents a spherical wave of angular frequency, ω, and spatial frequency, or wavevector, k. The velocity that the wave disturbance propagates with is ω/k or c/ n . In free space, light propagates at the speed of light, c, a fundamental and definedconstant in the SI system of units. Thus, the refractive index, n , is the ratio of the speed of light in free space to that in the specified medium. All points lying at the same distance, r , from the source, will oscillate at an angular frequency, ω, and in the same phase. Successive surfaces, where all points are oscillating entirely in phase are referred to as wavefronts and can be viewed at the crests of ripples emanating from a point disturbance. This is illustrated in Figure 1.2. This picture provides us with a more coherent definition of a ray. A ray is represented by the vector normal to the wavefront surface in the direction of propagation . Of course, Figure 1.2represents a simple spherical wave, with waves spreading from a single point. However, in practice, wavefront surfaces may be much more complex than this. Nevertheless, the precise definition of a ray remains clear:

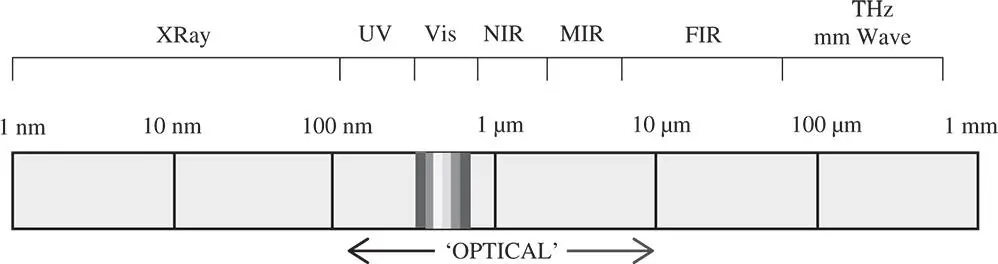
Figure 1.1 The electromagnetic spectrum.

Figure 1.2 Relationship between rays and wavefronts.

At any point in space in an optical field, a ray may be defined as the unit vector perpendicular to the surface of constant phase at that point with its sense lying in the same direction as that of the energy propagation .

Intuition tells us that light ‘travels in straight lines’. That is to say, light propagates between two points in such a way as to minimise the distance travelled. More generally, in fact, all geometric optics is governed by a very simple principle along similar lines. Light always propagates between two points in space in such a way as to minimise the time taken. If we consider two points, A and B, and a ray propagating between them within a medium whose refractive index is some arbitrary function, n ( r ), of position then the time taken is given by:

(1.3)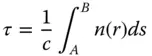

c is the speed of light in vacuo and ds is an element of path between A and B

This is illustrated in Figure 1.3.

Figure 1.3 Arbitrary ray path between two points.

Fermat's principle may then be stated as follows:

Light will travel between two points A and B such that the path taken represents a local minimum in the total optical path between these points .

Fermat's principle underlies all ray optics. All laws governing refraction and reflection of rays may be derived from Fermat's principle. Most importantly, to demonstrate the theoretical foundation of ray optics and its connection with physical or wave optics, Fermat's principle may be directly derived from the wave equation. This proof demonstrates that the path taken represents, in fact, a stationarysolution with respect to other possible paths. That is to say, technically, the optical path taken couldrepresent a local maximumor inflexionpoint rather than a minimum. However, for most practical purposes it is correct to say that the path taken represents the minimum possible optical path.

Fermat's principle is more formally set out in the Eikonal equation. Referring to Figure 1.2, if instead of describing the light in terms of rays it is described by the wavefront surfaces themselves. The function S ( r ) describes the phase of the wave at any point and the Eikonal equation, which is derived from the wave equation, is set out thus:

(1.4)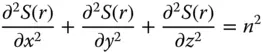

The important point about the Eikonal equation is not the equation itself, but the assumptions underlying it. Derivation of the Eikonal equation assumes that the rate of change in phase is small compared to the wavelength of light. That is to say, the radius of curvature of the wavefronts should be significantly larger than the wavelength of light. Outside this regime the assumptions underlying ray optics are not justified. This is where the effects of the wave nature of light (i.e. diffraction) must be considered and we enter the realm of physical optics. But for the time being, in the succeeding chapters we may consider that all optical systems are adequately described by geometrical optics.

So, for the purposes of this discussion, it is one simple principle, Fermat's principle, that provides the foundation for all ray optics. For the time being, we will leave behind specific consideration of the detailed behaviour of individual optical surfaces. In the meantime, we will develop a very generalised description of an idealised optical system that does not attribute specific behaviours to individual components. Later on, this ‘black box model’ will be used, in conjunction with Gaussian optics to provide a complete first order description of complex optical systems.

In applying geometrical optics to a real system, we are attempting to determine the path of a ray(s) through the system. There are a few underlying characteristics that underpin most optical systems and help to simplify analysis. First, most optical systems are sequential. An optical system might comprise a number of different elements or surfaces, e.g. lenses, mirrors, or prisms. In a sequential optical system, the order in which light propagates through these components is unique and pre-determined. Second, in most practical systems, light is constrained with respect to a mechanical or optical axis of symmetry, the optical axis, as illustrated in Figure 1.4. In real optical systems, light is constrained by the use of physical apertures or ‘stops’; this will be discussed in more detail later.

Of course, in practice, the optical axis need not be a continuous, straight line through an optical system. It may be bent, or folded by mirrors or prisms. Nevertheless, there exists an axis throughout the system with respect to which the rays are constrained.

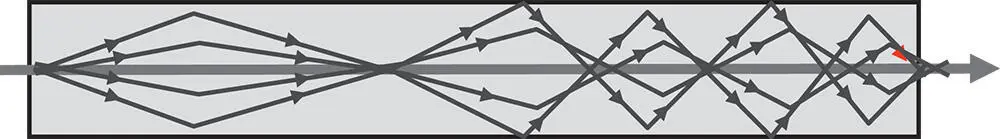
Figure 1.4 Constraint of rays with respect to optical axis.