1 ...6 7 8 10 11 12 ...32
1.2.4.1 BeiDou Satellites
BeiDou will consist of 27 MEO satellites, including 5 geostationary Earth orbit (GEO) satellites and 3 inclined geosynchronous orbit (IGSO) satellites. The GEO and IGSO satellites will be at longitudes to support the China and the surround areas of Southeast Asia.
The BDS‐2 and BDS‐3 operate on various frequencies in the L1 (BDS‐3 B1C signal at 1575.42 MHz), E6 (BDS B3I signal at 1268.5 MHz), E5 (BDS‐2 and BDS‐3 at 1207.14 MHz; and BDS‐3 (B2a) at 1176.45 MHz). These signals use various navigation data formats from the BDS MEO, GEO, or IGSO satellites to support global and regional civil services. Details of this section are given in Chapter 4.
1.2.5 Regional Satellite Systems
There are several regional satellite systems that provide regional navigation and/or augmentation service.
Quasi‐Zenith Satellite System (QZSS) is satellite‐based navigation system being developed by the Japanese government. QZSS has a constellation of four IGSO satellites that provide navigation and augmentation to GPS over Japan and Southeast Asia. The system transmits GPS‐type signals: L1 (L1 C/A and L1C), L2C, and L5, as well as, augmentation signals to support submeter and centimeter level services. Details of this section are given in Chapter 4.
The Indian Regional Navigation Satellite Systems, operationally known as NAVIC, is a regional satellite navigation system developed by the Indian Space Research Organization (ISRO). NAVIC constellation consists of eight satellites operation in GEO‐ and IGSO‐type orbits, where satellites transmit navigation signal in the L5 and S‐band. Details of this section are given in Chapter 4.
1.3 Inertial Navigation Overview
The following is a more‐or‐less heuristic overview of inertial navigation technology. Chapter 3 has the essential technical details about hardware and software used for inertial navigation, and Chapter 11 is about analytical methods for statistical characterization of navigation performance.
Although the theoretical foundations for inertial navigation have been around since the time of Isaac Newton (1643–1727), the technology for reducing it to practice would not become available until the twentieth century. This history can be found in the accounts by Draper [22], Gibson [23], Hellman [24], Mackenzie [2], Mueller [18], Wagner [25], and Wrigley [26]. For an account of the related computer hardware and software developments through that period, see McMurran [27].
1.3.1.1 Theoretical Foundations
It has been called “Newtonian navigation” [28] because its theoretical foundations have been known since the time of Newton. 2
Given the position x( t 0) and velocity v( t 0) of a vehicle at time t 0, and its acceleration a( s ) for times s > t 0, then its velocity v( t ) and position x( t ) for all time t > t 0can be defined as
(1.1) 
(1.2) 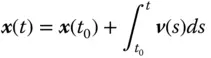
It follows that given the initial position x( t 0) and velocity v( t 0) of a vehicle or vessel, its subsequent position depends only on its subsequent accelerations. If these accelerations could be measured and integrated, this would provide a navigation solution.
Indications that this can be done can be found in nature:
1 Halters are an extra set of modified wings on some flying insects. During flight, these function as Coriolis vibratory gyroscopes (CVGs) to provide rotation rate feedback in attitude control. Their function was not known for a long time because they are too tiny to observe.
2 Vestibular systems include ensembles of gyroscopes (semicircular canals) and accelerometers (saccule and utricle) located in the bony mass behind each of your ears. Each of these is a complete 3D inertial measurement unit (IMU), used primarily to aid your vision system during rotations of the head but also useful for short‐term navigation in total darkness. These have been evolving since the time your ancestors were fish [29].
The development of man‐made solutions for inertial navigation came to have the same essential parts, except that they are not biological (yet).
1.3.1.2 Development Challenges: Then and Now
The technology of Newton's time was not adequate for practical implementation. What was missing included the following:
1 Methods for measuring three components of the acceleration vector a(t) in Eq. (1.1)with sufficient accuracy for the navigation problem.
2 Methods for maintaining the representation of vectors a(t) and v(t) in a common inertial coordinate frame for integration.
3 Methods for integrating a(t) and v(t) in real time (i.e. fast enough for the demands of the application).
4 Methods for initializing v(t) and x(t) in the common inertial coordinate frame.
5 Applications to justify the investments in technology required for developing the necessary solutions. It could not be justified for transportation at the pace of a sailing ship or a horse, but it could (and would) be justified by the military activities of World War II and the Cold War.
These challenges for inertial navigation system (INS) development were met and overcome during the great arms races of the mid‐to‐late twentieth century, the same time period that gave us GNSS. Like GNSS, INS technology first evolved for military applications and then became available for consumer applications as well. Today, the same concerns that have given us chip‐level GNSS receivers are giving us chip‐level inertial navigation technology. Chapter 3 describes the principles behind modern‐day inertial sensors, often using macro‐scale sensor models to illustrate what may be less obvious at the micro‐scale. The following subsections provide a more heuristic overview.
1.3.2 Development Results
1.3.2.1 Inertial Sensors
Rotation sensors are used in INSs for keeping track of the directions of the sensitive (input) axes of the acceleration sensors – so that sensed accelerations can be integrated in a fixed coordinate frame. Sensors for measuring rotation or rotation rates are collectively called gyroscopes , a term coined by Jean Bernard Léon Foucault (1819–1868). Foucault measured the rotation rate of Earth using two types of gyroscopes:
1 What is now called a momentum wheel gyroscope (MWG), which – if no torques are applied – tends to keep its spin axis in an inertially fixed direction while Earth rotates under it.
2 The Foucault pendulum, which might now be called a vibrating Coriolis gyroscope (vibrating at about 0.06 Hz). It depends on sinusoidal motion of a proof mass and the Coriolis 3 effect, an important design principle for miniature gyroscopes, as well.
Some of the physical phenomena used in the design of gyroscopes are listed in Table 1.1. Further explanation of the terminology is provided in Chapter 3.
Table 1.1A sampling of inertial sensor types.
| What it measures |
Sensor types |
Physical phenomena |
Implementation methods |
| Rotation (gyroscope) |
Momentum wheel gyroscope (MWG) |
Conservation of angular momentum |
Angle displacement |
| Torque rebalance |
| Coriolis vibratory gyroscope (CVG) |
Coriolis effect and vibration |
Balanced (tuning fork) |
| Wine glass resonance |
| Optical gyroscope |
Sagnac effect |
Fiber optic gyroscope (FOG) |
|
Ring laser gyroscope (RLG) |
| Acceleration (accelerometer) |
Mass spring (fish example) |
Stress in support |
Piezoresistive |
|
Piezoelectric |
|
|
Surface acoustic wave |
|
|
Vibrating wire in tension |
| Electromagnetic |
Induction |
Drag cup |
| Electromagnetic force |
Force rebalance |
| Pendulous integrating gyroscopic accelerometer (PIGA) |
Gyroscopic precession |
Angular displacement |
| Torque rebalance |
| Electrostatic |
Electrostatic force |
Force rebalance |
What we call acceleration sensors or accelerometers actually measure specific force , equal to the physical force applied to a mass divided by the mass, solving f = ma for a , given f (the sensor input) and m (a known constant). Accelerometers do not measure gravitational acceleration , but can measure the force applied to counter gravity. A spring scale used in a fish market for measuring the mass m of a fish, for example, is actually measuring the spring force f applied to the fish by the spring for countering gravity, solving f = ma for m , given f (spring strain, proportional to force) and a (local gravity being countered).
Читать дальше