Patrick Muldowney - Gauge Integral Structures for Stochastic Calculus and Quantum Electrodynamics
Здесь есть возможность читать онлайн «Patrick Muldowney - Gauge Integral Structures for Stochastic Calculus and Quantum Electrodynamics» — ознакомительный отрывок электронной книги совершенно бесплатно, а после прочтения отрывка купить полную версию. В некоторых случаях можно слушать аудио, скачать через торрент в формате fb2 и присутствует краткое содержание. Жанр: unrecognised, на английском языке. Описание произведения, (предисловие) а так же отзывы посетителей доступны на портале библиотеки ЛибКат.
- Название:Gauge Integral Structures for Stochastic Calculus and Quantum Electrodynamics
- Автор:
- Жанр:
- Год:неизвестен
- ISBN:нет данных
- Рейтинг книги:3 / 5. Голосов: 1
-
Избранное:Добавить в избранное
- Отзывы:
-
Ваша оценка:
Gauge Integral Structures for Stochastic Calculus and Quantum Electrodynamics: краткое содержание, описание и аннотация
Предлагаем к чтению аннотацию, описание, краткое содержание или предисловие (зависит от того, что написал сам автор книги «Gauge Integral Structures for Stochastic Calculus and Quantum Electrodynamics»). Если вы не нашли необходимую информацию о книге — напишите в комментариях, мы постараемся отыскать её.
, left off,
introduces readers to particular problems of integration in the probability-like theory of quantum mechanics. Written as a motivational explanation of the key points of the underlying mathematical theory, and including ample illustrations of the calculus, this book relies heavily on the mathematical theory set out in the author’s previous work. That said, this work stands alone and does not require a reading of
in order to be understandable.
Gauge Integral Structures for Stochastic Calculus and Quantum Electrodynamics Stochastic calculus, including discussions of random variation, integration and probability, and stochastic processes. Field theory, including discussions of gauges for product spaces and quantum electrodynamics. Robust and thorough appendices, examples, illustrations, and introductions for each of the concepts discussed within. An introduction to basic gauge integral theory. The methods employed in this book show, for instance, that it is no longer necessary to resort to unreliable «Black Box» theory in financial calculus; that full mathematical rigor can now be combined with clarity and simplicity. Perfect for students and academics with even a passing interest in the application of the gauge integral technique pioneered by R. Henstock and J. Kurzweil,
is an illuminating and insightful exploration of the complex mathematical topics contained within.

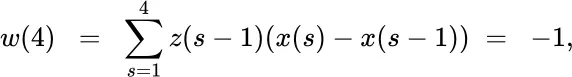
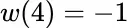 is a “negative gain” or net loss. With
is a “negative gain” or net loss. With  , this can be interpreted as the Stieltjes integral 3
, this can be interpreted as the Stieltjes integral 3
 of shares held at any time
of shares held at any time 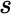 depends on whether the share price
depends on whether the share price 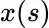 has moved up or down. So
has moved up or down. So 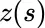 ,
, 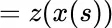 , is a deterministic function of
, is a deterministic function of 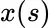 ; and the value of
; and the value of  varies randomly because
varies randomly because 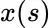 varies randomly.
varies randomly. , including the terminal value
, including the terminal value 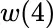 , or
, or  with
with 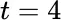 . Table 2.5gives the respective process sample paths for processes,
. Table 2.5gives the respective process sample paths for processes, 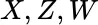 where the underlying share price process
where the underlying share price process  follows sequence DUUU (sample number 2 in Table 2.4).
follows sequence DUUU (sample number 2 in Table 2.4). ,
,  (and so on) are used here, in contrast to symbols
(and so on) are used here, in contrast to symbols  etc. which were used in discussion of stochastic calculus in Chapter 1. In the latter, the emphasis was on the classical rigorous theory in which random variables are measurable functions, and this is signalled by using
etc. which were used in discussion of stochastic calculus in Chapter 1. In the latter, the emphasis was on the classical rigorous theory in which random variables are measurable functions, and this is signalled by using  instead of
instead of 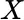 , etc.
, etc. (rather than
(rather than 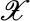 etc.) is used, the purpose is to indicate the “naive” or “natural” outlook which sees random variability, not in terms of abstract mathematical measurable sets and functions, but in terms of actual occurrences such as tossing a coin, or such as the unpredictable rise and fall of prices.
etc.) is used, the purpose is to indicate the “naive” or “natural” outlook which sees random variability, not in terms of abstract mathematical measurable sets and functions, but in terms of actual occurrences such as tossing a coin, or such as the unpredictable rise and fall of prices. etc.
etc.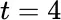 , what is the value 4 of
, what is the value 4 of 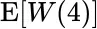 ?
?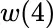 can be easily calculated, as in Table 2.5above. In fact, the 16 outcomes for net wealth (shareholding value) gain are
can be easily calculated, as in Table 2.5above. In fact, the 16 outcomes for net wealth (shareholding value) gain are










