Patrick Muldowney - Gauge Integral Structures for Stochastic Calculus and Quantum Electrodynamics
Здесь есть возможность читать онлайн «Patrick Muldowney - Gauge Integral Structures for Stochastic Calculus and Quantum Electrodynamics» — ознакомительный отрывок электронной книги совершенно бесплатно, а после прочтения отрывка купить полную версию. В некоторых случаях можно слушать аудио, скачать через торрент в формате fb2 и присутствует краткое содержание. Жанр: unrecognised, на английском языке. Описание произведения, (предисловие) а так же отзывы посетителей доступны на портале библиотеки ЛибКат.
- Название:Gauge Integral Structures for Stochastic Calculus and Quantum Electrodynamics
- Автор:
- Жанр:
- Год:неизвестен
- ISBN:нет данных
- Рейтинг книги:3 / 5. Голосов: 1
-
Избранное:Добавить в избранное
- Отзывы:
-
Ваша оценка:
Gauge Integral Structures for Stochastic Calculus and Quantum Electrodynamics: краткое содержание, описание и аннотация
Предлагаем к чтению аннотацию, описание, краткое содержание или предисловие (зависит от того, что написал сам автор книги «Gauge Integral Structures for Stochastic Calculus and Quantum Electrodynamics»). Если вы не нашли необходимую информацию о книге — напишите в комментариях, мы постараемся отыскать её.
, left off,
introduces readers to particular problems of integration in the probability-like theory of quantum mechanics. Written as a motivational explanation of the key points of the underlying mathematical theory, and including ample illustrations of the calculus, this book relies heavily on the mathematical theory set out in the author’s previous work. That said, this work stands alone and does not require a reading of
in order to be understandable.
Gauge Integral Structures for Stochastic Calculus and Quantum Electrodynamics Stochastic calculus, including discussions of random variation, integration and probability, and stochastic processes. Field theory, including discussions of gauges for product spaces and quantum electrodynamics. Robust and thorough appendices, examples, illustrations, and introductions for each of the concepts discussed within. An introduction to basic gauge integral theory. The methods employed in this book show, for instance, that it is no longer necessary to resort to unreliable «Black Box» theory in financial calculus; that full mathematical rigor can now be combined with clarity and simplicity. Perfect for students and academics with even a passing interest in the application of the gauge integral technique pioneered by R. Henstock and J. Kurzweil,
is an illuminating and insightful exploration of the complex mathematical topics contained within.

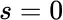 ) of the share to be
) of the share to be 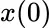 (or
(or 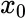 ), take the initial shareholding or number of shares owned to be
), take the initial shareholding or number of shares owned to be 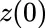 (or
(or 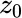 ). Then, at end of day 1 (
). Then, at end of day 1 ( 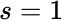 ),
),
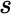 ,
,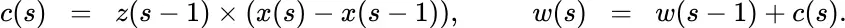
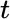 days,
days,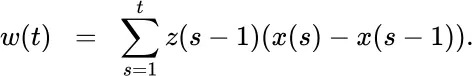
 represents number of “time ticks”—fractions of a second, say), with the meaning of the other variables adjusted accordingly, then
represents number of “time ticks”—fractions of a second, say), with the meaning of the other variables adjusted accordingly, then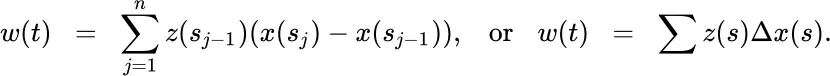
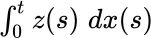 (a Stieltjes‐type integral) whenever the latter exists.
(a Stieltjes‐type integral) whenever the latter exists.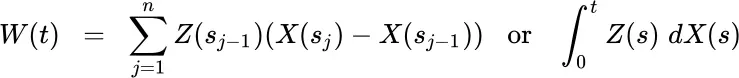
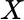 ,
, 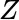 , and
, and 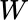 . These notations symbolize—in a “naive” or “realistic” way—the stochastic integral of the process
. These notations symbolize—in a “naive” or “realistic” way—the stochastic integral of the process 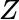 with respect to the process
with respect to the process 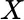 . In chapter 8of [MTRV], symbols
. In chapter 8of [MTRV], symbols 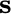 , or
, or 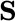 , or
, or 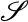 are used (in place of the symbol
are used (in place of the symbol  ) for various kinds of stochastic integral. In the context described here,
) for various kinds of stochastic integral. In the context described here, 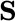 would be the appropriate notation. (See (5.28) below.)
would be the appropriate notation. (See (5.28) below.)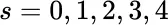 tracks the process over four days. Suppose the initial value of the share at the start of day 1 is
tracks the process over four days. Suppose the initial value of the share at the start of day 1 is 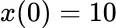 . Suppose on each day the value of the share can change by
. Suppose on each day the value of the share can change by 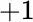 or
or 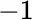 . That is, an “up” increment (U) or “down” increment (D). (Although the probabilities involved will not be used at this stage, in order to keep random variability in mind suppose that, at the end of each day, U occurs with probability
. That is, an “up” increment (U) or “down” increment (D). (Although the probabilities involved will not be used at this stage, in order to keep random variability in mind suppose that, at the end of each day, U occurs with probability 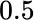 and suppose D occurs with probability
and suppose D occurs with probability 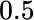 .)
.)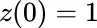 , or 1 share, and suppose the shareholder buys an extra share whenever the share value increases (U), and otherwise keeps the same number of shares. So there are no circumstances in which shareholding is decreased. (It is easy to imagine that the investor would apply a less optimistic and more prudent share purchasing strategy. But for purpose of illustration some particular strategy must be chosen, and this one is easy to describe.)
, or 1 share, and suppose the shareholder buys an extra share whenever the share value increases (U), and otherwise keeps the same number of shares. So there are no circumstances in which shareholding is decreased. (It is easy to imagine that the investor would apply a less optimistic and more prudent share purchasing strategy. But for purpose of illustration some particular strategy must be chosen, and this one is easy to describe.)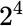 ,
,  , possible processes or histories, corresponding to the 16 possible permutations‐with‐repetition of the 2 symbols U and D, taken four at a time.
, possible processes or histories, corresponding to the 16 possible permutations‐with‐repetition of the 2 symbols U and D, taken four at a time.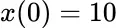 and
and 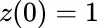 , the histories or processes of interest are prices
, the histories or processes of interest are prices  ; holdings
; holdings 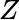 ; and total gains
; and total gains 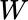 ; represented by
; represented by










