For each mineral, P‐ and S‐ wave velocities are computed using the Voigt‐Reuss‐Hill averages for bulk and shear moduli of an isotropic aggregate. The explored pressures and temperatures are spanned by two adiabatic compression paths that start 500 K above and below a typical adiabatic compression path for each mineral. To examine the impact of uncertainties on a given finite‐strain parameter, P‐ and S‐ wave velocities are then recalculated by first adding (+) and then subtracting (–) the respective uncertainty to a given parameter leaving all other parameters unchanged. The resulting difference in velocities Δ v = v +− v −is then compared to the original velocity v VRHas:
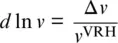
The effect of anisotropy is illustrated in the same way by using the Voigt (V) and Reuss (R) bounds on the moduli and setting Δ v = v V− v R. The velocity variations d ln v are mapped over relevant pressures and temperatures for olivine, wadsleyite, ringwoodite, and bridgmanite. Note that, for each of these minerals, the results of experiments and computations need to be substantially inter‐ and extrapolated across the respective pressure–temperature space.
Figures 3.1and 3.2show relative variations d ln v of P‐ and S‐ wave velocities, respectively, that arise from differences between the Voigt and Reuss bounds as well as from uncertainties on the parameters 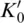 ,
, 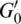 , γ 0, q 0, and η 0. Varying the Debye temperature θ 0within reported uncertainties does not change wave velocities by more than 0.5% for the minerals and conditions considered, and no corresponding plots have been included in Figures 3.1and 3.2. Parameter values and uncertainties were taken from the respective references or, in the case of San Carlos olivine, derived by fitting finite‐strain equations to the combined data set of elastic stiffness tensors and unit cell volumes at high pressures and high temperatures (Mao et al., 2015; Zha et al., 1998; Zhang & Bass, 2016). The uncertainties can therefore be considered to reflect the precision of current experimental and computational methods. The resulting variations in P‐ and S‐ wave velocities, however, display the impact of individual parameters rather than absolute uncertainties on wave velocities. This way of propagating uncertainties on individual parameters into uncertainties on wave velocities illustrates how the influence of a given parameter changes with pressure and temperature and allows to identify those parameters that exert the strongest impact on P‐ and S‐ wave velocities, respectively.
, γ 0, q 0, and η 0. Varying the Debye temperature θ 0within reported uncertainties does not change wave velocities by more than 0.5% for the minerals and conditions considered, and no corresponding plots have been included in Figures 3.1and 3.2. Parameter values and uncertainties were taken from the respective references or, in the case of San Carlos olivine, derived by fitting finite‐strain equations to the combined data set of elastic stiffness tensors and unit cell volumes at high pressures and high temperatures (Mao et al., 2015; Zha et al., 1998; Zhang & Bass, 2016). The uncertainties can therefore be considered to reflect the precision of current experimental and computational methods. The resulting variations in P‐ and S‐ wave velocities, however, display the impact of individual parameters rather than absolute uncertainties on wave velocities. This way of propagating uncertainties on individual parameters into uncertainties on wave velocities illustrates how the influence of a given parameter changes with pressure and temperature and allows to identify those parameters that exert the strongest impact on P‐ and S‐ wave velocities, respectively.
Aggregate P‐ and S‐ wave velocities of olivine, wadsleyite, and bridgmanite all show variations of more than 1% due to elastic anisotropy as reflected in the differences between the Voigt and Reuss bounds. The elastic anisotropy of ringwoodite single crystals is known to be fairly small (Mao et al., 2012; Sinogeikin et al., 1998; Weidner et al., 1984). As a result, aggregate P‐ and S‐ wave velocities differ by less than 0.5% for ringwoodite. For minerals with significant elastic anisotropy, including the major mantle minerals olivine, wadsleyite, and bridgmanite, uncertainties on wave velocities that arise from averaging over grains with different orientations may contribute substantially to absolute uncertainties as the actual elastic response of an isotropic polycrystalline aggregate may fall somewhere in between the bounds on elastic moduli. Note that alternative bounding schemes might provide tighter bounds on elastic moduli than the Voigt and Reuss bounds (Watt et al., 1976).
In comparison to the impact of elastic anisotropy on aggregate elastic moduli and wave velocities, the pressure derivatives 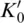 and
and 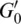 do not appear to strongly affect wave velocities when varied within reported uncertainties. This observation reflects the common approach of experimental and computational methods to address the response of elastic properties to compression and hence to best constrain pressure derivatives. To a certain extent, the comparatively small leverage of pressure derivatives on elastic wave velocities justifies inter‐ and extrapolations of the finite‐strain formalism to pressures and temperatures not covered by experiments or computations. Along adiabatic compression paths of typical mantle rocks, for example, changes in elastic properties that result from compression or volume reduction surpass changes that result from the corresponding adiabatic increase in temperature.
do not appear to strongly affect wave velocities when varied within reported uncertainties. This observation reflects the common approach of experimental and computational methods to address the response of elastic properties to compression and hence to best constrain pressure derivatives. To a certain extent, the comparatively small leverage of pressure derivatives on elastic wave velocities justifies inter‐ and extrapolations of the finite‐strain formalism to pressures and temperatures not covered by experiments or computations. Along adiabatic compression paths of typical mantle rocks, for example, changes in elastic properties that result from compression or volume reduction surpass changes that result from the corresponding adiabatic increase in temperature.
With the exception of the Debye temperature, the parameters describing the quasi‐harmonic or thermal contribution to elastic properties all show significant impact on computed wave velocities. Both P‐ and S‐ wave velocities of the minerals included in Figures 3.1and 3.2appear to be sensitive to variations in the Grüneisen parameter γ 0, in particular at low pressures and high temperatures. In general, the isotropic volume strain derivative q 0= η V0/ γ 0of the Grüneisen parameter has more impact on P‐ wave velocities while the isotropic shear strain derivative η S0mostly affects S‐ wave velocities. The S‐ wave velocities of transition zone minerals seem to be particularly sensitive to η S0. This sensitivity arises from comparatively large uncertainties on η S0for these minerals and from relatively high temperatures in the transition zone at comparatively small finite strains. The exothermic phase transitions from olivine to wadsleyite and from wadsleyite to ringwoodite are expected to raise the temperatures in the transition zone in addition to adiabatic compression (Katsura et al., 2010). For olivine and bridgmanite, adiabatic compression over extended pressure ranges somewhat mitigates the influence of thermoelastic parameters as compressional contributions to elastic moduli increase at the expense of thermal contributions.
A complete analysis of uncertainties on computed P‐ and S‐ wave velocities would include correlations between finite‐strain parameters that are, however, not regularly reported. The derivation of an internally consistent matrix of covariances between finite‐strain parameters requires coherent data sets of elastic properties at high pressures and high temperatures, i.e., data sets than can be simultaneously inverted for all relevant finite‐strain parameters. Due to experimental challenges in performing measurements of sound wave velocities at combined high pressures and high temperatures, however, finite‐strain parameters for most mantle minerals have been derived from separate but complementary data sets. Elastic moduli and their pressure derivatives are often obtained from sound wave velocity measurements at high pressures but ambient temperatures. Thermoelastic parameters are then independently derived from a thermal EOS, from the results of separate measurements at high temperatures, or from a computational study. This approach does not always generate data sets that can be combined and jointly inverted for complete and consistent sets of finite‐strain parameters and their covariances. As a consequence, the results of different studies are combined in terms of finite‐strain parameters that have been derived from separate data sets. As an example, the inversion of the combined data set of elastic stiffness tensors and unit cell volumes of San Carlos olivine that have been determined in separate studies at high pressures (Zha et al., 1998) and at combined high pressures and high temperatures (Mao et al., 2015; Zhang and Bass, 2016) required fixing the components of the Grüneisen tensor γ ii0and the Debye temperature θ 0in order to stabilize the inversion results. Correlations between finite‐strain parameters can be reduced by optimizing the sampling of the relevant volume–temperature space. Future studies that provide consistent elasticity data sets at combined high pressures and high temperatures will allow for analyzing parameter correlations and help to reduce uncertainties in computed P‐ and S‐ wave velocities by integrating covariances into the propagation of uncertainties.
Читать дальше

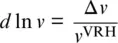
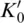 ,
, 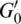 , γ 0, q 0, and η 0. Varying the Debye temperature θ 0within reported uncertainties does not change wave velocities by more than 0.5% for the minerals and conditions considered, and no corresponding plots have been included in Figures 3.1and 3.2. Parameter values and uncertainties were taken from the respective references or, in the case of San Carlos olivine, derived by fitting finite‐strain equations to the combined data set of elastic stiffness tensors and unit cell volumes at high pressures and high temperatures (Mao et al., 2015; Zha et al., 1998; Zhang & Bass, 2016). The uncertainties can therefore be considered to reflect the precision of current experimental and computational methods. The resulting variations in P‐ and S‐ wave velocities, however, display the impact of individual parameters rather than absolute uncertainties on wave velocities. This way of propagating uncertainties on individual parameters into uncertainties on wave velocities illustrates how the influence of a given parameter changes with pressure and temperature and allows to identify those parameters that exert the strongest impact on P‐ and S‐ wave velocities, respectively.
, γ 0, q 0, and η 0. Varying the Debye temperature θ 0within reported uncertainties does not change wave velocities by more than 0.5% for the minerals and conditions considered, and no corresponding plots have been included in Figures 3.1and 3.2. Parameter values and uncertainties were taken from the respective references or, in the case of San Carlos olivine, derived by fitting finite‐strain equations to the combined data set of elastic stiffness tensors and unit cell volumes at high pressures and high temperatures (Mao et al., 2015; Zha et al., 1998; Zhang & Bass, 2016). The uncertainties can therefore be considered to reflect the precision of current experimental and computational methods. The resulting variations in P‐ and S‐ wave velocities, however, display the impact of individual parameters rather than absolute uncertainties on wave velocities. This way of propagating uncertainties on individual parameters into uncertainties on wave velocities illustrates how the influence of a given parameter changes with pressure and temperature and allows to identify those parameters that exert the strongest impact on P‐ and S‐ wave velocities, respectively.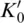 and
and 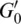 do not appear to strongly affect wave velocities when varied within reported uncertainties. This observation reflects the common approach of experimental and computational methods to address the response of elastic properties to compression and hence to best constrain pressure derivatives. To a certain extent, the comparatively small leverage of pressure derivatives on elastic wave velocities justifies inter‐ and extrapolations of the finite‐strain formalism to pressures and temperatures not covered by experiments or computations. Along adiabatic compression paths of typical mantle rocks, for example, changes in elastic properties that result from compression or volume reduction surpass changes that result from the corresponding adiabatic increase in temperature.
do not appear to strongly affect wave velocities when varied within reported uncertainties. This observation reflects the common approach of experimental and computational methods to address the response of elastic properties to compression and hence to best constrain pressure derivatives. To a certain extent, the comparatively small leverage of pressure derivatives on elastic wave velocities justifies inter‐ and extrapolations of the finite‐strain formalism to pressures and temperatures not covered by experiments or computations. Along adiabatic compression paths of typical mantle rocks, for example, changes in elastic properties that result from compression or volume reduction surpass changes that result from the corresponding adiabatic increase in temperature.










