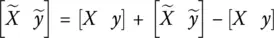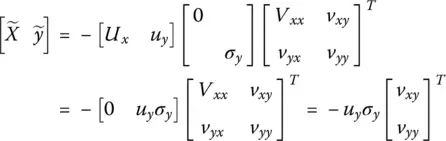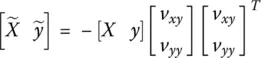Magdalena Salazar-Palma - Modern Characterization of Electromagnetic Systems and its Associated Metrology
Здесь есть возможность читать онлайн «Magdalena Salazar-Palma - Modern Characterization of Electromagnetic Systems and its Associated Metrology» — ознакомительный отрывок электронной книги совершенно бесплатно, а после прочтения отрывка купить полную версию. В некоторых случаях можно слушать аудио, скачать через торрент в формате fb2 и присутствует краткое содержание. Жанр: unrecognised, на английском языке. Описание произведения, (предисловие) а так же отзывы посетителей доступны на портале библиотеки ЛибКат.
- Название:Modern Characterization of Electromagnetic Systems and its Associated Metrology
- Автор:
- Жанр:
- Год:неизвестен
- ISBN:нет данных
- Рейтинг книги:4 / 5. Голосов: 1
-
Избранное:Добавить в избранное
- Отзывы:
-
Ваша оценка:
Modern Characterization of Electromagnetic Systems and its Associated Metrology: краткое содержание, описание и аннотация
Предлагаем к чтению аннотацию, описание, краткое содержание или предисловие (зависит от того, что написал сам автор книги «Modern Characterization of Electromagnetic Systems and its Associated Metrology»). Если вы не нашли необходимую информацию о книге — напишите в комментариях, мы постараемся отыскать её.
Presents modern computational concepts in electromagnetic system characterization Describes a solution to the generation of non-minimum phase from amplitude-only data Covers model-based parameter estimation and planar near-field to far-field transformation as well as spherical near-field to far-field transformation
is ideal for graduate students, researchers, and professionals working in the area of antenna measurement and design. It introduces and explains a new process related to their work efforts and studies.

 ( x ; a ) as well as the independent variables X . Considering the errors of the measured data vector, y , and the independent variables, X , (1.25)can be re‐written as
( x ; a ) as well as the independent variables X . Considering the errors of the measured data vector, y , and the independent variables, X , (1.25)can be re‐written as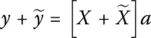
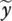 and
and 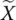 are the errors in both the dependent variable measurements and independent variable measurements, respectively. We then want to approximate in a way that minimizes these errors in the dependent and independent variables. This can be expressed by,
are the errors in both the dependent variable measurements and independent variable measurements, respectively. We then want to approximate in a way that minimizes these errors in the dependent and independent variables. This can be expressed by,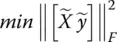
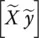 is an augmented matrix with the columns of error matrix
is an augmented matrix with the columns of error matrix 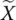 concatenated with the error vector
concatenated with the error vector  . The operator ‖•‖ Frepresents the Frobenius norm of the augmented matrix. The Frobenius norm is defined as the square root of the sum of the absolute squares of all of the elements in a matrix. This can be expressed in equation form as the following, where A is any matrix,
. The operator ‖•‖ Frepresents the Frobenius norm of the augmented matrix. The Frobenius norm is defined as the square root of the sum of the absolute squares of all of the elements in a matrix. This can be expressed in equation form as the following, where A is any matrix,

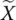 ; y +
; y + 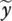 ] must have n linearly independent columns. However, this matrix has n + 1 columns in total and therefore is rank is deficient by 1. We then must find the smallest matrix [
] must have n linearly independent columns. However, this matrix has n + 1 columns in total and therefore is rank is deficient by 1. We then must find the smallest matrix [ 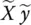 ] that changes matrix [ X y ] with a rank of n + 1, to a matrix {[ X y ] + [
] that changes matrix [ X y ] with a rank of n + 1, to a matrix {[ X y ] + [  ]} with a rank n . According to the Eckart‐Young‐Mirsky theorem we can achieve this by defining {[ X y ] + [
]} with a rank n . According to the Eckart‐Young‐Mirsky theorem we can achieve this by defining {[ X y ] + [ 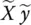 ]} as the best rank‐ n approximation to [ X y ] and by eliminating the last singular value of [ X y ] which contains the least amount of system information and provides a unique solution. The Eckart–Young–Mirsky theorem ( https://en.wikipedia.org/wiki/Low‐rank_approximation) states a low‐rank approximation is a minimizationproblem, in which the cost functionmeasures the fit between a given matrix (the data) and an approximating matrix (the optimization variable), subject to a constraint that the approximating matrix has reduced rank. To illustrate how this is accomplished, we take the SVD of [ X y ] as follows
]} as the best rank‐ n approximation to [ X y ] and by eliminating the last singular value of [ X y ] which contains the least amount of system information and provides a unique solution. The Eckart–Young–Mirsky theorem ( https://en.wikipedia.org/wiki/Low‐rank_approximation) states a low‐rank approximation is a minimizationproblem, in which the cost functionmeasures the fit between a given matrix (the data) and an approximating matrix (the optimization variable), subject to a constraint that the approximating matrix has reduced rank. To illustrate how this is accomplished, we take the SVD of [ X y ] as follows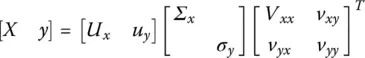
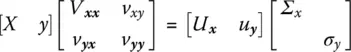
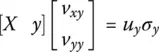
 ]} is the closest rank‐ n approximation to [ X y ]. Matrix {[ X y ] + [
]} is the closest rank‐ n approximation to [ X y ]. Matrix {[ X y ] + [ 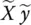 ]} has the same singular vectors contained in ∑ xabove with σ yequal to zero. We can then write the SVD of {[ X y ] + [
]} has the same singular vectors contained in ∑ xabove with σ yequal to zero. We can then write the SVD of {[ X y ] + [ 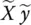 ]} as such
]} as such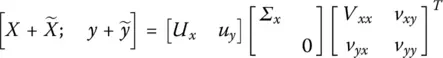
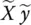 ] we must solve the following
] we must solve the following