Generalized Ordinary Differential Equations in Abstract Spaces and Applications
Здесь есть возможность читать онлайн «Generalized Ordinary Differential Equations in Abstract Spaces and Applications» — ознакомительный отрывок электронной книги совершенно бесплатно, а после прочтения отрывка купить полную версию. В некоторых случаях можно слушать аудио, скачать через торрент в формате fb2 и присутствует краткое содержание. Жанр: unrecognised, на английском языке. Описание произведения, (предисловие) а так же отзывы посетителей доступны на портале библиотеки ЛибКат.
- Название:Generalized Ordinary Differential Equations in Abstract Spaces and Applications
- Автор:
- Жанр:
- Год:неизвестен
- ISBN:нет данных
- Рейтинг книги:4 / 5. Голосов: 1
-
Избранное:Добавить в избранное
- Отзывы:
-
Ваша оценка:
Generalized Ordinary Differential Equations in Abstract Spaces and Applications: краткое содержание, описание и аннотация
Предлагаем к чтению аннотацию, описание, краткое содержание или предисловие (зависит от того, что написал сам автор книги «Generalized Ordinary Differential Equations in Abstract Spaces and Applications»). Если вы не нашли необходимую информацию о книге — напишите в комментариях, мы постараемся отыскать её.
Explore a unified view of differential equations through the use of the generalized ODE from leading academics in mathematics Generalized Ordinary Differential Equations in Abstract Spaces and Applications
Generalized Ordinary Differential Equations in Abstract Spaces and Applications

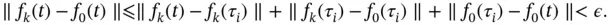
 and, therefore,
and, therefore, 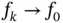 uniformly on
uniformly on  .
.
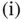 . By hypothesis, the sequence
. By hypothesis, the sequence  converges uniformly to
converges uniformly to  . Then, Moore–Osgood theorem (see, e.g., [19]) implies
. Then, Moore–Osgood theorem (see, e.g., [19]) implies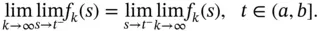
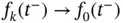 , for
, for  . In a similar way, one can show that
. In a similar way, one can show that  , for every
, for every 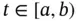 .
. . It suffices to show that
. It suffices to show that 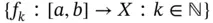 is an equiregulated set. Indeed, by Lemma 1.13,
is an equiregulated set. Indeed, by Lemma 1.13, 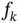 converges uniformly to
converges uniformly to 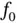 .
.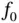 is regulated, its lateral limits exist. Then, for every
is regulated, its lateral limits exist. Then, for every 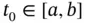 and every
and every 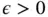 , there is
, there is 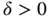 such that
such that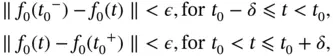
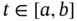 .
. such that, for
such that, for 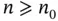 , we have
, we have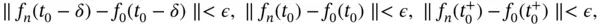
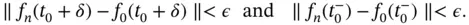
 satisfies
satisfies  , we have, for every
, we have, for every  ,
,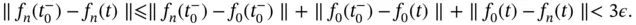
 satisfies
satisfies  , we get, for
, we get, for 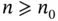 ,
,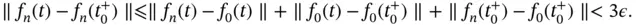
 is an equiregulated sequence, and the proof is complete.
is an equiregulated sequence, and the proof is complete.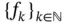 is bounded by an equiregulated sequence of functions, then
is bounded by an equiregulated sequence of functions, then 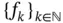 is also equiregulated.
is also equiregulated.










