Artificial Intelligence for Renewable Energy Systems

With the increase in magnetizing reactance xmq along q -axis, real part of stator eigenvalue of three- and six-phase generator was decreased by 34.4% and 68.8% (with no variation in stator eigenvalue I), as shown in Figures 1.20aand 1.21a, respectively. A major effect was noted from rotor side. Value of real part of rotor eigenvalue is increasing linearly for both three-phase and six-phase generator. It may be noted that the generator operation becomes unstable at higher value of xmq due to positive value of real part of rotor eigenvalue, as shown in Figure 1.21b. Hence, six-phase generator is more sensitive toward the variation of magnetizing reactance xmq . Also, a linear increase in the value of real eigenvalue II was noted for both three- and six-phase generator as shown in Figures 1.20cand 1.21d, respectively. But in three-phase generator, a higher decrease in real eigenvalue III was found in comparison with six-phase generator as shown in Figures 1.20dand 1.21e, respectively. No variation in real eigenvalue I was found for both three- and six-phase generator, as shown in Figures 1.20band 1.21c, respectively.

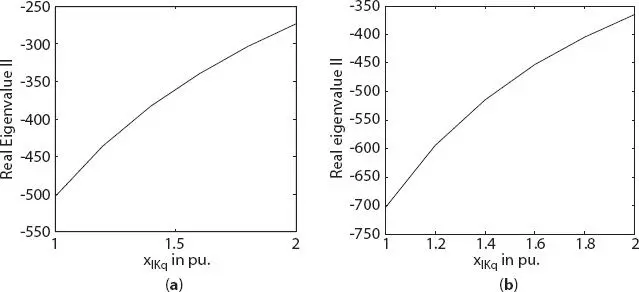
Figure 1.19 Variation in eigenvalue II with damper leakage reactance change along q -axis in (a) three-phase and (b) six-phase generator.

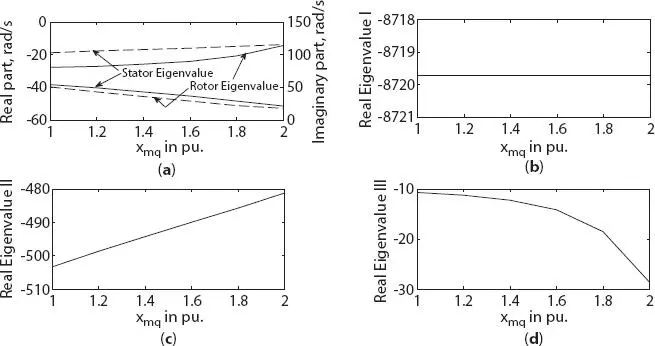
Figure 1.20 Variation in eigenvalue of three-phase synchronous machine with magnetizing reactance change along q -axis (a) stator and rotor eigenvalue, (b) real eigenvalue I, (c) real eigenvalue II, (d) real eigenvalue III.

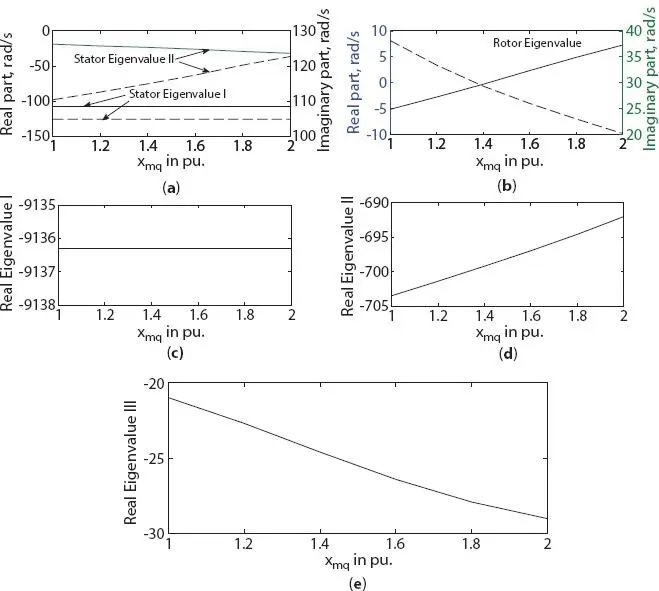
Figure 1.21 Variation in eigenvalue of six-phase synchronous machine with magnetizing reactance change along q -axis (a) stator eigenvalue I and II, (b) rotor eigenvalue, (c) real eigenvalue I, (d) real eigenvalue II, (e) real eigenvalue III.

Both three- and six-phase generator evaluated eigenvalues were found to unchanged with the variation in magnetizing reactance xmd along d -axis. Hence, it is not presented.

Considering the constant grid voltage at 240 V (rms value per phase), eigenvalue of both three- and six-phase generator is plotted in Figure 1.18for increase in load from 0.25 to 1.0 pu. From stator side, real component of stator eigenvalue decreases (increase in magnitude with negative sign) for both three- and six-phase generator, as shown in Figure 1.22a. (No variation was noted in stator eigenvalue I, hence not shown). Decrease in the value of real part of stator eigenvalue was higher (70.8%) than its three-phase counterpart (47.9%). Hence, tendency toward stability of six-phase generator is higher than three-phase generator. On rotor side, increase (i.e., decrease in magnitude with negative sign) in real component was noted to be same (20.7%) for both three- and six-phase generator as shown in Figure 1.22b. Hence, under load variation, rotor behavior will be the same for both three- and six-phase generator. On real eigenvalue II, a slight increase in the value for three-phase (by 4.5%) and six-phase generator (by 1.5%) was noted, as shown in Figure 1.22c. Also, a very small decrease in real eigenvalue III for six-phase (by 3.2%) was noted with 17.1% variation in three-phase generator as shown in Figure 1.22d. Hence, both rotor and damper winding behavior will be approximately the same for both three- and six-phase generator. Since, variation in real eigenvalue I was not noted, and hence not shown.

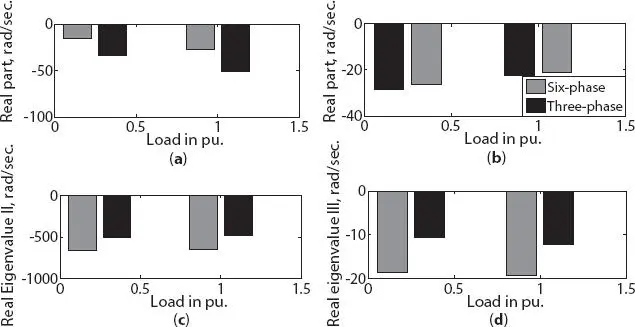
Figure 1.22 Change in real/real component of generator eigenvalue due to load variation. (a) stator eigenvalue, (b) rotor eigenvalue, (c) real eigenvalue II, (d) real eigenvalue III.

This chapter deals with a detailed analysis of six-phase synchronous generator, applicable for wind power generation system. This includes the mathematical modeling of grid connected six-phase synchronous generator and its dynamic performance under load variation. Initially, machine was operated at no-load followed by the step increase in output power (i.e., active power increased at time t = 5 and 15 s from no-load to 50% of rated, to rated value). Hence, increase in active component (i.e., q -axis component) of current was noted to be increased with no change in reactive component (i.e., d -axis component) current, associated with both the three-phase stator winding. This has resulted in an overall increase in current of both stator winding sets. On the basis of performance results, it may be inferred that a stable operation is achieved with a step change in output power for a grid connected six-phase synchronous generator.

Furthermore, a detailed small signal stability analysis was presented for grid connected six-phase synchronous generator with its three-phase counterpart. For this purpose, a linearized mathematical model was developed using Park’s variables, where eigenvalue criteria were used to access the stability under small disturbance. A larger effect was found with the change in stator resistance on both six-phase and three-phase generator. Six-phase generator was found to be more stable due to larger magnitude of negative component of both stator and rotor eigenvalues. Variation of stator leakage reactance also affects the machine stability. It was found that from rotor side, six-phase generator operation is tending toward stability, while three-phase generator is approaching toward instability. Hence, machine operation will remain more stable under stator leakage reactance variation in comparison with three-phase generator. On the field side, major effect was noted in smallest real eigenvalue III, where under variation in resistance rfr and leakage reactance xlfr , wherein six-phase generator remains stable with respect to its three-phase counterpart. From damper side also, operation of six-phase generator was found to have higher stability when compared with its three-phase equivalent.

Hence, stability of generator operation can be enhanced with increased values of stator resistance and, on rotor side, with higher leakage reactance value of field winding circuit or/and by increased damper winding resistance along q axis.

1. Singh, G.K., Self-excited induction generator research—a survey. Electr. Power Syst. Res ., 69, 2, 107–114, 2004.

2. Chinmaya, K.A. and Singh, G.K., Performance evaluation of multiphase induction generator in stand-alone and grid connected wind energy conversion system. IET Renewable Power Gener ., 12, 7, 823–83, 2018.

3. https://gwec.net/global-figures/wind-energy-global-status

4. Singh, G.K., Multi-phase induction machine drive research survey. Electr. Power Syst. Res ., 61, 2, 139–147, 2002.

5. Levi, E., Multiphase electric machines for variable speed applications. IEEE Trans. Ind. Electron ., 55, 5, 1893–909, 2008.