Paul J. van Leeuwen - Quantum Physics is NOT Weird

Such scientists exist, but they seem to be in a minority.

By the way, Planck only supposed discrete energy transfer between the walls of the Black Body emitter. He did not theorize about speeding light particles (photons) as Einstein would do later in his publication of the photoelectric effect. As you will see later in this book, Einstein's light particles do lead a very dubious existence.

“As far as the laws of mathematics refer to reality, they are not certain, and as far as they are certain, they do not refer to reality”

Albert Einstein, physicist 1879-1955

In this chapter I start with a more extensive treatment of the character and behavior of different types of waves – standing waves in particular– in different mediums, in order to understand that they all have very common characteristics. Before the end of the nineteenth century physicists were already very proficient in mathematics describing the behavior of every known type of wave. Applying their wave mathematics to the quantum world seemed an obvious choice and proved itself to become so highly apt for creating a quantum theory, that quantum physics would become the most successful and accurate physics theory in the 20 thcentury.

But before quantum theory was firmly established many questions concerning the atom and its behavior had to be solved. And every inspired solution generated more questions than it had solved. Newtonian physics seemed poised to slide away into unpredictable depths.

Einstein used Planck’s idea of discrete energy packets to solve the riddle as to why only light above certain frequencies could free electrons from metal and was awarded a Nobel prize. His solution was however in paradoxical contradiction with Maxwell’s EM-waves. Louis De Broglie, a French prince, suggested standing electron waves around the atom nucleus, which was later confirmed, serendipitously, by electron interference. But how is it possible for an electron be a particle and a wave at the same time? Is it possible to reconcile these disparate views?

From the introduction of Maxwell’s equations for electromagnetic waves, three major different types of waves [1]were distinguished in classical physics. For understanding the quantum physical wave phenomena that will be discussed in the coming chapters it is a good idea to delve a little bit deeper into the subject of waves at this point as wave behavior plays an extremely important role in quantum physics.

Surface waves propagate in liquids. The movement of the particles in waves traveling along the liquid surface is roughly perpendicular to the surface. The particles move only a little back and forth in the propagation direction of the wave. The wave transports no liquid in the direction of propagation. This type of wave is easy to recognize visually. It is also easy to generate them, throwing a pebble in a pond is sufficient. In figure 4.1 the movement of the liquid particles is indicated by ellipses. The deeper under the surface, the smaller the ellipses, the less the liquid moves. A diver will notice this when swimming a little below a troubled surface. Every fluid particle – including those at the surface – moves not very far from its equilibrium position. Surface waves that intersect with each other exhibit superposition and interference, they reinforce or cancel each other out for a moment and then roll on again.

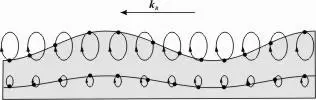
Figure 4.1: The movement of particles in a liquid transporting a surface wave. Source: Mpasternak on Wikimedia Commons.

Sound waves are propagations[2] of pressure fluctuations in gases, liquids, and solids. Their constituent particles – atoms or molecules – oscillate in the same direction as the propagation of the wave. The superposition principle also applies to sound waves, so they will also show interference. Interference of sound waves with slightly differing frequencies, will be experienced as overtones. Sound waves are not experienced as oscillations but as tones. Healthy, young ears can experience sound frequencies between 20 and 20,000 Hertz.

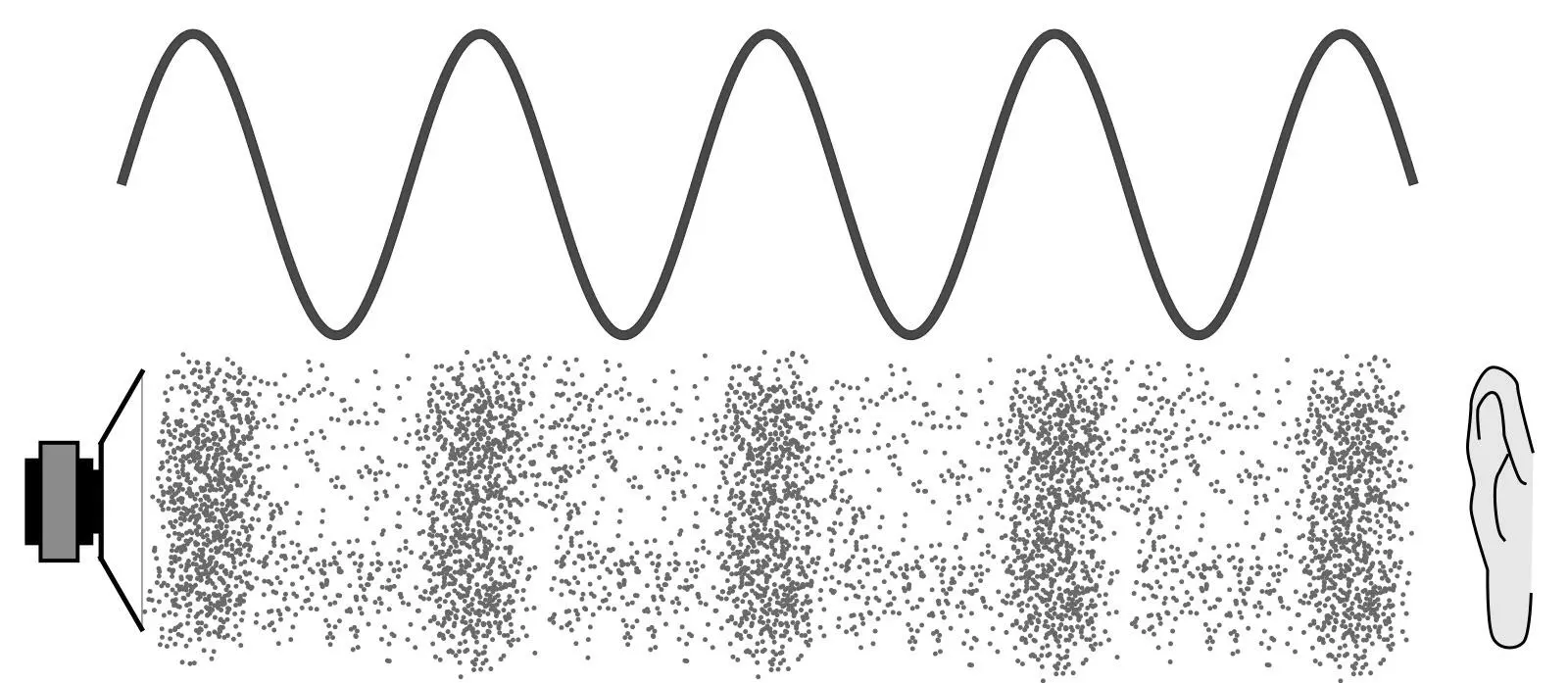
Figure 4.2: A sound wave propagating compression variations. Source: Pluke on Wikimedia Commons

Electromagnetic waves consist of oscillating electric and magnetic fields aligned perpendicular to the wave direction and also perpendicular to each other. See figure 3.7 on page 44. We experience electromagnetic waves between 400 THz and 790 THz (Terahertz: 1 THz = 1,000,000,000,000 Hz) as visible light. We do not experience the wave behavior of light as oscillations but as colors. EM-waves also exhibit interference and superposition.

Modern physics has also identified other types of waves, e.g., gravitational waves and quantum waves; we will deal extensively with those quantum waves in the following chapters. For gravitational waves I must refer you to other literature.

All these types of waves exhibit refraction, which occurs when a wave propagates into another, slower, medium at an oblique angle with the boundary. Note that the level of abstraction increases in the above-mentioned order – liquid, sound, and EM-waves. It is easy for us to see the everyday occurrence of waves on water. We hear sound waves, but we do not see them neither do we experience the pressure variations as oscillations. But we are still able to visualize the propagating pressure variations in our imagination.

However, trying to visualize oscillating magnetic and electric fields becomes rather difficult because those waves do not need matter for their propagation. We will see that EM-waves seem more like clouds of unimaginable small non-material particles of pure energy traveling through empty space with light speed. We will see eventually that that is also an untenable position.

Note: The quantum wave is something that we never observe as a material wave. This will become important in the unmasking of the often-advocated particle-wave duality.

In 1905, in his Annus Mirabilis, Albert Einstein(1879-1955) published four very important physics articles, presenting groundbreaking theories concerning:

special relativity[3]; about time and space effects between uniformly – that is not changing in velocity – moving systems,

the Brownian motion[4]; where he convincingly demonstrated the existence of the molecules and their movement in a liquid, which manifests itself as heat,

the photoelectric effect[5]; explaining the way light of sufficient high frequency can free electrons from metal and why light of lower frequencies is not able to do that,

the equivalence of energy and mass[6]; a relation derived from the special relativity theory.

In 1921 Einstein received the Nobel Prize for his explanation of the photoelectric effect. He explained that effect with an adaptation of the Planck Black Body radiation law. He realized that he could treat the radiation within the hollow space of a Black Body emitter as if it was a gas of light particles [ 7 ]. His adaptation was based on the statistical derivation of the gas laws, formulated by Ludwig Boltzmann (1844-1906) in 1884. Boltzmann’s statistical approach could be applied to Einstein's gas of light particles model with some slight adjustments.

Boltzmann’s classic statistical gas laws assumed enormous numbers of very small and fast-moving and colliding particles. Initially Boltzmann's statistical approach was not received favorably by many of his colleagues because, according to them, there was no place for fuzzy concepts as chance and probability in Newtonian mechanics. Tragically, he did not enjoy the enormous success of his statistical approach. Boltzmann was manic-depressive. In 1906 he fell into a deep depression leading to suicide.