Julian Barbour - The End of Time - The Next Revolution in Physics

To come straight to the point, it soon became clear in the discussions with Karel that the idea of best matching and the whole way of thinking about duration as a measure of difference were already both contained within the mathematics of general relativity, though not in a transparent form. These facts are still not widely known, mainly, I think, because of a certain inertia. General relativity was discovered as a theory of four-dimensional space-time, and that is still essentially the way it is presented. The fact that it is simultaneously a dynamical theory describing the changes of three-dimensional things is given much less weight. This is why so few people are aware that there is such a deep issue and crisis about the nature of time at the heart of general relativity.

I think that the nature of the problem can be explained to a non-scientist. Here, at least, is my attempt. Figure 29 is a very schematic representation of the three different kinds of four-dimensional space-time that have been considered in this book. As usual, only one of the three dimensions of space is shown. It and its material contents are represented by the horizontal direction, while time runs vertically. Thus, the more or less horizontal lines and curves in the three parts of the diagram represent space and its material contents at different ‘times’. They are each Nows in my sense. As we have seen, Newtonian space-time is like a pack of ordinary cards. Each card is a Now, and they are all horizontal. I called Minkowski space-time a magical pack of cards because its Nows, or hyperplanes of simultaneity, can be drawn in different ways. Depending on the Lorentz frame that is chosen, different families of parallel Nows are obtained. Time has become relative to the frame. In general relativity, this relativity of time is taken much further: provided the Nows do not cut through the light cone, they can be drawn in an immense number of different ways. It is the complete absence of uniqueness in the way this is done that led Einstein to comment that the concept of Now does not exist in modern physics. However, this reflects the space-time viewpoint. The dynamical viewpoint puts things in a different perspective.

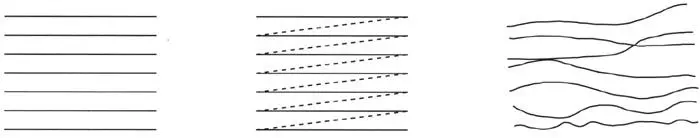
Figure 29.The three different kinds of space-time: on the left, Newtonian space-time, with ‘horizontal’ Nows; in the middle, Minkowski space-time, with alternative ‘tilted’ Nows; on the right, the space-time of general relativity, with Nows running in arbitrary directions.

To see this, suppose we consider two neighbouring Nows, as shown in Figure 30, in a space-time that satisfies the equations of general relativity. Each Now is a 3-space with its own intrinsic three-dimensional geometry and material contents embedded within space-time. This four-dimensional space-time has its own geometry too, and permits the construction of ‘struts’ between the two Nows. The struts are the world lines of bodies that follow geodesics in space-time, leaving the earlier Now along the space-time direction that is perpendicular to it at the point of departure. Each ‘strut’ is, so to speak, erected on the first Now. It will pierce the second Now at some point. Taken altogether, such struts uniquely determine a pairing of each point of the first Now with a point of the second Now. They do something else, too. If a clock travels along each strut between its two ends, it will measure the proper time between them as it goes. Because the two Nows have been chosen arbitrarily, the proper time will in general be different for each strut.

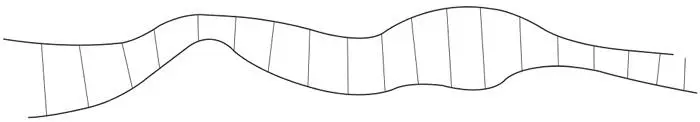
Figure 30.The two continuous curves represent (in one dimension) the two slightly different 3-spaces mentioned in the text; the more or less vertical lines are the ‘struts’.

What has this to do with best matching? Everything. Imagine mean-minded mathematicians who stick ‘pins’ like those that I stuck into Tristan and Isolde into the two 3-spaces to identify the two ends of all the struts in Figure 30. The pins carry little flags with the ‘lengths’ of the corresponding struts – the proper time – along them. However, all this information, which tells us exactly how the two 3-spaces are positioned relative to each other in space-time, is made invisible to other mathematicians who are ‘given’ just the two 3-spaces, the Nows with their intrinsic geometries and matter distributions, and set the task of finding the struts’ positions and lengths. Will they succeed?

Despite niggling qualifications, the answer is yes. When you unpack the mathematics of Einstein’s theory and see how it works from the point of view of geometrodynamics, it appears to have been tailor-made to solve this problem. This was shown in 1962 in a remarkable, but not very widely known paper of just two pages by Ralph Baierlein, David Sharp and John Wheeler (the first two were students of Wheeler at Princeton). I shall refer to these authors, whose paper has the somewhat enigmatic title ‘Three-dimensional geometry as a carrier of information about time’, as BSW. Initials can become a menace, but the BSW paper is so central to my story that I think they are warranted.

It is the implications of the BSW paper that I discussed with Karel in 1980. They can be quickly summarized. The basic problem that BSW considered was what kind of information, and how much, must be specified if a complete space-time is to be determined uniquely. This is exactly analogous to the question that Poincaré asked in connection with Newtonian dynamics, and then showed that the information in three Nows was needed. As we have seen, a theory will be Machian if two Nows are sufficient. What BSW showed is that the basic structure of general relativity meets this requirement.

In fact, the all-important Einstein equation that does the work is precisely a statement that a best-matching condition between the two 3-spaces does hold. The pairing of points established by it is exactly the pairing established by the orthogonal struts. In fact, the key geometrical property of space-times that satisfy Einstein’s equations reflects an underlying principle of best matching built into the foundations of the theory. I think that Einstein, with his deep conviction that nature is supremely rational, would have been most impressed had he lived to learn about it.

Equally beautiful and interesting is the condition that determines ‘how far apart in time’ the 3-spaces are. It is closely analogous to the rule by which duration can be introduced as a distinguished simplifier in Machian dynamics and the method by which the astronomers introduced ephemeris time. There is, however, an important difference. In the simple Machian case, the distinguished simplifier creates the same ‘time separation’ across the whole of space. In Einstein’s geometrodynamics, the separation between the 3-spaces varies from point to point, but the principle that determines it is a generalization, now applied locally, of the principle that works in the Newtonian case and explains how people can keep appointments. This is why I say that, quite unbeknown to him, Einstein put a theory of Mach’s principle and duration at the heart of his theory.

I go further. The equivalence principle too is very largely explained by best matching. To model the real universe, the 3-spaces must have matter distributions within them. The analogue in two dimensions is markings on bodies or paintings on curved surfaces. When we go through the best-matching procedure, sticking pins into Isolde, it is not only points on her skin that are matched to points on Tristan, but also any tattoos or other decorative markings. All these decorations – matter in the real universe – contribute with the geometry in determining the best-matching position and the distinguished simplifier that holds the 3-spaces apart and creates proper time between them. When this idea is combined with the relativity requirement, the equivalence principle comes out more or less automatically.