In 1992, based on these findings, the International Union of Crystallography changed the definition of a crystal toward uniting the regular crystals and quasicrystals under single title with an emphasis on the similarity of diffraction phenomena: “A material is a crystal if it has essentially a sharp diffraction pattern. The word essentially means that most of the intensity of the diffraction is concentrated in relatively sharp Braggpeaks, besides the always present diffuse scattering.” In 2011, Dan Shechtmanwas awarded Nobel Prize in Chemistry “for the discovery of quasicrystals.”
1.1 Crystal Symmetry in Real Space
Across this book, we will focus on physical properties of regular crystals, amorphs and quasicrystals being out of our scope here. Thinking on conventional crystals, we first keep in mind their translational symmetry. As we already mentioned, the long-range periodic order in crystals leads to translational symmetry, which is commonly described in terms of Bravaislattices (named after French crystallographer Auguste Bravais):
(1.1) 
The nodes, r s, of Bravaislattice are produced by linear combinations of three non-coplanar vectors, a 1 , a 2 , a 3, called translation vectors. The integer numbers in Eq. (1.1)can be positive, negative, or zero. Atomic arrangements within every crystal can be described by the set of analogous Bravaislattices.
Classification of Bravaislattices is based on the relationships between the lengths of translation vectors, | a 1| = a , | a 2| = b , | a 3| = c and the angles, α , β , γ , between them. In fact, all possible types of Bravaislattices can be attributed to seven symmetry systems:
Triclinic : a ≠ b ≠ c and α ≠ β ≠ γ ;
Monoclinic : a ≠ b ≠ c and α = β = 90°, γ ≠ 90°; in this setting, angle γ is between translation vectors a 1(| a 1| = a ) and a 2(| a 2| = b ); whereas the angles α and β are, respectively, between translation vectors a 2^ a 3and a 1^ a 3;
Orthorhombic : a ≠ b ≠ c and α = β = γ = 90°;
Tetragonal : a = b ≠ c and α = β = γ = 90°;
Cubic : a = b = c and α = β = γ = 90°;
Rhombohedral : a = b = c and α = β = γ ≠ 90°;
Hexagonal : a = b ≠ c and α = β = 90°, γ = 120°.
A parallelepiped built by the aid of vectors a 1, a 2, a 3is called a unit cell and is the smallest block, which being duplicated by the translation vectors allows us to densely fill the 3D space without voids.
Translational symmetry, however, is only a part of the whole symmetry in crystals. Atomic networks, described by Bravaislattices, also possess the so-called local (point) symmetry, which includes lattice inversion with respect to certain lattice points, mirror reflections in some lattice planes, and lattice rotations about certain rotation axes (certain crystallographic directions). After application of all these symmetry elements, the lattice remains invariant. Furthermore, rotation axes are defined by their order, n . The latter, in turn, determines the minimum angle, 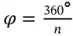 , after rotation by which the lattice remains indistinguishable with respect to its initial setting (lattice invariance). In regular crystals, the permitted rotation axes, i.e. those matching translational symmetry (see Appendix 1.A), are twofold (180°-rotation, n = 2), threefold (120°-rotation, n = 3), fourfold (90°-rotation, n = 4), and sixfold (60°-rotation, n = 6). Of course, onefold, i.e. 360°-rotation ( n = 1), is a trivial symmetry element existing in every Bravaislattice. The international notations for these symmetry elements are:
, after rotation by which the lattice remains indistinguishable with respect to its initial setting (lattice invariance). In regular crystals, the permitted rotation axes, i.e. those matching translational symmetry (see Appendix 1.A), are twofold (180°-rotation, n = 2), threefold (120°-rotation, n = 3), fourfold (90°-rotation, n = 4), and sixfold (60°-rotation, n = 6). Of course, onefold, i.e. 360°-rotation ( n = 1), is a trivial symmetry element existing in every Bravaislattice. The international notations for these symmetry elements are: 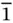 – for inversion center, m – for mirror plane, and 1 , 2 , 3 , 4 , 6 – for respective rotation axes. We see that fivefold rotation axis and axes of the order, higher than n = 6, are incompatible with translational symmetry (see Appendix 1.A).
– for inversion center, m – for mirror plane, and 1 , 2 , 3 , 4 , 6 – for respective rotation axes. We see that fivefold rotation axis and axes of the order, higher than n = 6, are incompatible with translational symmetry (see Appendix 1.A).
To deeper understand why some rotation axes are permitted, while others not, let us consider the covering of the 2D space by regular geometrical figures, having n equal edges and central angle,  ( Figure 1.4). Correspondingly, the angle, δ , between adjacent edges is:
( Figure 1.4). Correspondingly, the angle, δ , between adjacent edges is:
(1.2) 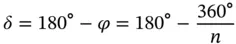
To produce a pattern without voids by using these figures, we require that the full angle around each meeting point, M, defined by p adjacent figures, should be 360°, i.e. p · δ = 360°. Therefore, using Eq. (1.2)yields:
(1.3) 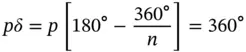
or
(1.4) 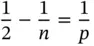

Figure 1.4Dense filling of 2D space by regular geometrical figures.
Finally, we obtain:
(1.5) 
It follows from Eq. (1.5)that there is a very limited set of regular figures (with 2 < n ≤ 6) useful for producing periodic patterns, which fill the 2D space with no voids (i.e. providing integer numbers, p ). These are hexagons ( n = 6, p = 3, ϕ = 60°), squares ( n = 4, p = 4, ϕ = 90°), and triangles ( n = 3, m = 6, ϕ = 120°). Based on the value of central angle, ϕ , these regular figures possess the sixfold, fourfold, and threefold rotation axes, respectively. Since they are related to regular geometrical figures, these rotation axes are called high-symmetry elements. Regarding the twofold axis, it fits the symmetry of the parallelogram, which also can be used for filling the 2D space without voids but does not represent a regular geometrical figure. For this reason, the twofold rotation axis is classified as a low symmetry element (together with inversion center, 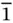 , and mirror plane, m ). It also comes out from Eq. (1.5), that regular figures with fivefold rotation axis ( n = 5), as well as with rotation axes higher than n > 6, are incompatible with translational symmetry, i.e. cannot be used for producing periodic patterns without voids since parameter, p , is not an integer number.
, and mirror plane, m ). It also comes out from Eq. (1.5), that regular figures with fivefold rotation axis ( n = 5), as well as with rotation axes higher than n > 6, are incompatible with translational symmetry, i.e. cannot be used for producing periodic patterns without voids since parameter, p , is not an integer number.
Читать дальше


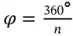 , after rotation by which the lattice remains indistinguishable with respect to its initial setting (lattice invariance). In regular crystals, the permitted rotation axes, i.e. those matching translational symmetry (see Appendix 1.A), are twofold (180°-rotation, n = 2), threefold (120°-rotation, n = 3), fourfold (90°-rotation, n = 4), and sixfold (60°-rotation, n = 6). Of course, onefold, i.e. 360°-rotation ( n = 1), is a trivial symmetry element existing in every Bravaislattice. The international notations for these symmetry elements are:
, after rotation by which the lattice remains indistinguishable with respect to its initial setting (lattice invariance). In regular crystals, the permitted rotation axes, i.e. those matching translational symmetry (see Appendix 1.A), are twofold (180°-rotation, n = 2), threefold (120°-rotation, n = 3), fourfold (90°-rotation, n = 4), and sixfold (60°-rotation, n = 6). Of course, onefold, i.e. 360°-rotation ( n = 1), is a trivial symmetry element existing in every Bravaislattice. The international notations for these symmetry elements are: 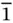 – for inversion center, m – for mirror plane, and 1 , 2 , 3 , 4 , 6 – for respective rotation axes. We see that fivefold rotation axis and axes of the order, higher than n = 6, are incompatible with translational symmetry (see Appendix 1.A).
– for inversion center, m – for mirror plane, and 1 , 2 , 3 , 4 , 6 – for respective rotation axes. We see that fivefold rotation axis and axes of the order, higher than n = 6, are incompatible with translational symmetry (see Appendix 1.A). ( Figure 1.4). Correspondingly, the angle, δ , between adjacent edges is:
( Figure 1.4). Correspondingly, the angle, δ , between adjacent edges is: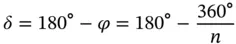
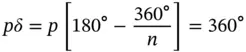
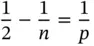


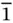 , and mirror plane, m ). It also comes out from Eq. (1.5), that regular figures with fivefold rotation axis ( n = 5), as well as with rotation axes higher than n > 6, are incompatible with translational symmetry, i.e. cannot be used for producing periodic patterns without voids since parameter, p , is not an integer number.
, and mirror plane, m ). It also comes out from Eq. (1.5), that regular figures with fivefold rotation axis ( n = 5), as well as with rotation axes higher than n > 6, are incompatible with translational symmetry, i.e. cannot be used for producing periodic patterns without voids since parameter, p , is not an integer number.










