Saeid Sanei - EEG Signal Processing and Machine Learning
Здесь есть возможность читать онлайн «Saeid Sanei - EEG Signal Processing and Machine Learning» — ознакомительный отрывок электронной книги совершенно бесплатно, а после прочтения отрывка купить полную версию. В некоторых случаях можно слушать аудио, скачать через торрент в формате fb2 и присутствует краткое содержание. Жанр: unrecognised, на английском языке. Описание произведения, (предисловие) а так же отзывы посетителей доступны на портале библиотеки ЛибКат.
- Название:EEG Signal Processing and Machine Learning
- Автор:
- Жанр:
- Год:неизвестен
- ISBN:нет данных
- Рейтинг книги:3 / 5. Голосов: 1
-
Избранное:Добавить в избранное
- Отзывы:
-
Ваша оценка:
- 60
- 1
- 2
- 3
- 4
- 5
EEG Signal Processing and Machine Learning: краткое содержание, описание и аннотация
Предлагаем к чтению аннотацию, описание, краткое содержание или предисловие (зависит от того, что написал сам автор книги «EEG Signal Processing and Machine Learning»). Если вы не нашли необходимую информацию о книге — напишите в комментариях, мы постараемся отыскать её.
EEG Signal Processing and Machine Learning — читать онлайн ознакомительный отрывок
Ниже представлен текст книги, разбитый по страницам. Система сохранения места последней прочитанной страницы, позволяет с удобством читать онлайн бесплатно книгу «EEG Signal Processing and Machine Learning», без необходимости каждый раз заново искать на чём Вы остановились. Поставьте закладку, и сможете в любой момент перейти на страницу, на которой закончили чтение.
Интервал:
Закладка:
In the following sections most of the tools and algorithms for the above objectives are explained and the mathematical foundations discussed. The application of these algorithms to analysis of the normal and abnormal EEGs however will follow in the later chapters of this book. The reader should also be aware of the required concepts and definitions borrowed from linear algebra. Further details of which can be found in [9]. Throughout this chapter and the reminder of this book continuous time is denoted by ‘ t ’ and discrete time, with normalized sampling period T = 1, with ‘ n ’. In some illustrations however the actual timings in seconds are presented.
4.2 Nonlinearity of the Medium
The head as a mixing medium combines EEG signals which are locally generated within the brain at the sensor positions. As a system, the head may be more or less susceptible to such sources in different situations. Generally, an EEG signal can be considered as the output of a nonlinear system, which may be characterized deterministically. This concept represents the fundamental difference between EEG and magnetoencephalography (MEG) signals. Unlike in EEG, for MEG the resistivity (or conductivity) of the head has significantly less effect on the magnetic field although the slight nonlinearity in MEG stems from minor differences in head tissue permittivity values.
Electromagnetic source characterization and localization requires accurate volume conductor models representing head geometry and the electrical conductivity field. It has been shown that, despite extensive research, the measurements of head tissue parameters are inconsistent and vary in different experiments [10]. There is more discussion on this concept in Chapter 10of this book where we introduce brain source localization.
The changes in brain metabolism as a result of biological and physiological phenomena in the human body can change the process of combining the EEG source signals. Some of these changes are influenced by the activity of the brain itself. These effects make the system nonlinear. Analysis of such a system is very complicated and up to now nobody has fully modelled the system to aid in the analysis of brain signals.
Conversely, some measures borrowed from chaos theory and analysis of the dynamics of time series such as dissimilarity, attractor dimension, and largest Lyapunov exponents (LLE) can characterize the nonlinear behaviour of EEG signals. These concepts are discussed in Section 4.7and some of their applications given in Chapter 11.
4.3 Nonstationarity
Nonstationarity of the signals can be quantified by evaluating the changes in signal distribution over time. In a strict‐sense stationary process the signal distribution remains the same for different time intervals. However, often wide‐sense stationarity is not required and therefore, it is sufficient to have various statistics such as mean and variance fixed (or without significant change) over time.
Although in many applications the multichannel EEG distribution is considered as multivariate Gaussian, the mean and covariance properties generally change from segment to segment. As such EEGs are considered stationary only within short intervals and are generally quasi‐stationary. Although this interval can change due to the rapid changes in the brain state such as going from closed eye to open eye, sleep to wakefulness, normal to seizure, change in alertness, brain responding to a stimulation in the form of event‐related potential (ERP) and evoked potential (EP) signals, eye blinking, and emotion change, in practise, a 10 seconds window of EEG is considered stationary.
The change in the distribution of the signal segments can be measured in terms of both the parameters of a Gaussian process and the deviation of the distribution from Gaussian. The non‐Gaussianity of the signals can be checked by measuring or estimating some higher‐order moments such as skewness, kurtosis, negentropy, and Kullback–Leibler (KL) distance (aka KL divergence).
Skewness is a measure of asymmetry, or more precisely, the lack of symmetry in distribution. A distribution is symmetric if it looks the same to the left and right of its midline or mean point. The skewness is defined for a real signal as
(4.1) 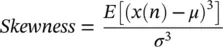
where μ and σ are respectively, the mean and variance and E denotes statistical expectation. If the distribution is more to the right of the mean point the skewness is negative, and vice versa. For a symmetric distribution such as Gaussian, the skewness is zero.
Kurtosis is a measure for showing how peaked or flat a distribution is relative to a normal distribution. That is, datasets with high kurtosis tend to have a distinct peak near the mean, decline rather rapidly, and have heavy tails. Datasets with low kurtosis tend to have a flat top near the mean rather than a sharp peak. A uniform distribution would be the extreme case. The kurtosis for a signal x ( n ) is defined as:
(4.2) 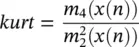
where m i( x ( n )) is the i th central moment of the signal x(n) , i.e. m i(x(n)) = E [(x(n) − μ ) i]. The kurtosis for signals with normal distributions is three. Therefore, an excess or normalized kurtosis is often used and defined as
(4.3) 
which is zero for Gaussian distributed signals. Often the signals are considered ergodic, hence the statistical averages can be assumed identical to time averages so that they can be estimated with time averages.
The negentropy of a signal x ( n ) [11] is defined as:
(4.4) 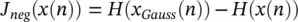
where x Gauss( n ) is a Gaussian random signal with the same covariance as x ( n ) and H(.) is the differential entropy [12] defined as:
(4.5) 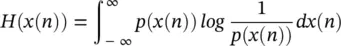
and p (x( n )) is the signal distribution. Negentropy is always nonnegative.
Entropy, by itself, is an important measure of EEG behaviour particularly in the cases in which the brain synchronization changes such as when brain waves become gradually more synchronized when the brain approaches the seizure onset. It is also a valuable indicator of other neurological disorders presented in psychiatric diseases.
By replacing the probability density function (pdf) with joint or conditional pdfs in Eq. (4.5), joint or conditional entropy is defined respectively. In addition, there are new definitions of entropy catering for neurological applications such as the multiscale fluctuation‐based dispersion entropy defined in [13], which is briefly explained in this chapter, and those in the references herein.
The KL distance between two distributions p 1and p 2is defined as:
(4.6) 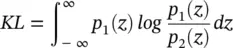
Интервал:
Закладка:
Похожие книги на «EEG Signal Processing and Machine Learning»
Представляем Вашему вниманию похожие книги на «EEG Signal Processing and Machine Learning» списком для выбора. Мы отобрали схожую по названию и смыслу литературу в надежде предоставить читателям больше вариантов отыскать новые, интересные, ещё непрочитанные произведения.
Обсуждение, отзывы о книге «EEG Signal Processing and Machine Learning» и просто собственные мнения читателей. Оставьте ваши комментарии, напишите, что Вы думаете о произведении, его смысле или главных героях. Укажите что конкретно понравилось, а что нет, и почему Вы так считаете.












