Computational Modeling and Simulation Examples in Bioengineering

The shape of AAA is very important. The severity of AAA is commonly estimated in clinical practice by considering the AAA maximal diameter. However, from the mechanical point of view, the hemodynamic effects and the mechanical stresses within the AAA tissue certainly are important in the process of the AAA rupture. Bulge diameter alone may not be a sufficient criterion for determination of rupture risk; therefore, an insight into the hemodynamic effects and the stress–strain quantification and distribution within the vessel wall are of great significance even in medical practice.

A simplified geometry of an aneurism is shown in Figure 1.5. With the on‐web software, the 3D finite element model for the blood flow domain can be parametrically generated. A transition smoothness between the surfaces is achieved by using Bezier's curves. Also, the results can be displayed with a user‐friendly menu in a way suitable for an insight into medical aspects of the blood flow conditions. A detailed description of the software use is given on the web (within Tutorial of each example), while the description of geometric parameters is given in the caption of Figure 1.5.

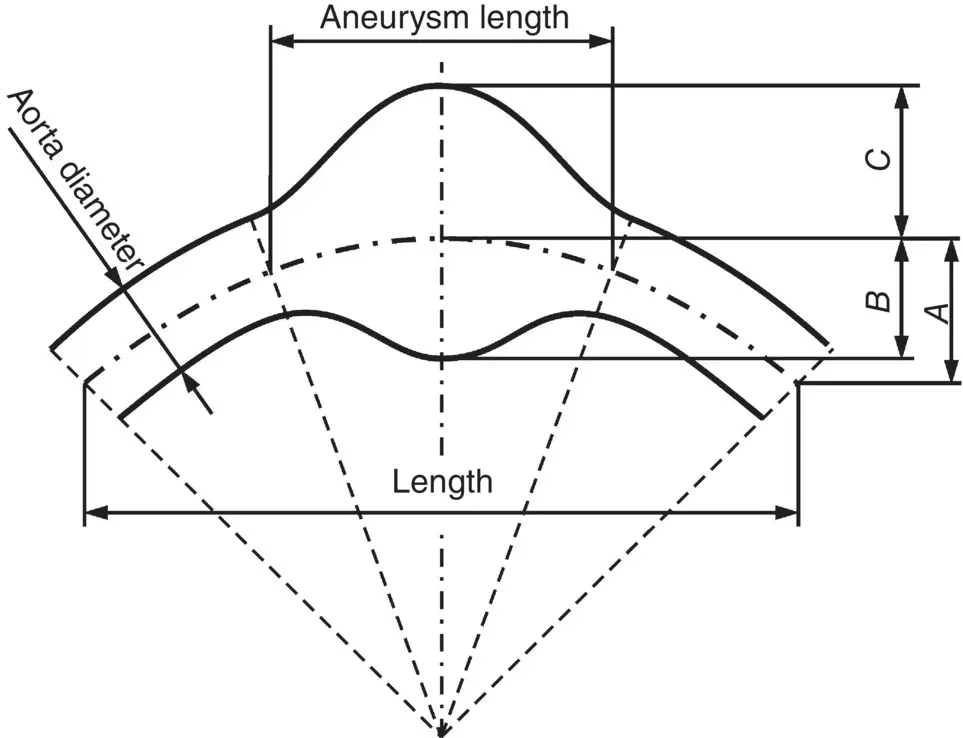
Figure 1.5Geometrical parameters of AAA: “Length” is the parameter which defines the total horizontal projection of the generated aneurysm model; “ A ” is the height of the arc of central line; “Aorta diameter” is the abdominal aorta diameter; “ B ” is the radius from the central line to the inner wall of the aneurysm; “ C ” is the radius from the central line to the outer wall of the aneurysm; “Aneurysm length” is an average length of the AAA.

At the inflow aorta cross‐section, a fully developed parabolic flow is assumed, determined by a selected volume flux. The normal stress and tangential stress are set to be equal to zero (stress‐free condition) or they are prescribed at the outlet cross‐section.

It is assumed that the entering flow is pulsatile, with a typical waveform shown in Figure 1.6[127]. As described in the software menu, the waveform can be changed.

Figure 1.6A typical in‐flow waveform at the aorta entry. Q is the volumetric in‐flux and t / T is the relative time with respect to the cycle period T .

Results for two examples of the symmetric AAA are given here: (i) case with rigid walls and (ii) AAA with deformable walls. Results not shown here and solutions for other model parameters can be obtained using Software on the web.

We analyze an aneurism at the straight aorta domain, where aorta proximal and distal to the AAA bulge is idealized as straight rigid tube and branching arteries are excluded. The model has ratio D / d = 2.75 and geometry generated according to Figure 1.5( D and d are diameters of the bulge and aorta, respectively). The data are: blood density is ρ = 1.05 g/cm 3; kinematic viscosity (Newtonian fluid) ν = 0.035 cm 2/s, d = 12.7 mm. The inflow velocity is defined by the flux function given in Figure 1.6. The FE mesh consisted of approximately 8000 3D 8‐node brick elements.

The results for the velocity and pressure at peak systole t / T = 0.16 are shown in Figure 1.7. The velocity disturbance in the region of the aneurism is notable. Also, the region of maximum pressure is located inside AAA.

Figure 1.7Velocity field (left panel) and pressure distribution (right panel) for peak systole t / T = 0.16 of AAA for the model with D / d = 2/75, d = 12.7 mm.

Here, an aneurysm of the straight aorta with deformable walls is modeled according to the FSI algorithm. Blood flow is calculated using 2112 eight‐node 3D elements, and 264 four‐node shell elements used to model the aorta wall, with the wall thickness δ = 0.2 cm. The material constants for blood as in the previous example, while data for the vessel wall are: Young's modulus E = 2.7 MPa, Poisson's ratio v = 0.45, wall thickness δ = 0.2 cm, and tissue density ρ = 1.1 g/cm 3. Boundary conditions for the model are prescribed velocity profile (see Figure 1.8a) and output pressure profile as given in Figure 1.8b.

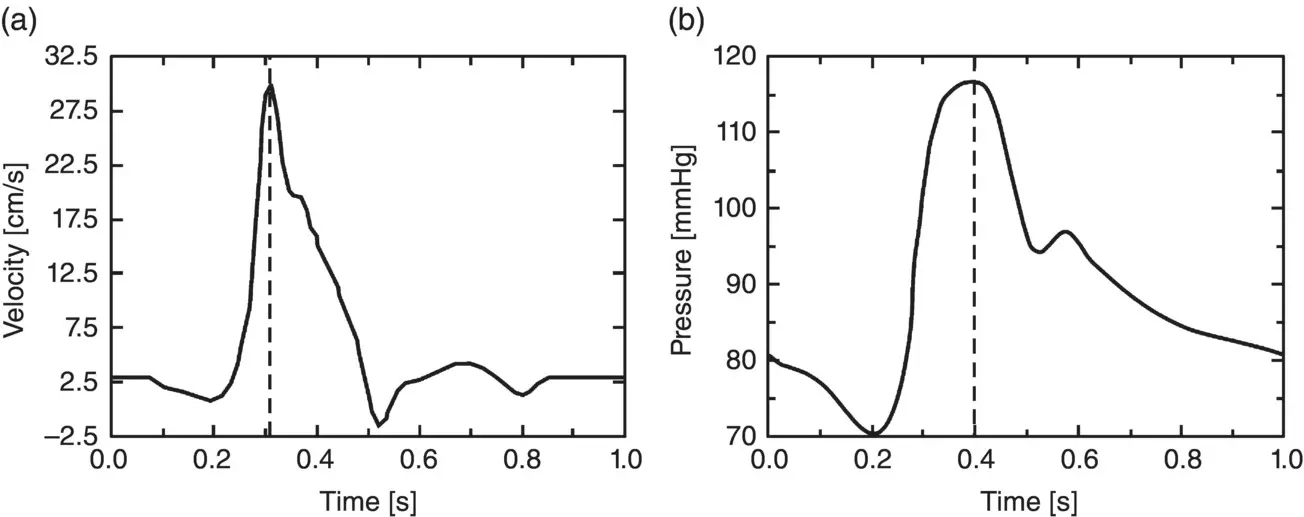
Figure 1.8Input velocity and output pressure profiles for the AAA on a straight vessel. Inlet peak systolic flow is at t = 0.305 s and outlet peak systolic pressure is at t = 0.4 s. (a) Velocity waveform; (b) pressure waveform.

Source : Modified from Scotti et al. [44].

The results for velocity magnitude distribution at t = 0.305 s are shown in Figure 1.9a. The von Mises wall stress distributions at t = 0.4 s is given in Figure 1.9b. It can be seen that the velocities are low in the domain of the aneurism, while the larger values of the wall stress are at the proximal and distal aneurism zones.

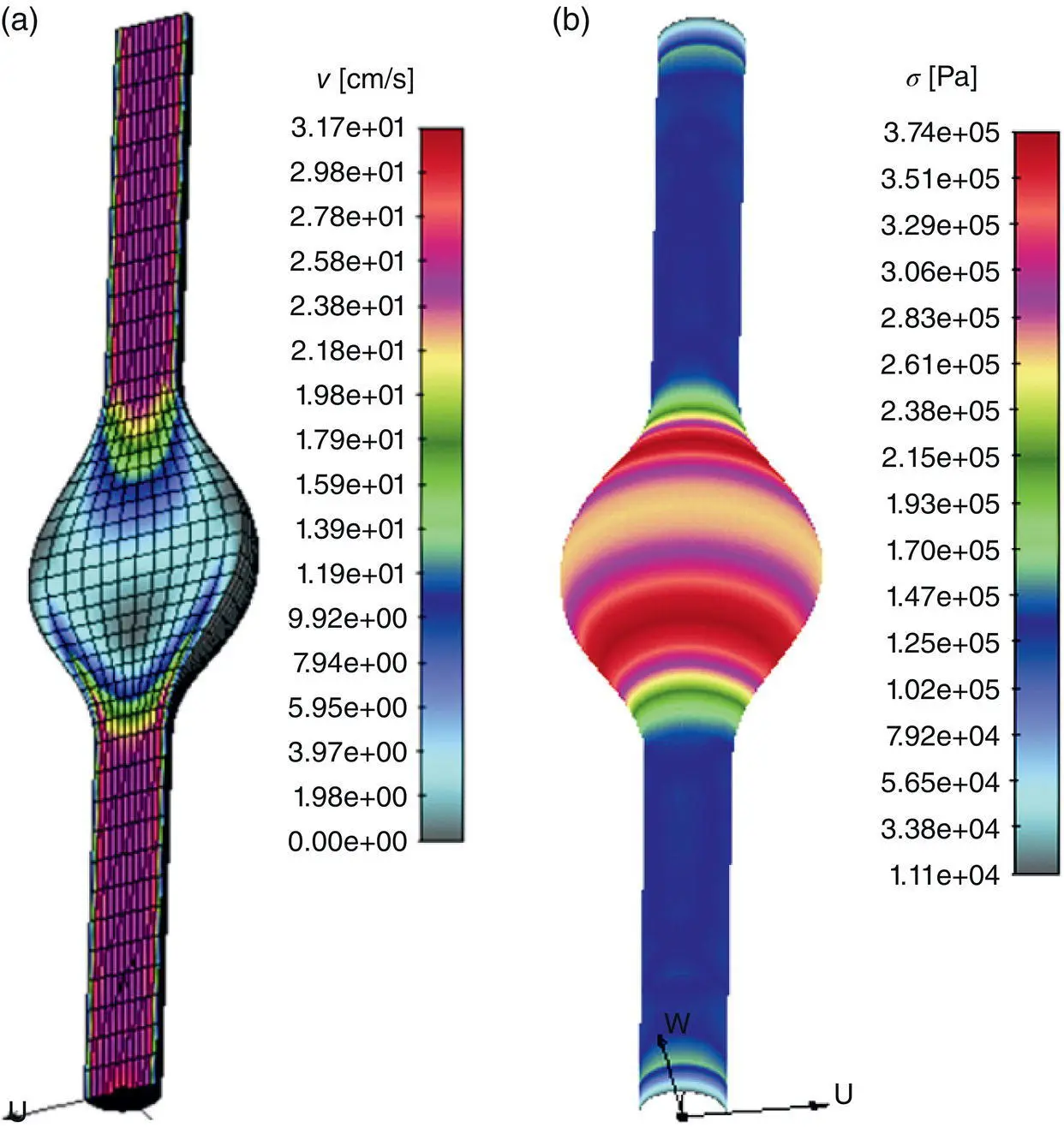
Figure 1.9Velocity magnitude field and von Mises wall stress distribution for symmetric AAA on the straight vessel. (a) Velocity field distribution for peak at t = 0.305 s; (b) von Mises wall stress distributions for blood pressure peak at t = 0.4 s.

1 1 McGloughlin, T.M. and Doyle, B.J. (2010). New approaches to abdominal aortic aneurysm rupture risk assessment: engineering insights with clinical gain. Arterioscler. Thromb. Vasc. Biol. 30: 1687–1694. https://doi.org/10.1161/ATVBAHA.110.204529.

2 2 Fleming, C., Whitlock, E.P., Beil, T., and Lederle, F.A. (2005). Review: screening for abdominal aortic aneurysm: a best‐evidence systematic review for the U.S. Preventive Services Task Force. Ann. Intern. Med. 142: 203–211.

3 3 O'Gara, T.P. (2003). Aortic aneurysm. Circulation 107: e43–e45.

4 4 Minino, A.M., Heron, M.P., Murphy, S.L., and Kochanek, K.D. (2007). Deaths: final data for 2004. Nat. Vital. Stat. Rep. 55: 1–119.

5 5 Sakalihasan, N., Limet, R., and Defawe, O.D. (2005). Abdominal aortic aneurysm. Lancet 365 (9470): 1577–1589.

6 6 Thompson, M.M. (2003). Controlling the expansion of abdominal aortic aneurysms. Br. J. Surg. 90: 897–898.

7 7 Thompson, M.M. (2003). Infrarenal abdominal aortic aneurysms. Curr. Treat Opt. Cardiovasc. Med. 5 (2): 137–146.

8 8 Thompson, R.W. and Baxter, B.T. (1999). MMP inhibition in abdominal aortic aneurysms. Rationale for a prospective randomized clinical trial. Ann. N. Y. Acad. Sci. 878: 159–178.

9 9 Bown, M.J., Sutton, A.J., Bell, P.R., and Sayers, R.D. (2002). A meta‐analysis of 50 years of ruptured abdominal aortic aneurysm repair. Br. J. Surg. 89: 714–730.