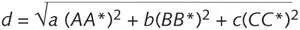Julian Barbour - The End of Time - The Next Revolution in Physics

The idea that Bruno and I first developed seemed so natural it surprised us that no one had thought of it earlier. However, I learned quite recently that something similar was proposed in 1904 (in an obscure booklet by one Wenzel Hofmann), and then rediscovered in 1914 by the physicist Hans Reissner and again in 1925 by none other than Schrödinger, just before he discovered wave mechanics. This was especially ironic since Bruno had been Schrödinger’s student. I think the main reason why these papers got overlooked was that they were completely overshadowed by Einstein’s general relativity and the excitement of the discovery of quantum mechanics in 1925/6. There is also an undoubted tendency for physicists to work within a so-called paradigm (the American philosopher of science Thomas Kuhn’s famous expression), and pay at best fleeting attention to ideas that do not fit within the existing established patterns of thought.

I mention these things because the next idea that Bruno and I tried seems to me just as natural as our first idea, if one approaches the problems of describing motion and change with an open mind. It does, however, seem very different from the present paradigm, which has become deep-rooted with the long hegemony of Newtonian ideas, which were only partly changed by Einstein. Although, as we shall see, our second idea is actually built into Einstein’s theory at its very heart, within the context of classical physics it merely provides a different perspective on that theory. However, for the study of quantum effects it does represent a genuine alternative, and the attempt to create a quantum theory of the universe may force its adoption, alien though it may appear to many working scientists.

Let me now explain this second idea. So far, I have explained only what the points of Platonia are. Each is a possible relative arrangement, a configuration, of all the matter in the universe. If there are only three bodies in it, Platonia is Triangle Land, each point of which is a triangle (Figures 3 and 4). Can we somehow say ‘how far apart’ any two similar but distinct triangles are? If so, this will define a ‘distance’ between neighbouring points in Triangle Land, and just as mathematicians seek geodesics using ordinary distances on curved surfaces, we can start to look for geodesics in Platonia. If we can find them, they will be natural candidates for actual histories of the universe, which we have identified as paths in Platonia. They will be Machian histories if the ‘distance’ between any two neighbouring points in Platonia is determined by their structures and nothing else, and we shall not need to suppose that they are embedded in some extra structure like absolute space.

There is such a simple and natural solution to the problem of finding geodesics in Platonia that I would like to spell it out. The fact that it does seem to be used by nature is one of the two prime pieces of evidence I have for suggesting that the universe is timeless. (The second, equally simple in its way, comes from quantum mechanics.) How it works out for the simplest example of a universe of three bodies is described in Box 8.

BOX 8 Intrinsic Difference and Best Matching

In Figure 21, triangle ABC is one point in Triangle Land, and the slightly different triangle A*B*C* is a neighbouring point. A ‘distance’ between them can be found in many ways, but one of the simplest is the following. Imagine that ABC is held fixed, and A*B*C* is placed in any position relative to it. This creates ‘distances’ AA*, BB*, CC* between the corresponding vertices, at which we suppose there are bodies of masses a, b, c . We form a ‘trial distance’ d by taking each mass and multiplying it by the square of the corresponding distance, adding the results and taking the square root of the sum. Thus

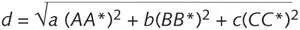
This is an arbitrary quantity, since the relative positioning of the two triangles is arbitrary. It is, however, possible to consider all relative positionings and find the one for which d is minimized. This is a very natural quantity to find, and it is not arbitrary. Two different people setting out to find it for the same two triangles would always get the same result. It measures the intrinsic difference between the two matter distributions represented by the triangles. It is completely determined by them, and does not rely on any external structure like absolute space.

The intrinsic difference between two arbitrary matter distributions can be found similarly. One distribution is supposed fixed, and the other moved relative to it. In any trial position, the analogue of the above expression is calculated, and the position in which it is minimized is sought. Because this special position reduces the apparent difference between the two matter configurations to a minimum, it may be called the best-matching position .

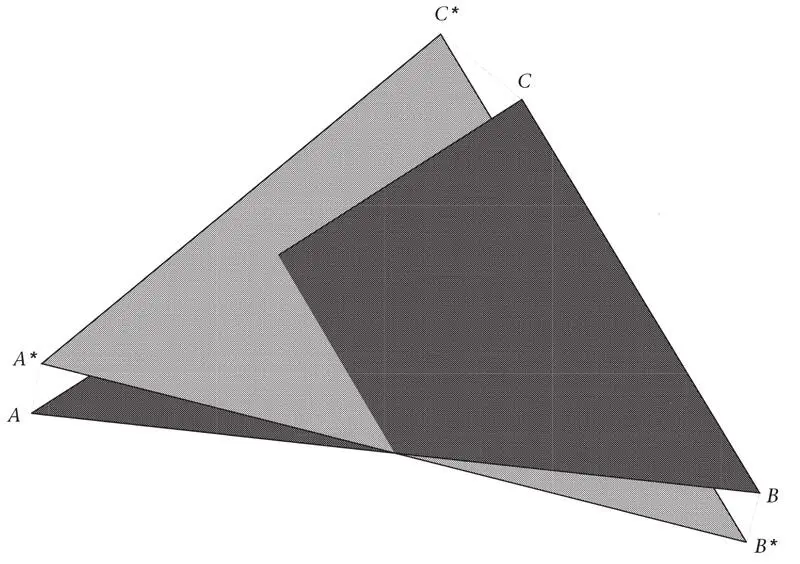
Figure 21.A trial relative placing of the two triangles.

Using the intrinsic difference defined in Box 8, we can determine ‘shortest paths’ or ‘histories’ in Platonia as explained above. However, the intrinsic difference by itself does not lead to very interesting histories, and it is more illuminating to consider a related quantity. The potential energy of any matter distribution (Figure 17) is determined by its relative configuration, and is therefore already ‘Machian’. Each matter distribution has its own Newtonian gravitational potential energy. Two nearly identical matter distributions have almost the same potential. Now, the intrinsic difference is determined by two nearly identical configurations. To obtain more interesting histories we can simply multiply the intrinsic differences by the potential (strictly speaking, by the square root of minus the potential). This will change the definition of ‘distance’, but it will still enable us to determine ‘shortest distances’. You do not need to worry about these details, but I do want to give you a flavour of what is involved.

I think you will agree that finding shortest paths in an imagined timeless landscape bears little direct resemblance to our powerful sense of the passage of time. Yet the outcome turned out to be remarkably like what happens in Newtonian theory. Let me explain, taking again the example of a three-body universe, for which Platonia is Triangle Land.

Any continuous path in it corresponds to a sequence of triangles: they are the ‘points’ through which the path passes. But this is very similar to what comes out of Newtonian theory (Figure 1) – which, however, yields not only the triangles but also their positions in absolute space and separations in time. Remember that the triangles in Figure 1 are ‘lit up’ by flashes at each unit of absolute time, and that we see them, in perspective, in absolute space at those times. However, these are invisible aids. The astronomers see neither when they look through telescopes, all they see are stars. Thus, as far as observable things are concerned, both theories yield the same kind of thing – sequences of triangles. The question is, what kind of sequences do the two theories yield? In what respects do the theories differ when it comes down to what is actually observable?

The major difference is that the Machian theory makes more definite predictions. As a theory of geodesics, it determines the shortest path between any two fixed points in Platonia. It covers Platonia with such paths. These geodesics have the following important property: at any one point in Platonia, many of them pass through it. In fact, for every direction that you can go from a point, there is just one geodesic. This is the crucial difference. As Figures 13 and 14 highlighted, when the Newtonian histories are represented as paths in Platonia, it turns out that many can pass through the same point, and have at that point the same direction. However, these paths then ‘splay out’ and go to quite different places in Platonia. In Newtonian terms, they differ in energy and angular momentum. The difference is not apparent in the initial point and direction, but comes to light dramatically in the later evolution of the paths. This defect is absent in the Machian theory. For any given point and direction at that point, there is just one geodesic. Bruno and I constructed the theory precisely to achieve that aim.