Astronomers estimate that the nearest black hole to Earth is roughly 300 light-years away: a hundred times farther than the nearest star (other than the Sun), Proxima Centauri.
Now armed with a basic understanding of the universe, fields, warped time and space and especially black holes, we are ready, at last, to explore Interstellar ’s Gargantua.
If we know the mass of a black hole and how fast it spins, then from Einstein’s relativistic laws we can deduce all the hole’s other properties: its size, the strength of its gravitational pull, how much its event horizon is stretched outward near the equator by centrifugal forces, the details of the gravitational lensing of objects behind it. Everything.
This is amazing. So different from everyday experience. It is as though knowing my weight and how fast I can run, you could deduce everything about me: the color of my eyes, the length of my nose, my IQ, …
John Wheeler (my mentor, who gave “black holes” their name) has described this by the phrase “A black hole has no hair”—no extra, independent properties beyond its mass and its spin. Actually, he should have said, “A black hole has only two hairs, from which you can deduce everything else about it,” but that’s not as catchy as “no hair,” which quickly became embedded in black-hole lore and scientists’ lexicon. [13] The literal French translation of “a black hole has no hair” is so obscene that French publishers resisted it vigorously, to no avail.
From the properties of Miller’s planet, as depicted in Interstellar , a physicist who knows Einstein’s relativistic laws can deduce Gargantua’s mass and spin, and thence all else about it. Let’s see how this works. [14] For some quantitative details, see Some Technical Notes , at the end of this book.
Gargantua’s Mass

Miller’s planet (which I talk about at length in Chapter 17) is about as close to Gargantua as it can possibly be and still survive. We know this because the crew’s extreme loss of time can only occur very near Gargantua.
At so close a distance, Gargantua’s tidal gravitational forces (Chapter 4) are especially strong. They stretch Miller’s planet toward and away from Gargantua and squeeze the planet’s sides (Figure 6.1).
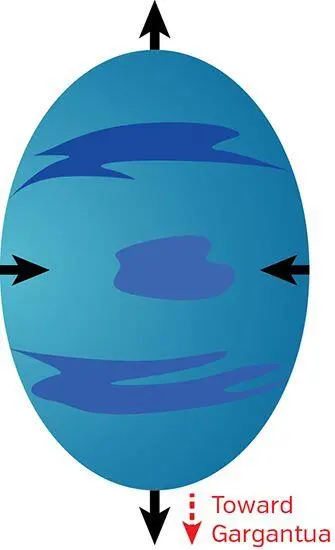
Fig. 6.1. Gargantua’s tidal gravitational forces stretch and squeeze Miller’s planet.
The strength of this stretch and squeeze is inversely proportional to the square of Gargantua’s mass. Why? The greater Gargantua’s mass, the greater its circumference, and therefore the more similar Gargantua’s gravitational forces are on the various parts of the planet, which results in weaker tidal forces. (See Newton’s viewpoint on tidal forces; Figure 4.8.) Working through the details, I conclude that Gargantua’s mass must be at least 100 million times bigger than the Sun’s mass. If Gargantua were less massive than that, it would tear Miller’s planet apart!
In all my science interpretations of what happens in Interstellar , I assume that this actually is Gargantua’s mass: 100 million Suns. [15] A more reasonable value might be 200 million times the Sun’s mass, but I want to keep the numbers simple and there’s a lot of slop in this one, so I chose 100 million.
For example, I assume this mass in Chapter 17, when explaining how Gargantua’s tidal forces could produce the giant water waves that inundate the Ranger on Miller’s planet.
The circumference of a black hole’s event horizon is proportional to the hole’s mass. For Gargantua’s 100 million solar masses, the horizon circumference works out to be approximately the same as the Earth’s orbit around the Sun: about 1 billion kilometers . That’s big! After consulting with me, that’s the circumference assumed by Paul Franklin’s visual-effects team, when producing the images in Interstellar .
Physicists attribute to a black hole a radius equal to its horizon’s circumference divided by 2π (about 6.28). Because of the extreme warping of space inside the black hole, this is not the hole’s true radius. Not the true distance from the horizon to the hole’s center, as measured in our universe. But it is the event horizon’s radius (half its diameter) as measured in the bulk; see Figure 6.3 below. Gargantua’s radius, in this sense, is about 150 million kilometers, the same as the radius of the Earth’s orbit around the Sun.
Gargantua’s Spin

When Christopher Nolan told me how much slowing of time he wanted on Miller’s planet, one hour there is seven years back on Earth , I was shocked. I didn’t think that possible and I told Chris so. “It’s non-negotiable,” Chris insisted. So, not for the first time and also not the last, I went home, thought about it, did some calculations with Einstein’s relativistic equations, and found a way.
I discovered that, if Miller’s planet is about as near Gargantua as it can get without falling in, [16] See Figure 17.2 and the discussion of it in Chapter 17.
and if Gargantua is spinning fast enough, then Chris’s one-hour-in-seven-years time slowing is possible. But Gargantua has to spin awfully fast.
There is a maximum spin rate that any black hole can have. If it spins faster than that maximum, its horizon disappears, leaving the singularity inside it wide open for all the universe to see; that is, making it naked —which is probably forbidden by the laws of physics (Chapter 26).
I found that Chris’s huge slowing of time requires Gargantua to spin almost as fast as the maximum: less than the maximum by about one part in 100 trillion . [17] In other words, its spin is the maximum minus 0.00000000000001 of the maximum.
In most of my science interpretations of Interstellar , I assume this spin.
The crew of the Endurance could measure the spin rate directly by watching from far, far away as the robot TARS falls into Gargantua (Figure 6.2). [18] When TARS falls in, the Endurance is not far, far away, but rather is on the critical orbit, quite near the horizon, whirling around the hole nearly as fast as TARS; so Amelia Brand, in the Endurance , does not see TARS swept around at high speed. For more on this, see Chapter 27.
As seen from afar, TARS never crosses the horizon (because signals he sends after crossing can’t get out of the black hole). Instead, TARS’ infall appears to slow down, and he appears to hover just above the horizon. And as he hovers, Gargantua’s whirling space sweeps him around and around Gargantua, as seen from afar. With Garantua’s spin very near the maximum possible, TARS’ orbital period is about one hour, as seen from afar.
You can do the math yourself: the orbital distance around Gargantua is a billion kilometers and TARS covers that distance in one hour, so his speed as measured from afar is about a billion kilometers per hour, which is approximately the speed of light! If Gargantua were spinning faster than the maximum, TARS would whip around faster than the speed of light, which violates Einsteinʼs speed limit. This is a heuristic way to understand why there is a maximum possible spin for any black hole.
Читать дальше















