Pete Bonanni - The Art of the Kill

Turn rate [16] Turn rate = K G/V K is a constant and big G and V are the same as in the equation for turn radius. is the second important factor for turning the jet. Turn rate indicates how fast the aircraft moves around the turn radius or circle we just talked about. It is also described as how fast an aircraft can change its nose position. Turn rate is measured in degrees per second and is also dependent on Gs and airspeed.

The higher the G in the above equation, the faster the turn rate. Velocity still remains an important factor. Notice that G is divided by velocity. If G remains at maximum, a higher velocity will cause turn rate to decrease. The reverse is true: a lower velocity will yield a higher turn rate.

You may think that slowing down to minimum airspeed and pulling as hard as you can is the best course of action in order to achieve a high turn rate. Not so fast. There is a relationship between airspeed and Gs. At lower airspeeds, you have less G available or, in other words, you can’t pull as many Gs as you get slow. Less lift is produced by the wings of an aircraft at slower speeds, and as a result, there is less force available to turn the aircraft. If you get going really fast (above Mach 1, for example), you also lose G availability. For every fighter, there is an optimum airspeed for achieving the highest turn rate. The airspeed where the jet has the quickest turn rate with the smallest turn radius is called corner velocity. In most modern fighters, it is between 400 to 500 KCAS. [17] KCAS — knots, computed airspeed The F-16 has a corner velocity of about 450 KCAS.

Figure 2-3 shows the relationship between airspeed (labeled as a Mach number), turn rate and turn radius. The top of the figure shows turn rate and turn radius broken out individually, while the bottom of the graph shows them combined. These graphs in Figure 2-3 are generic turn rate and radius charts. The bottom chart represents the approximate turn performance of an F-16.

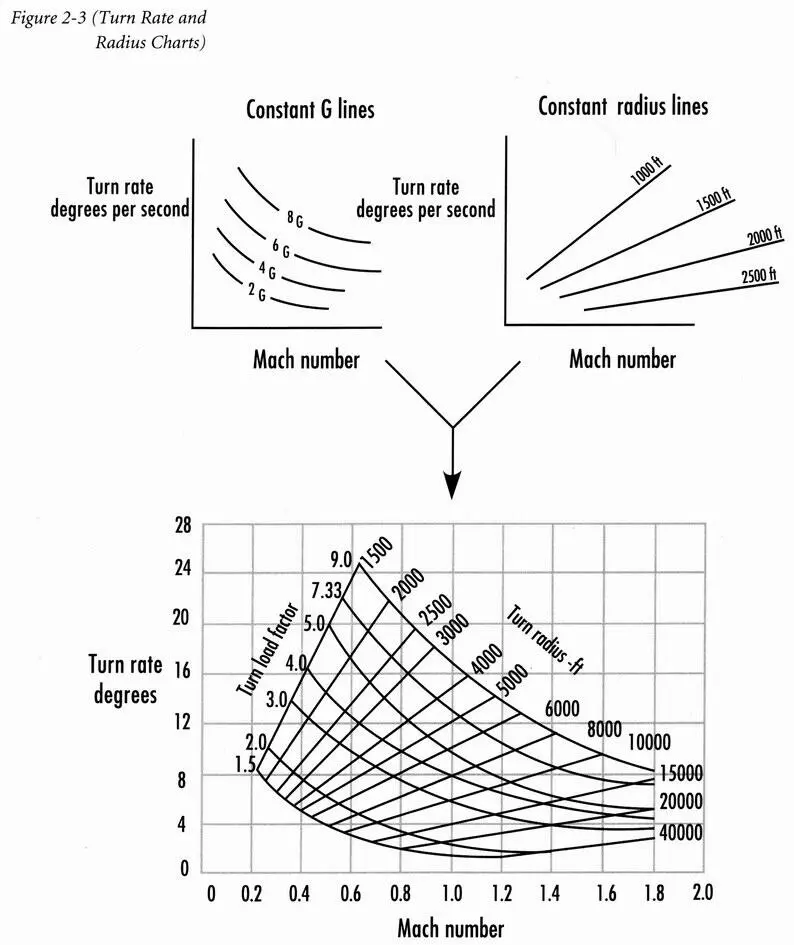
Note that at 0.6 Mach, the jet can pull 9 Gs and turn at a rate of 24° per second. At 0.6 Mach, the jet can also turn in a radius of 1,500 feet. This is the best (tightest) radius the jet can achieve at the highest turn rate possible. The jet can turn this same radius at slower airspeeds, but turn rate will go down significantly. At 0.4 Mach, for example, the jet can turn with a radius of 1,500 feet, but the turn rate falls from 24° to 16° a second. Just to put this figure in perspective, a 2° per second turn rate advantage will allow you to dominate an adversary.

The airspeed of a jet can be controlled by the pilot in the following four ways:

► Throttle position

► Drag devices

► Nose position in relation to the horizon

► Aircraft G

Throttle position controls how much slow, cold air you turn into fast, hot gas. Drag devices refer primarily to speed brakes. Nose position in relation to the horizon also affects airspeed. For example, a nose-low position will increase your airspeed because of the effect of gravity. Finally, G force causes airspeed to bleed off. Remember the brief discussion earlier about exchanging energy for position. No modern fighter flying at medium altitude can stay at corner velocity while pulling max Gs for long. As you pull Gs, you will get slower. It is important, however, to start maneuvering close to corner velocity because the first turn you make is usually the most important in the fight.

Fighter pilots should think in terms of both turn rate and turn radius. A fighter with a superior turn rate can outmaneuver a fighter that has a poor turn rate but a tighter turn radius. Fighter pilots have a simple two-word saying: “Rate kills.” What this means is that the ability to move (or rate) your nose is the primary means of employing weapons (which is what offensive BFM is all about). A bandit may have a tight turn circle, but if you can rate your nose on him and shoot, the fight is over. The flaming wreckage will no longer cause you BFM problems.

I have heard it said (incorrectly) that you fly in relation to the bandit and not the earth. While it is obvious that you must fly in relation to the bandit, you must simultaneously keep your nose in control of the horizon. Gravity affects airspeed, as already mentioned. Gravity also affects G availability. If you pull the nose of a fighter straight across the horizon, gravity will have no effect on your turn performance. When you pull the nose up or down, however, gravity becomes a player.

Figure 2-4 introduces a new term: radial G. To understand how an aircraft turns, you must understand that there are two factors that determine the rate and radius of a fighter’s turn. The first is the G being felt and read out on the G meter in the cockpit. The second is the pull of gravity. Radial G is a term used by fighter pilots to describe the effective G that determines a fighter’s turn. Figure 2-4 shows this concept by depicting a fighter doing a loop. In Figure 2-4, the cockpit G (the G felt by the pilot) is a constant 5 Gs. You will notice that when an aircraft is straight and level and trying to pull in the vertical, the effective G or radial G is only 4. Gravity is subtracted from cockpit G so that the jet is pulling only 4 radial Gs. When the fighter is pulling 5 Gs in the cockpit in the pure vertical (90° point) either straight up or straight down, gravity has no effect, so radial G is equal to cockpit G. When the fighter is inverted and pulling straight down at 5 Gs, gravity adds 1 G to your effective or radial G. The fighter, in effect, is turning at 6 Gs at this point. Radial G then is simply a term that describes the effective or turning G created by combining the positive or negative influence of gravity with cockpit G.

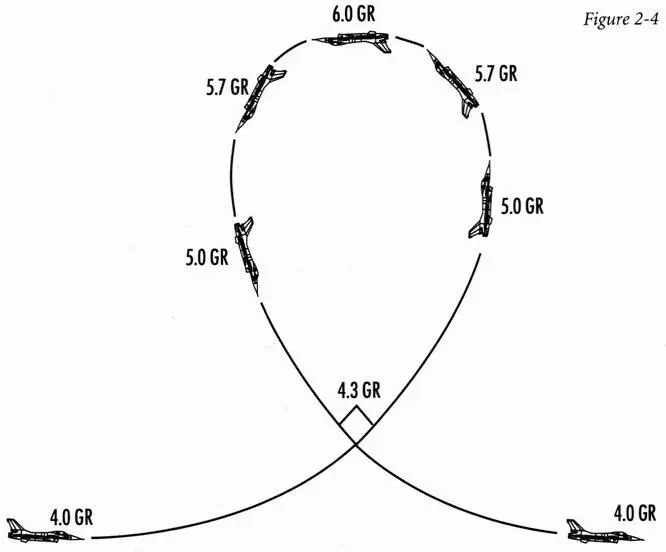
What Figure 2-4 shows is that cockpit G is not equal to radial or turning G when maneuvering in the vertical. Remember that 2° per second is a significant turning advantage. The extra G you can get by placing your nose below the horizon when you turn can give you at least 2° per second turn advantage. Most of the time, 1 GR equates to 3°-4° per second.

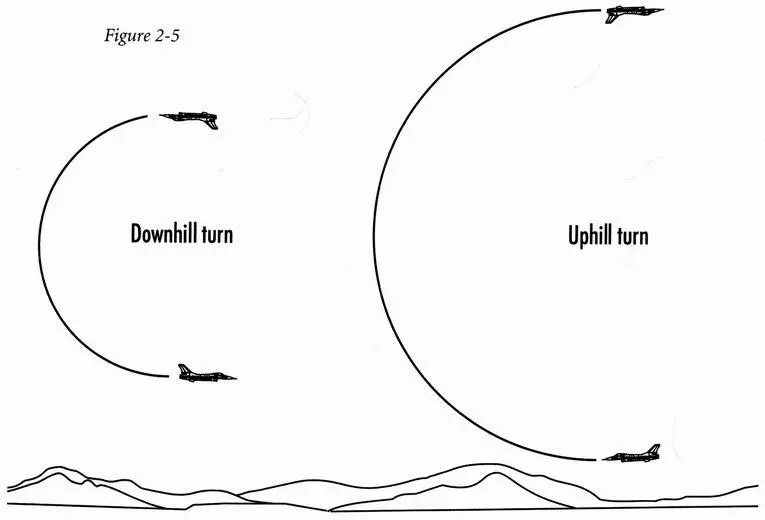
The concept of radial G can be seen in Figure 2-5. In this figure, both fighters are pulling the same cockpit G. Notice that the fighter with his lift vector below the horizon is turning tighter. What is not so obvious in this figure is that the fighter turning toward the ground is also moving or rating the nose faster.

When a bandit turns his jet, he creates BFM problems for you. To solve these problems, you need to turn your jet. In order to turn your jet and solve these BFM problems, you need turning room. Turning room is the offset or distance from the bandit. There are three basic types of turning room: lateral (or horizontal) turning room, vertical turning room, and a combination of both. In order to understand the concept of turning room, you must first understand turn circles. Turn circles are simply the paths that a fighter cuts through the sky when it turns. Figure 2-6 shows a turn circle.

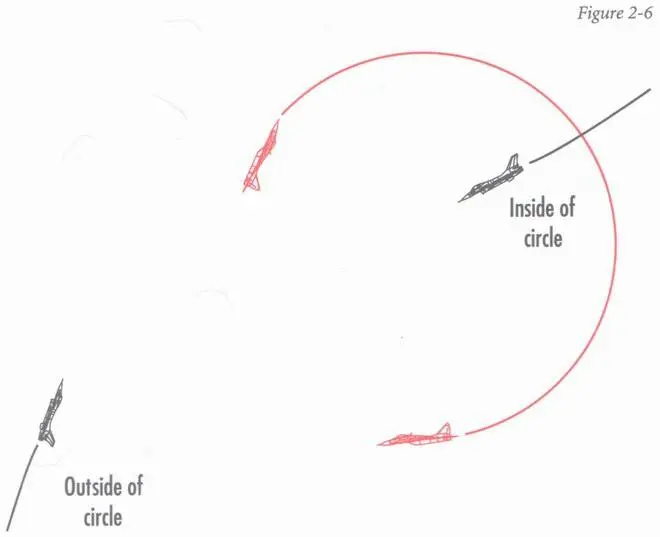
The concept of turn circles is critically important to understand because, in order to turn and solve BFM problems created by the bandit, you must first drive your jet inside the bandit’s turn circle.